A splash in heard 3.12 s after a stone is dropped into a well 45 m deep. The speed of sound in air is [g=10ms−2]
330ms−1
375ms−1
340ms−1
346ms−1
The correct Answer is:B
To solve the problem, we need to analyze the motion of the stone dropped into the well and the time taken for the sound of the splash to travel back up to the top of the well.
1. Understanding the Problem:
- A stone is dropped into a well that is 45 m deep.
- The total time taken from when the stone is dropped to when the splash is heard is 3.12 seconds.
- We need to find the speed of sound in air.
2. Setting Up the Equations:
- Let t1 be the time taken for the stone to fall to the water surface.
- Let t2 be the time taken for the sound to travel back up to the top of the well.
- According to the problem, we have:
t1+t2=3.12 seconds
3. Calculating the Time for the Stone to Fall:
- The stone is dropped, so its initial velocity u=0.
- The distance fallen by the stone is 45 m.
- Using the equation of motion:
s=ut+12gt2
where s=45 m, g=10m/s2, we can substitute:
45=0⋅t1+12⋅10⋅t21
Simplifying this gives:
45=5t21⟹t21=455=9⟹t1=3 seconds
4. Finding the Time for Sound to Travel:
- Now we can find t2:
t2=3.12−t1=3.12−3=0.12 seconds
5. Calculating the Speed of Sound:
- The distance the sound travels is the same depth of the well, which is 45 m.
- The speed of sound v can be calculated using the formula:
v=distancetime=45 mt2
Substituting t2=0.12 seconds:
v=450.12=375 m/s
Final Answer:
The speed of sound in air is 375 m/s.
- A stone is dropped into a well that is 45 m deep.
- The total time taken from when the stone is dropped to when the splash is heard is 3.12 seconds.
- We need to find the speed of sound in air.
- Let t1 be the time taken for the stone to fall to the water surface.
- Let t2 be the time taken for the sound to travel back up to the top of the well.
- According to the problem, we have:
t1+t2=3.12 seconds
- The stone is dropped, so its initial velocity u=0.
- The distance fallen by the stone is 45 m.
- Using the equation of motion:
s=ut+12gt2
where s=45 m, g=10m/s2, we can substitute:
45=0⋅t1+12⋅10⋅t21
Simplifying this gives:
45=5t21⟹t21=455=9⟹t1=3 seconds
- Now we can find t2:
t2=3.12−t1=3.12−3=0.12 seconds
- The distance the sound travels is the same depth of the well, which is 45 m.
- The speed of sound v can be calculated using the formula:
v=distancetime=45 mt2
Substituting t2=0.12 seconds:
v=450.12=375 m/s
The speed of sound in air is 375 m/s.
Similar Questions
A stone is dropped into a well 45m deep. The sound of the splash of the splash is heard 3.13s after the stone is dropped. Find the speed of sound in air. Take g=10m/s2.
View SolutionIf the splash is hear 4.23 seconds after a stone is dropped into a well, 78.4 metres deep, find the velocity of sound in air.
View Solution
Knowledge Check
- Question 1 - Select One
The splash of sound was heard 5.35s after dropping a stone into a well 122.5 m deep. Velocity of sound in air is
A350cm/sB350m/sC392cm/sD0cm/s - Question 2 - Select One
An iron block is dropped into a deep well. Sound of splash is heard after 4.23 s. If the depth of the well is 78. 4 m , then find the speed of sound in air (g=9.8m/s2)
A300 m/sB320 m/sC280 m/sD340.8m/s - Question 3 - Select One
A detector is released from rest over a source of sound of frequency f0=103Hz. The frequency observed by the detector at time t is plotted in the graph. The speed of sound in air is (g=10m/s2)
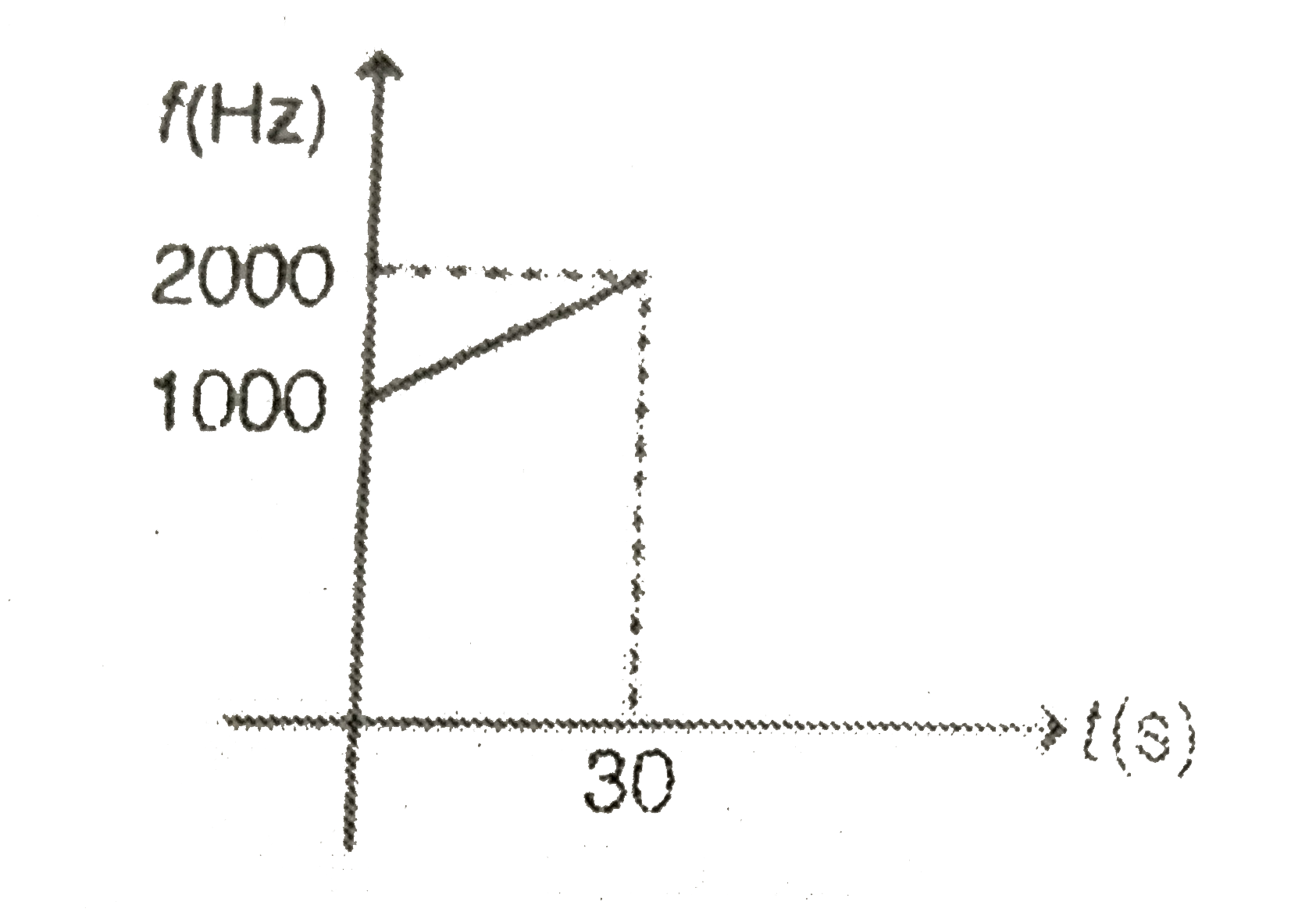 A330m/sB350 m/sC300 m/sD310 m/s
A330m/sB350 m/sC300 m/sD310 m/s
A stone is dropped in a well 107 m deep. If the splash is heard 5 seconds after the stone is dropped, then calculate the speed of sound in air.
View SolutionA stone is dropped into a well. The splash is heard 3.35 s later. What is the depth of the well?
View SolutionAt SPT, The speed of sound in hydrogen is 1324 m/s then the speed of sound in air
View SolutionAn echo of sound produced by a person, is heard from a distant tower after an interval of 1.5 s. If the speed of sound in air is 330ms−1, then the distance of the tower from the person is _______ m.
View SolutionAn echo of sound produced by a person, is heard from a distant tower after an interval of 1.5 s. If the speed of sound in air is 330ms−1, then the distance of the tower from the person is _______ m.
View Solution

