Which of the following pairs have same dimensional formula for both the quantities ?
(i) kinetic energy and torque
(ii) resistance and inductance
(iii) Young's Modulus and Pressure
(i) only
(ii) only
(i) and (iii) only
all the above
The correct Answer is:C
To solve the problem of identifying which pairs of quantities have the same dimensional formula, we will analyze each pair step by step.
Step 1: Analyze Kinetic Energy and Torque
Kinetic Energy (KE) is given by the formula:
KE=12mv2
Where:
- m is mass (dimension (M))
- v is velocity (dimension (LT−1))
The dimensional formula for kinetic energy is:
(KE)=(M)(L2T−2)=(ML2T−2)
Torque (τ) is given by the formula:
τ=r×F
Where:
- r is the distance (dimension (L))
- F is force (dimension (MLT−2))
The dimensional formula for torque is:
(τ)=(L)(MLT−2)=(ML2T−2)
Conclusion for Step 1:
Both kinetic energy and torque have the same dimensional formula:
(ML2T−2)
Step 2: Analyze Resistance and Inductance
Resistance (R) is defined by Ohm's law:
R=VI
Where:
- V is voltage (dimension (ML2T−3I−1))
- I is current (dimension (I))
The dimensional formula for resistance is:
(R)=(ML2T−3I−1)(I)=(ML2T−3I−2)
Inductance (L) is given by:
L=VdIdt
Where:
- dIdt has the dimension of current change over time, which is (IT−1)
The dimensional formula for inductance is:
(L)=(ML2T−3I−1)(IT−1)=(ML2T−2I−2)
Conclusion for Step 2:
Resistance and inductance have different dimensional formulas:
- Resistance: (ML2T−3I−2)
- Inductance: (ML2T−2I−2)
Step 3: Analyze Young's Modulus and Pressure
Young's Modulus (Y) is defined as:
Y=StressStrain
Where:
- Stress is defined as force per unit area: Stress=FA
- Strain is dimensionless.
Thus, the dimensional formula for Young's Modulus is:
(Y)=(F)(A−1)1=(MLT−2)(L2)=(ML−1T−2)
Pressure (P) is defined as:
P=FA
Where:
- Force is (MLT−2) and area is (L2).
The dimensional formula for pressure is:
(P)=(F)(A)=(MLT−2)(L2)=(ML−1T−2)
Conclusion for Step 3:
Both Young's Modulus and Pressure have the same dimensional formula:
(ML−1T−2)
Final Conclusion
The pairs with the same dimensional formulas are:
- (i) Kinetic Energy and Torque: Same dimensional formula (ML2T−2)
- (iii) Young's Modulus and Pressure: Same dimensional formula (ML−1T−2)
Thus, the correct options are (i) and (iii).
KE=12mv2
Where:
- m is mass (dimension (M))
- v is velocity (dimension (LT−1))
(KE)=(M)(L2T−2)=(ML2T−2)
τ=r×F
Where:
- r is the distance (dimension (L))
- F is force (dimension (MLT−2))
(τ)=(L)(MLT−2)=(ML2T−2)
Both kinetic energy and torque have the same dimensional formula:
(ML2T−2)
R=VI
Where:
- V is voltage (dimension (ML2T−3I−1))
- I is current (dimension (I))
(R)=(ML2T−3I−1)(I)=(ML2T−3I−2)
L=VdIdt
Where:
- dIdt has the dimension of current change over time, which is (IT−1)
(L)=(ML2T−3I−1)(IT−1)=(ML2T−2I−2)
Resistance and inductance have different dimensional formulas:
- Resistance: (ML2T−3I−2)
- Inductance: (ML2T−2I−2)
Y=StressStrain
Where:
- Stress is defined as force per unit area: Stress=FA
- Strain is dimensionless.
(Y)=(F)(A−1)1=(MLT−2)(L2)=(ML−1T−2)
P=FA
Where:
- Force is (MLT−2) and area is (L2).
(P)=(F)(A)=(MLT−2)(L2)=(ML−1T−2)
Both Young's Modulus and Pressure have the same dimensional formula:
(ML−1T−2)
The pairs with the same dimensional formulas are:
- (i) Kinetic Energy and Torque: Same dimensional formula (ML2T−2)
- (iii) Young's Modulus and Pressure: Same dimensional formula (ML−1T−2)
Similar Questions
Knowledge Check
- Question 1 - Select One
Which of the following pair have same dimensional formula?
AAngular momentum, TorqueBTorque, WorkCplank constant, Boltzmann constantDGas constant, Pressure - Question 2 - Select One
Which of the following have same dimensional formula as that of the surface tension ?
AViscosityBPlanck's constantCForce constantDYoung's modulus - Question 3 - Select One
In which of the following pair of compounds having same general formula ?
A B
B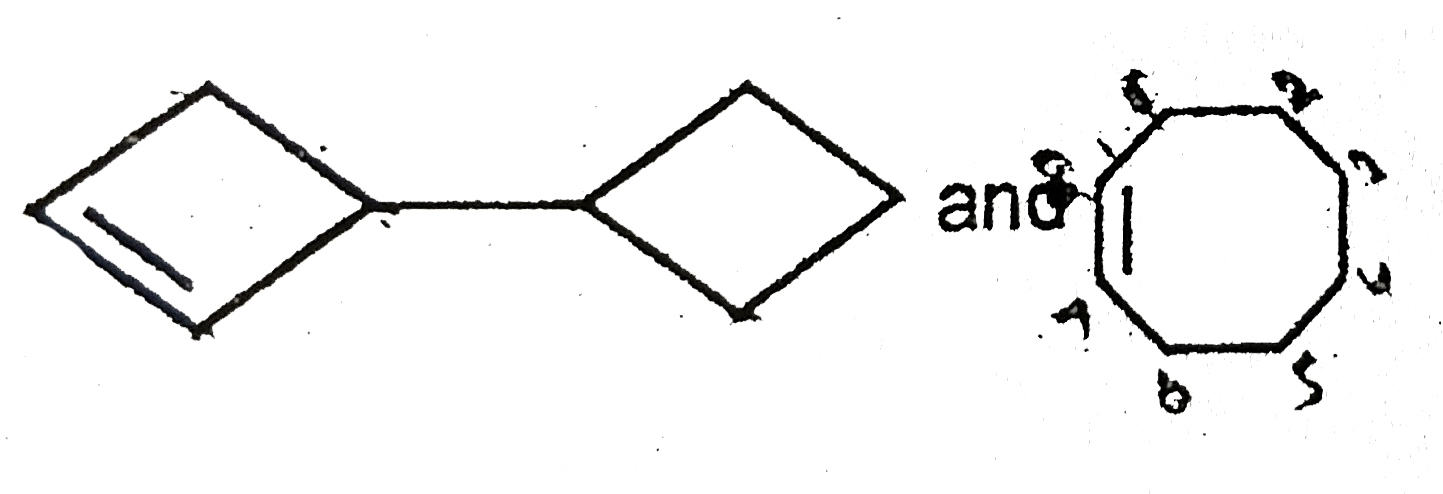 CCH3−C≡C−CH3andCH2=CH−CH=CH−CH=CH2D
CCH3−C≡C−CH3andCH2=CH−CH=CH−CH=CH2D
Which of the following pairs have the same general molecular formula CnH2n+2O.
View SolutionWhich of the following pairs of physical quantities does not have same dimensional formula?
View SolutionWhich of the following pairs of componds have the same general formula CnH2n ?
View SolutionWhich of the following pairs of physical quantites does not have same dimensional formula ?
View SolutionWhich of the following pairs have the same dimensions ?
(L = inductance , C = capacitance , R = resistance)View Solution

