The magnetic field midway between two parallel current carrying wires, carrying currents I and 2i in same direction is B. If the current in the wire with current I is switched off, the magnetic field will become
B/3
2B
B/2
B/4
The correct Answer is:B
To solve the problem, we need to analyze the magnetic fields produced by two parallel wires carrying currents I and 2I in the same direction.
1. Identify the Magnetic Fields:
- For a long straight wire, the magnetic field at a distance r from the wire carrying current I is given by the formula:
B=μ0I2πr
- For the first wire carrying current I:
B1=μ0I2πr
- For the second wire carrying current 2I:
B2=μ0(2I)2πr=μ02I2πr
2. Determine the Direction of the Magnetic Fields:
- Using the right-hand rule, the magnetic field B1 due to the first wire (current I) will be directed downwards at the midpoint between the two wires.
- The magnetic field B2 due to the second wire (current 2I) will be directed upwards at the same point.
3. Calculate the Net Magnetic Field:
- Since B2 is greater than B1, the net magnetic field B at the midpoint is:
B=B2−B1=μ02I2πr−μ0I2πr=μ0I2πr
- Thus, the net magnetic field B is:
B=μ0I2πr
4. Switch Off the Current in the First Wire:
- When the current I in the first wire is switched off, the magnetic field due to the first wire becomes zero.
- The magnetic field at the midpoint is now only due to the second wire with current 2I:
B′=B2=μ0(2I)2πr
5. Express the New Magnetic Field in Terms of B:
- We previously found that:
B=μ0I2πr
- Therefore, the new magnetic field B′ can be expressed as:
B′=2×μ0I2πr=2B
Conclusion:
When the current in the wire carrying current I is switched off, the magnetic field at the midpoint becomes 2B.
- For a long straight wire, the magnetic field at a distance r from the wire carrying current I is given by the formula:
B=μ0I2πr
- For the first wire carrying current I:
B1=μ0I2πr
- For the second wire carrying current 2I:
B2=μ0(2I)2πr=μ02I2πr
- Using the right-hand rule, the magnetic field B1 due to the first wire (current I) will be directed downwards at the midpoint between the two wires.
- The magnetic field B2 due to the second wire (current 2I) will be directed upwards at the same point.
- Since B2 is greater than B1, the net magnetic field B at the midpoint is:
B=B2−B1=μ02I2πr−μ0I2πr=μ0I2πr
- Thus, the net magnetic field B is:
B=μ0I2πr
- When the current I in the first wire is switched off, the magnetic field due to the first wire becomes zero.
- The magnetic field at the midpoint is now only due to the second wire with current 2I:
B′=B2=μ0(2I)2πr
- We previously found that:
B=μ0I2πr
- Therefore, the new magnetic field B′ can be expressed as:
B′=2×μ0I2πr=2B
When the current in the wire carrying current I is switched off, the magnetic field at the midpoint becomes 2B.
Similar Questions
Knowledge Check
Two parallel wires carrying current I and 2I in same direction have magnetic field B at the midpoint between them. If the current 2I is switched off, the magnetic field at that point will be
AB/2BBC2BD3BThe magnetic field at a point midway between two parallel long wires carrying currents in the same direction is 10μT. If the direction of the smaller current among them is reversed, the field becomes 30μT . The ratio of the larger to the smaller current in them is
A3:1B2:1C4:1D3:2For the current carrying wire, show that the magnetic field at P is
 Aμ0i4π(3π2R+√2d)⊙Bμ0i4π(3π2R−√2d)⊗Cμ0i4π(3π2R−√2d)⊙Dμ0i4π(3π2R+√2d)⊗
Aμ0i4π(3π2R+√2d)⊙Bμ0i4π(3π2R−√2d)⊗Cμ0i4π(3π2R−√2d)⊙Dμ0i4π(3π2R+√2d)⊗
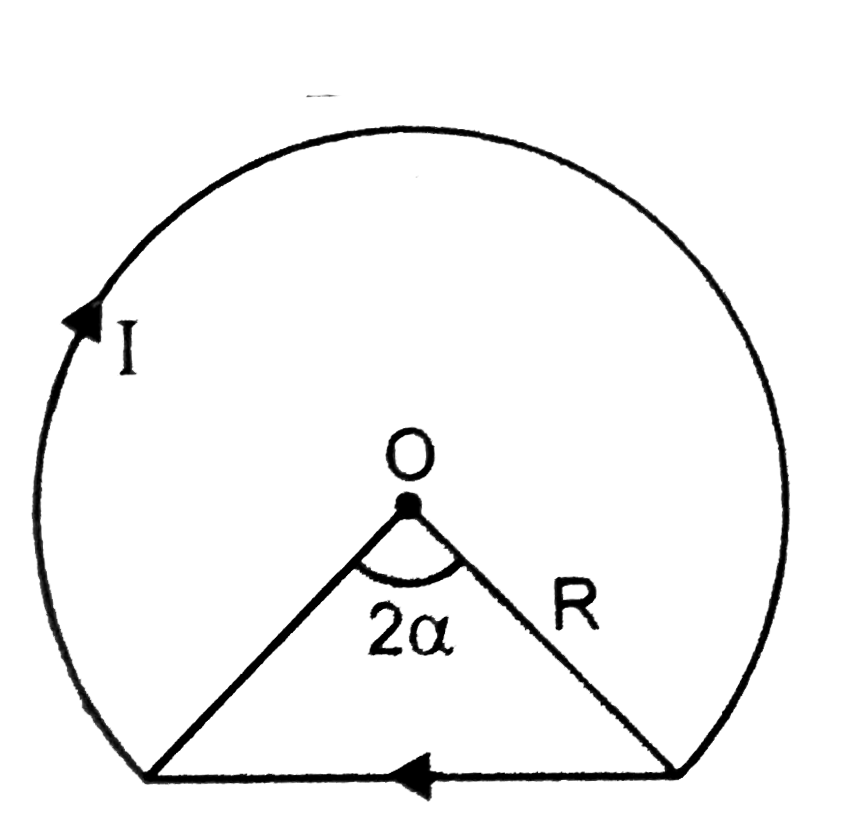
The magnetic field intensity due to a thin wire carrying current I shown in figure, is
View SolutionThe direction of magnetic field at a point due to an infinitely long wire carrying current is
View SolutionTwo infinitely long wires each carrying current I along the same direction are made into the geometry as shown in the figure. The magnetic field at the point P is

View SolutionThe magnetic field at a distance r from a long wire carrying current i is 0.4 T. The magnetic field at a distance 2r is
View SolutionAlong the direction of current carrying wire, the value of magnetic field is ?
View Solution
