Compute the Gibbs energy change of the reaction at 27∘C for the combustion of methane.
CH4(g)+2O2(g)→CO2(g)+2H2O(l)ΔfHΘ(in kh mol1) −74.8 − −393.5 −285.8SΘm(in J K^(-1)mol−1) 186 205 214 70
To compute the Gibbs energy change (ΔG) for the combustion of methane at 27°C, we will follow these steps:
Step 1: Write the Reaction
The combustion of methane can be represented as:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
Step 2: Identify Given Data
From the problem statement, we have the following data:
- Enthalpy of formation (ΔfH) in kJ/mol:
- CH₄(g): -74.8 kJ/mol
- O₂(g): 0 kJ/mol (element in its standard state)
- CO₂(g): -393.5 kJ/mol
- H₂O(l): -285.8 kJ/mol
- Standard molar entropy (S) in J/(K·mol):
- CH₄(g): 186 J/(K·mol)
- O₂(g): 205 J/(K·mol)
- CO₂(g): 214 J/(K·mol)
- H₂O(l): 70 J/(K·mol)
Step 3: Calculate ΔH for the Reaction
Using the formula:
ΔH=∑(ΔH of products)−∑(ΔH of reactants)
Calculating for products:
- For CO₂: -393.5 kJ/mol
- For 2H₂O: 2 × (-285.8 kJ/mol) = -571.6 kJ/mol
Total for products:
−393.5+(−571.6)=−965.1 kJ/mol
Calculating for reactants:
- For CH₄: -74.8 kJ/mol
- For 2O₂: 2 × 0 = 0 kJ/mol
Total for reactants:
−74.8+0=−74.8 kJ/mol
Now, substituting these values into the ΔH equation:
ΔH=−965.1−(−74.8)=−965.1+74.8=−890.3 kJ/mol
Step 4: Calculate ΔS for the Reaction
Using the formula:
ΔS=∑(S of products)−∑(S of reactants)
Calculating for products:
- For CO₂: 214 J/(K·mol)
- For 2H₂O: 2 × 70 J/(K·mol) = 140 J/(K·mol)
Total for products:
214+140=354 J/(K⋅mol)
Calculating for reactants:
- For CH₄: 186 J/(K·mol)
- For 2O₂: 2 × 205 J/(K·mol) = 410 J/(K·mol)
Total for reactants:
186+410=596 J/(K⋅mol)
Now, substituting these values into the ΔS equation:
ΔS=354−596=−242 J/(K⋅mol)
Step 5: Convert ΔS to kJ
Since ΔH is in kJ, we need to convert ΔS to kJ:
ΔS=−242 J/(K⋅mol)=−0.242 kJ/(K⋅mol)
Step 6: Calculate ΔG
Using the Gibbs free energy equation:
ΔG=ΔH−TΔS
Where:
- T = 27°C = 300 K (convert to Kelvin by adding 273)
Now substituting the values:
ΔG=−890.3 kJ/mol−(300 K×−0.242 kJ/(K⋅mol))
ΔG=−890.3+72.6
ΔG=−817.7 kJ/mol
Final Answer
The Gibbs energy change (ΔG) for the combustion of methane at 27°C is approximately:
ΔG≈−817.7 kJ/mol
---
The combustion of methane can be represented as:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
From the problem statement, we have the following data:
- Enthalpy of formation (ΔfH) in kJ/mol:
- CH₄(g): -74.8 kJ/mol
- O₂(g): 0 kJ/mol (element in its standard state)
- CO₂(g): -393.5 kJ/mol
- H₂O(l): -285.8 kJ/mol
- CH₄(g): 186 J/(K·mol)
- O₂(g): 205 J/(K·mol)
- CO₂(g): 214 J/(K·mol)
- H₂O(l): 70 J/(K·mol)
Using the formula:
ΔH=∑(ΔH of products)−∑(ΔH of reactants)
- For CO₂: -393.5 kJ/mol
- For 2H₂O: 2 × (-285.8 kJ/mol) = -571.6 kJ/mol
−393.5+(−571.6)=−965.1 kJ/mol
- For CH₄: -74.8 kJ/mol
- For 2O₂: 2 × 0 = 0 kJ/mol
−74.8+0=−74.8 kJ/mol
ΔH=−965.1−(−74.8)=−965.1+74.8=−890.3 kJ/mol
Using the formula:
ΔS=∑(S of products)−∑(S of reactants)
- For CO₂: 214 J/(K·mol)
- For 2H₂O: 2 × 70 J/(K·mol) = 140 J/(K·mol)
214+140=354 J/(K⋅mol)
- For CH₄: 186 J/(K·mol)
- For 2O₂: 2 × 205 J/(K·mol) = 410 J/(K·mol)
186+410=596 J/(K⋅mol)
ΔS=354−596=−242 J/(K⋅mol)
Since ΔH is in kJ, we need to convert ΔS to kJ:
ΔS=−242 J/(K⋅mol)=−0.242 kJ/(K⋅mol)
Using the Gibbs free energy equation:
ΔG=ΔH−TΔS
- T = 27°C = 300 K (convert to Kelvin by adding 273)
ΔG=−890.3 kJ/mol−(300 K×−0.242 kJ/(K⋅mol))
ΔG=−890.3+72.6
ΔG=−817.7 kJ/mol
The Gibbs energy change (ΔG) for the combustion of methane at 27°C is approximately:
ΔG≈−817.7 kJ/mol
Similar Questions
Compute the standard free energy of the reaction at 27∘C for the combustion of methane using the given data.
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
SpeciesCH4O2CO2H2OΔfH∘/(kJmol−1)−74.8−−393.5−285.8S∘/(JK−1mol−1)18620521470View SolutionCompute the standard free enegry of the reaction at 27∘C for the combustion fo methane using the give data:
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
SpeciesCH4O2CO22H2O(l)ΔfHΘ(kJmol−1)−74.8−−393.5−285.8SΘ(JK−1mol−1)18620521470View Solution
Knowledge Check
- Question 1 - Select One
Estimate the enthalpy of combustion of methane in KJ .mol−1
CH4(g)+2O2(g)→CO2(g)+2H2O(g)
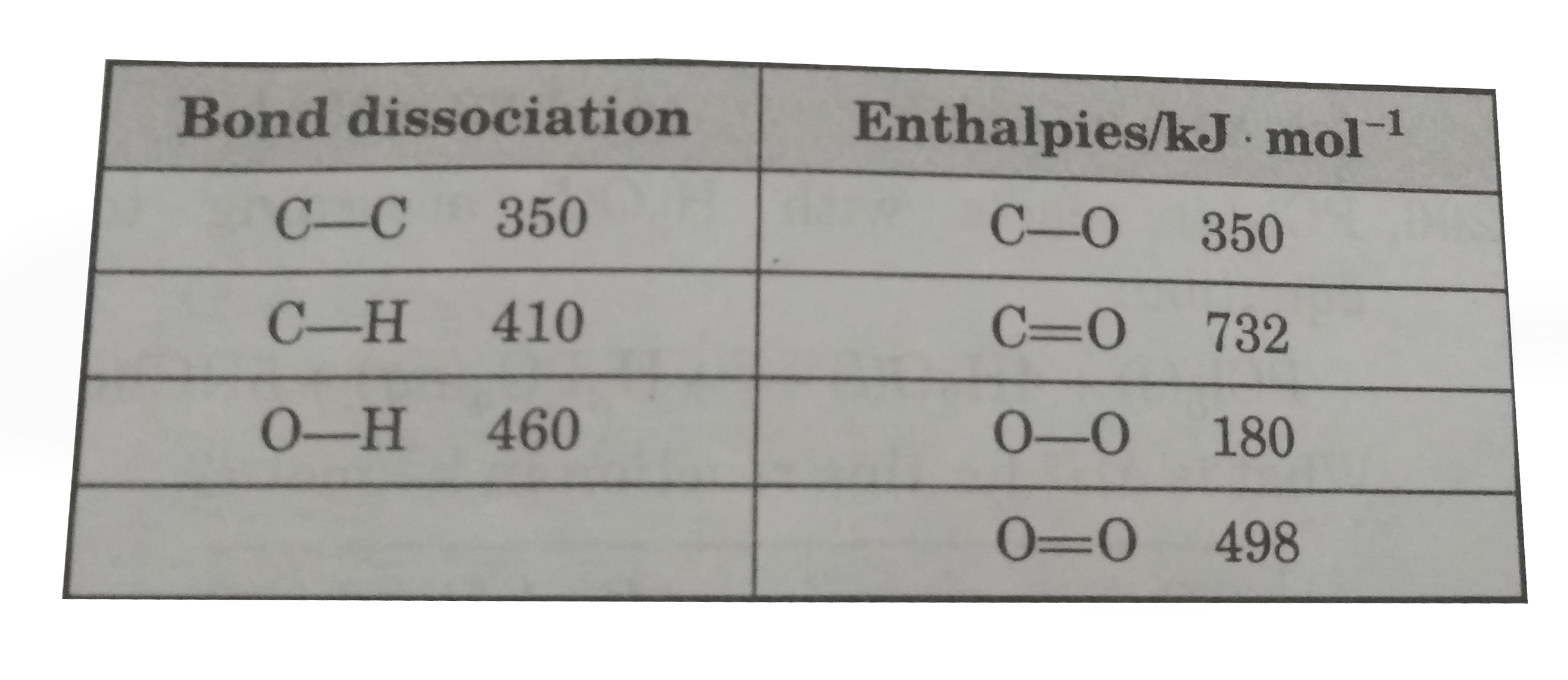 A668B540C−540D−668
A668B540C−540D−668 - Question 2 - Select One
The thermochemical reaction
CH4(g)+2O2(g)→CO2(g)+2H2O(l)ΔC_(C)H=−890.3KJ mol−1 is an example of -Aenthalpy of atomizationBenthalpy of combustionCenthalpy of solutionDNone of these
Compute the standard free energy of the reaction at 27∘C for the combustion of methane using the given data.
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
SpeciesCH4O2CO2H2OΔfH∘/(kJmol−1)−74.8−−393.5−285.8S∘/(JK−1mol−1)18620521470View SolutionWhat is the value of ΔH in the following reaction at 300 K ?
CH4(g)2O2(g)→CO2(g)+2H2O(l),ΔU=885389J/mol.View SolutionCalculate the standard Gibbs enegry change for the combustion of α−D glucose at 300K.
C6H12O6(s)+6O2(g)→6CO2(g)+6H2O(l)
Given the standard enthalpies of formation (kJmol−1)
C6H12O6=−1274.5,CO2=−393.5,H2O=−285.8.
Entropies (JKmol−1)
C6H12O6=212.1,O1=205.0,CO2=213,H2O=69.9View SolutionCalculate the standard free energy change for the formation of methane at 300K:
C(graphite)+2H2(g)→CH4(g)
The following data are given:
ΔfHΘ(kJmol−1):CH4(g)=−74.81
ΔfSΘ(kJmol−1):C(graphite)=5.70,H2(g)=130.7CH4(g)=186.3View SolutionThe enthalpy of reaction for thr equation
2H2(g)+O2(g)→2H2O(l) is ΔH∘=−572kjmol
what will be the standard enthalpy for the formation of H2O(l)?View SolutionCalculate the enthalpy change for the reaction
CH4(g)+2O2(g)→CO2(g)+2H2O(l)
The enethalpy of formation of CH4(g),CO2(g) and H2O(l) are -74.8 kJ mol−1, -393.5 kJ mol−1 and −285.8kJmol−1 respectively.View Solution

