Question

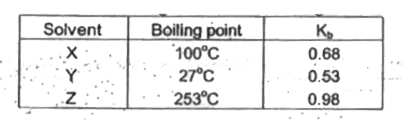
Match the following if the molar mass of X, Y and Z are the same. $\begin{array}{ccc}\text{solvent}& T_b{/}^{\circ }C& K_b/kgKmo{l}^{-1}\\ X& 127& 0.73\\ Y& 27& 0.53\\ Z& 253& 0.98\end{array}$

Explanation

To solve the problem of matching the solvents X, Y, and Z based on their boiling points (T_b) and boiling point elevation constants (K_b), we can follow these steps:

1. Understand the Relationship: According to the problem, we know that the molar mass of X, Y, and Z is the same. We also know that K_b is directly proportional to T_b based on the formula and Trouton's rule.

2. List the Given Data:
- For solvent X: T_b = 127°C, K_b = 0.73 kg K mol^(-1)
- For solvent Y: T_b = 27°C, K_b = 0.53 kg K mol^(-1)
- For solvent Z: T_b = 253°C, K_b = 0.98 kg K mol^(-1)

3. Determine the Order of T_b:
- The boiling points in ascending order are:
- Y: 27°C
- X: 127°C
- Z: 253°C

4. Determine the Order of K_b:
- The K_b values in ascending order are:
- Y: 0.53 kg K mol^(-1)
- X: 0.73 kg K mol^(-1)
- Z: 0.98 kg K mol^(-1)

5. Match the Solvents:
- Since K_b is directly proportional to T_b:
- The lowest T_b (Y: 27°C) corresponds to the lowest K_b (Y: 0.53 kg K mol^(-1)).
- The middle T_b (X: 127°C) corresponds to the middle K_b (X: 0.73 kg K mol^(-1)).
- The highest T_b (Z: 253°C) corresponds to the highest K_b (Z: 0.98 kg K mol^(-1)).

6. Final Matching:
- Therefore, the matches are:
- X: 127°C, K_b = 0.73
- Y: 27°C, K_b = 0.53
- Z: 253°C, K_b = 0.98

Final Answer:
- X matches with 127°C and K_b = 0.73
- Y matches with 27°C and K_b = 0.53
- Z matches with 253°C and K_b = 0.98