Question

Using the concept of integration evaluate an area by definite integral

Explanation

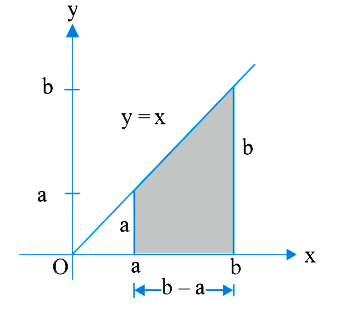
We sketch the region under the curvey `y=x,alexleb` (figure) and see that it is a trapezoid with height `(b-a)` and bases a and b. The value of the integrl is the area of this trapezoid :
`int_(a)^(b)xdx=(b-a)(a+b)/(2)=(b^(2))/(2)-(a^(2))/(2)`
Notice that `x^(2)//2` is an antiderivative of x, further evidence of a connection between antiderivatives and summation.