A trapezium ABCD is inscribed into a semicircle of radius l so that the base AD of the trapezium is a diameter and the vertices B & C lie on the circumference . Find the base angle θ of the trapezium ABCD which has the greatest perimeter .
To solve the problem of finding the base angle θ of the trapezium ABCD inscribed in a semicircle of radius l that maximizes the perimeter, we can follow these steps:
Step 1: Understand the Geometry
We have a semicircle with diameter AD and points B and C on the circumference. The trapezium ABCD has AD as its base.
Step 2: Define the Variables
Let the radius of the semicircle be R (which is given as l). The angle θ is the base angle at points A and D.
Step 3: Calculate Lengths
- The length of the base AD is equal to the diameter of the semicircle, which is 2R.
- The heights from points B and C to the base AD can be expressed in terms of θ:
- The height BE from B to AD is Rsinθ.
- The height CF from C to AD is also Rsinθ.
Step 4: Calculate the Lengths of Sides AB and CD
Using the cosine of the angle:
- The length AB=Rcosθ and CD=Rcosθ.
Step 5: Calculate the Length of BC
Using the fact that BC=AD−(AB+CD):
- BC=2R−2Rcosθ=2R(1−cosθ).
Step 6: Calculate the Perimeter P
The perimeter P of trapezium ABCD is given by:
P=AB+BC+CD+AD=Rcosθ+2R(1−cosθ)+Rcosθ+2R
Simplifying:
P=2R+2R(1−cosθ)=2R(2−cosθ)
Step 7: Differentiate the Perimeter
To find the angle θ that maximizes the perimeter, we differentiate P with respect to θ:
dPdθ=2Rsinθ
Setting the derivative equal to zero to find critical points:
2Rsinθ=0
This gives sinθ=0 or θ=0 or θ=π.
Step 8: Check the Maximum Perimeter
To find the maximum perimeter, we also consider the second derivative test or evaluate the function at critical points. The feasible angle for a trapezium is θ=π3.
Conclusion
The base angle θ of trapezium ABCD that maximizes the perimeter is:
θ=π3
We have a semicircle with diameter AD and points B and C on the circumference. The trapezium ABCD has AD as its base.
Let the radius of the semicircle be R (which is given as l). The angle θ is the base angle at points A and D.
- The length of the base AD is equal to the diameter of the semicircle, which is 2R.
- The heights from points B and C to the base AD can be expressed in terms of θ:
- The height BE from B to AD is Rsinθ.
- The height CF from C to AD is also Rsinθ.
Using the cosine of the angle:
- The length AB=Rcosθ and CD=Rcosθ.
Using the fact that BC=AD−(AB+CD):
- BC=2R−2Rcosθ=2R(1−cosθ).
The perimeter P of trapezium ABCD is given by:
P=AB+BC+CD+AD=Rcosθ+2R(1−cosθ)+Rcosθ+2R
Simplifying:
P=2R+2R(1−cosθ)=2R(2−cosθ)
To find the angle θ that maximizes the perimeter, we differentiate P with respect to θ:
dPdθ=2Rsinθ
Setting the derivative equal to zero to find critical points:
2Rsinθ=0
This gives sinθ=0 or θ=0 or θ=π.
To find the maximum perimeter, we also consider the second derivative test or evaluate the function at critical points. The feasible angle for a trapezium is θ=π3.
The base angle θ of trapezium ABCD that maximizes the perimeter is:
θ=π3
Topper's Solved these Questions
- MAXIMA AND MINIMA BOOK - MOTIONCHAPTER - MAXIMA AND MINIMA EXERCISE - EXERCISE -1 (SECTION - A)
7 Videos
- MAXIMA AND MINIMA BOOK - MOTIONCHAPTER - MAXIMA AND MINIMA EXERCISE - EXERCISE -1 (SECTION -B)
16 Videos
- MATRICES BOOK - MOTIONCHAPTER - MATRICES EXERCISE - Exercise - 4 (Level-II)
28 Videos
- METHOD OF DIFFERENTIATIONBOOK - MOTIONCHAPTER - METHOD OF DIFFERENTIATIONEXERCISE - EXERCISE - 4 LEVEL -II
5 Videos
Similar Questions
ABCD is a trapezium such that AB||CD, ∠A : ∠D = 2 :1, ∠B : ∠C = 7 : 5. Find the angles of the trapezium.
View SolutionA current I=√2 A flows in a circuit having the shape of isosceles trapezium. The ratio of the bases of the trapezium is 2. Find the magnetic induction B at symmetric point O in the plane of the trapezium. The length of the smaller base of the trapezium is 100mm and the distance r=50mm.
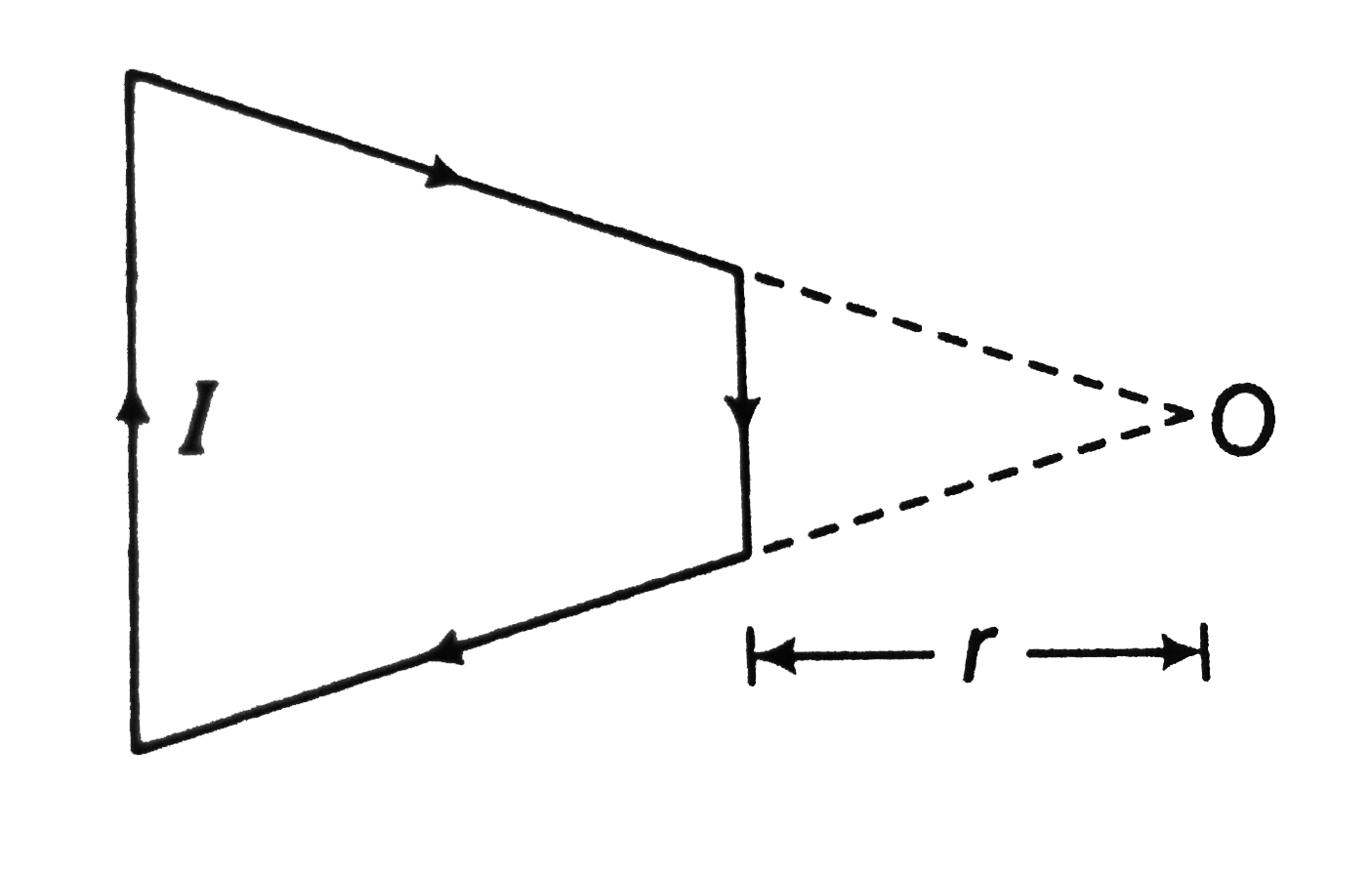
View Solution
Knowledge Check
In the given figure , ABCD is a trapezium in which the parallel sides AB,CD are both perpendicular to BC. Find the area of the trapezium :
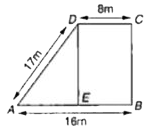 A140m2B168m2C180m2D156.4m2
A140m2B168m2C180m2D156.4m2In an isosceles trapezium ABCD, ∠C is equal to:
 A115∘B65∘C105∘D75∘
A115∘B65∘C105∘D75∘In the given pattern, eight isosceles trapeziums surround a regular octagon. The measure of ∠B in the trapezium ABCD is :
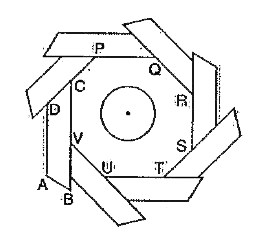 A55∘B40∘C45∘D60∘
A55∘B40∘C45∘D60∘
A circle is inscribed in a trapezium in which one of the non-parallel sides is perpendicular to the two parallel sides. Then A) the diameter of the inscribed circle is the geometric mean of the lengths of the parallel sides B) the diameter of the inscribed circle is the harmonic mean of the lengths of the parallel sides C) the area of the trapezium is the area of the rectangle having lengths of its sides as the lengths of the parallel sides of the trapezium D) the area of the trapezium is half the area of the rectangle having lengths of its sides as the lengths of the parallel sides of the trapezium
View SolutionA right angled trapezium is circumscribed about a circle. Find the radius of the circle. If the lengtyhs of the bases (i.e.parallel sides ) are equal to a and b.
View SolutionABCD is a cyclic trapezium with ADBC. If ∠B=700, determine other three angles of the trapezium.
View SolutionABCD is a trapezium in which AB || DC, ABデ78 cm, CD = 52 cm. AD = 28 cm and BC =30cm . Find the area of the trapezium.
View SolutionABCD is a trapezium in which AB∣∣DCand∠A=∠B=45∘. Find angles C and D of the trapezium.
View Solution
