A money of mass 40 kg climbs up a rope, of breaking load 600 N hanging from a ceiling. If it climbs up the rope with the maximum possible acceleration, then the time taken by monkey to climb up is [length of rope is 10 m]
2 s
1 s
4 s
3 s
The correct Answer is:A
To solve the problem step by step, we need to analyze the forces acting on the monkey and then use kinematic equations to find the time taken to climb the rope.
Step 1: Determine the maximum force the monkey can exert.
The breaking load of the rope is given as 600 N. This means the maximum tension (T) in the rope while the monkey is climbing cannot exceed 600 N.
Step 2: Calculate the weight of the monkey.
The weight (W) of the monkey can be calculated using the formula:
W=m⋅g
where:
- m=40kg (mass of the monkey)
- g=9.8m/s2 (acceleration due to gravity)
Calculating the weight:
W=40kg⋅9.8m/s2=392N
Step 3: Determine the net force acting on the monkey.
The net force (F_net) acting on the monkey when it climbs with maximum acceleration can be calculated using:
Fnet=T−W
Given that the maximum tension T=600N:
Fnet=600N−392N=208N
Step 4: Calculate the maximum acceleration of the monkey.
Using Newton's second law:
Fnet=m⋅a
We can rearrange this to find the acceleration (a):
a=Fnetm=208N40kg=5.2m/s2
Step 5: Use kinematic equations to find the time taken to climb the rope.
We will use the equation of motion:
s=ut+12at2
where:
- s=10m (length of the rope)
- u=0m/s (initial velocity)
- a=5.2m/s2
Substituting the known values:
10=0⋅t+12⋅5.2⋅t2
This simplifies to:
10=2.6t2
Now, solving for t2:
t2=102.6≈3.846
Taking the square root to find t:
t≈√3.846≈1.96s
Final Answer:
The time taken by the monkey to climb up the rope is approximately 1.96 seconds.
---
The breaking load of the rope is given as 600 N. This means the maximum tension (T) in the rope while the monkey is climbing cannot exceed 600 N.
The weight (W) of the monkey can be calculated using the formula:
W=m⋅g
where:
- m=40kg (mass of the monkey)
- g=9.8m/s2 (acceleration due to gravity)
W=40kg⋅9.8m/s2=392N
The net force (F_net) acting on the monkey when it climbs with maximum acceleration can be calculated using:
Fnet=T−W
Given that the maximum tension T=600N:
Fnet=600N−392N=208N
Using Newton's second law:
Fnet=m⋅a
We can rearrange this to find the acceleration (a):
a=Fnetm=208N40kg=5.2m/s2
We will use the equation of motion:
s=ut+12at2
where:
- s=10m (length of the rope)
- u=0m/s (initial velocity)
- a=5.2m/s2
10=0⋅t+12⋅5.2⋅t2
This simplifies to:
10=2.6t2
Now, solving for t2:
t2=102.6≈3.846
Taking the square root to find t:
t≈√3.846≈1.96s
The time taken by the monkey to climb up the rope is approximately 1.96 seconds.
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 2 SECTION-D :- Spring force and spring cutting problems1videos
NEWTON'S LAWS OF MOTION & FRICTION
- BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 2 SECTION-E:- Static friction, Kinetic friction13videos
NEWTON'S LAWS OF MOTION & FRICTION
- BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 2 SECTION-B :- Pulley Block System7videos
NEWTON'S LAWS OF MOTION & FRICTION
- BOOK - MOTIONCHAPTER - MODERN PHYSICS -1EXERCISE - EXERCISE-4 ( LEVEL- II)39videos
MODERN PHYSICS -1
- BOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - EXERCISE-4 ( LEVEL-II)15videos
NLM & FRICTION
Similar Questions
Knowledge Check
- Question 1 - Select One
A monkey of mass 40 kg climbs on a massless rope of breaking strength 600 N. The rope will break if the monkey. (Take g=10m/s2)
Aclimbs up with a uniform speed of 6m/sBclimbs up with an acceleration of 6m/s2Cclimbs down with an acceleration of 4m/s2Dclimbs down with a uniform speed of 5m/s - Question 2 - Select One
A monkey of mass 40kg climbs on a massless rope of breaking strenght 600N. The rope will break if the monkey (Take g=10m/s2) .
Aclimbs up with a uniform speed of 6m/sBclimbs up with an acceleration of 6m/s2Cclimbs with an acceleration of 4m/s2Dclimbs down with a uniform speed of of 5m/s2 - Question 3 - Select One
A body of mass 40 kg wants to climb up a rope hanging vertically. The rope can withstands a maximum tension of 500 N. What is the maximum acceleration with which the boy can climb rope? Tak e g=10m/s2
A1.5m/s2B2.0m/s2C2.5m/s2D3.0m/s2
If the breaking strength of string is 600N then find out the maximum acceleration of the man with which he can climb up the road
 View Solution
View SolutionA light string fixed at one end to a clamp on ground passes over a fixed pulley and hangs at the other side. It makes an angle of 30∘ with the ground. A monkey of mass 5 kg climbs up the rope. The clamp can tolerate a vertical force of 40 N only. The maximum acceleration in upward direction with which the monkey can climb safely is:
(neglect friction and take g=10m/s2)
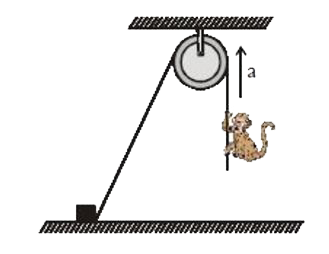 View Solution
View SolutionA massless rope of length 100m and of breaking strength 108N is hanging from the branch of a tree. A monkey of mass 6 kg is trying to climb up the rope starting from rest. Find the shortes time in which monkey can climb the full length of rope with uniform acceleration so that the string will not break ?
View SolutionA massless rope of length 100 m and of breaking strength 108 N is hanging from the branch of a tree. A monkey of mass 6 kg is trying to climb up the ropw starting from rest. Find the shortest time in which monkey can climb the full length of rope with uniform acceleration so that the string will not break ?
View SolutionA monkey of mass 30 kg climbs a rope which can withstand a maximum tension of 360 N. The maximum acceleration which this rope can tolerate for the climbing of monkey is:
(g=10m/s2)View Solution


