Solve the system of equations : z+ay+a2x+a3=0z+by+b2x+b3=0z+cy+c2x+c3=0] where a≠b≠c.
Step by step video & image solution for Solve the system of equations : z+ay+a^2x+a^3=0 z+by+b^2x+b^3=0 z+cy+c^2x+c^3=0] where a != b != c. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Solve the system of equations : z+ay+a2x+a3=0z+by+b2x+b3=0z+cy+c2x+c3=0] where a≠b≠c.
View SolutionSolve the system of equations 2x+3y−3z=0, 3x−3y+z=0 and 3x−2y−3z=0
View SolutionSolve the system of equations 2x+3y−3z=0, 3x−3y+z=0 and 3x−2y−3z=0
View SolutionThe solutions of the equations
z+ay+a2x+a3=0,z+by+b2x+b3=0,
z+cy+c2x+c3=0 are ….
View SolutionSolve the system of the equations: ax+by+cz=d, a2x+b2y+c2z=d2, a3x+b3y+c3z=d3 .
View SolutionThe solution of the system of equations a2x+ay+z=−a3,b2x+by+z=−b3,c2x+cy+z=−c3 is
(A) x=−(a+b+c),y=ab+bc+ca,z=−abc
(B) x=(a+b+c),y=ab+bc+ca,z=−abc
(C) x=−(a−b−c),y=ab+bc+ca,z=−abc
(D) None of these
View SolutionIf the system of equations ax+by+c=0,bx+cy+a=0,cx+ay+b=0 has infinitely many solutions then the system of equations (b+c)x+(c+a)y+(a+b)z=0(c+a)x+(a+b)y+(b+c)z=0(a+b)x+(b+c)y+(c+a)z=0 has
A. only one solution
B. no solution
C. infinite number of solutions
D. none of these
View SolutionIf the system of equations ax+by+c=0,bx+cy+a=0,cx+ay+b=0 has infinitely many solutions then the system of equations (b+c)x+(c+a)y+(a+b)z=0
(c+a)x+(a+b)y+(b+c)z=0
(a+b)x+(b+c)y+(c+a)z=0 has
View SolutionSuppose a, b and c are distinct and x,y and z are connected by the system of eqations x+ay+a2z=a3,x+by+b2z=b3andx+cy+c2z=c3.
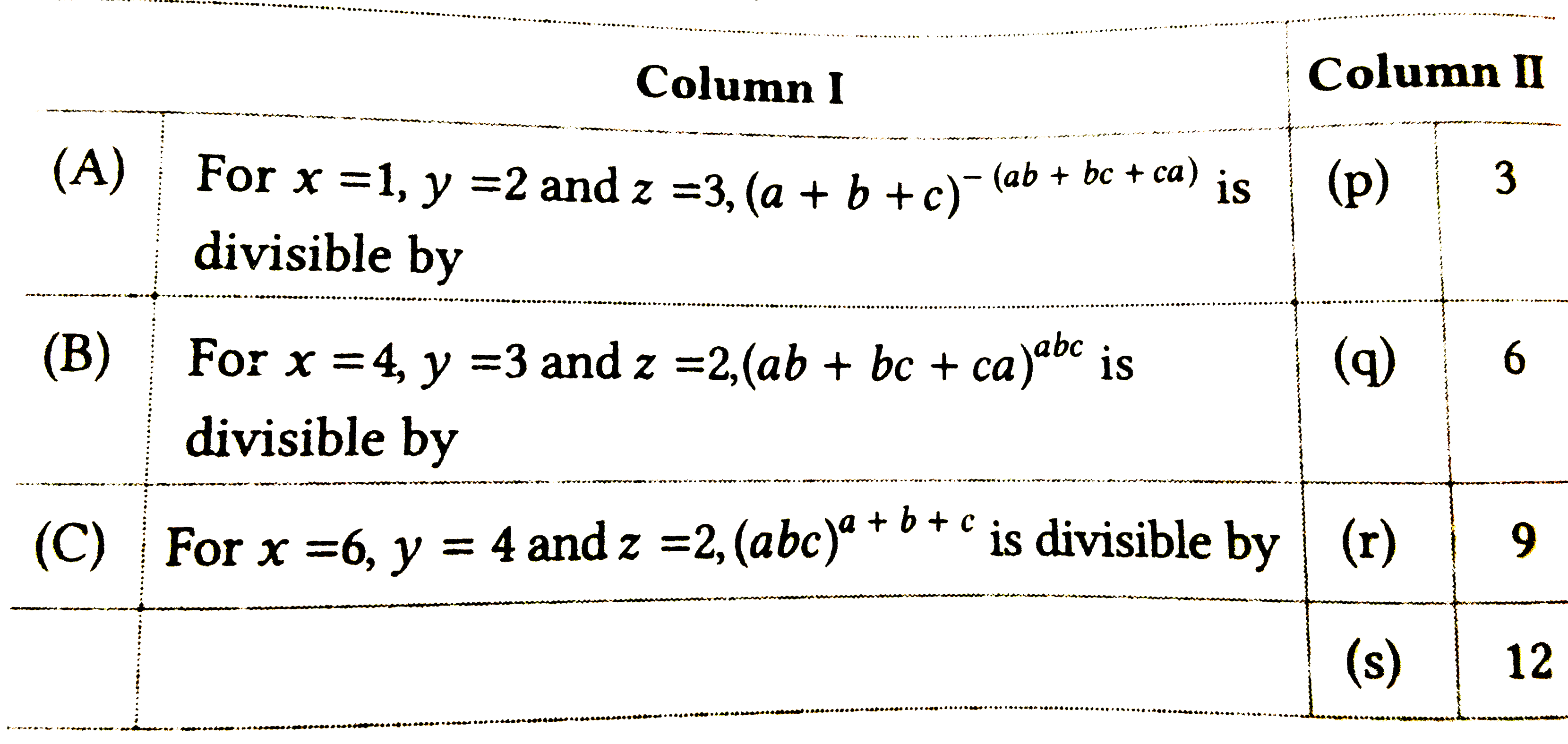
View SolutionSuppose a, b and c are distinct and x,y and z are connected by the system of eqations x+ay+a2z=a3,x+by+b2z=b3andx+cy+c2z=c3.

View Solution
