Two sources $S_{1} and S_{2}$ separated 2.0 m, vibrate according to equation $y_{1} = 0.03 sin π t and y_{2} = 0.02 sin π t where y_{1}, y_{2}$ and t are in M.K.S unit. They send out waves of velocity 1.5 m/s. Calculate the amplitude of the resultant motion of the particle co-linear with $S_{1} and S_{2}$ and located at a point to the left of $S_{1}$ .

Video Solution

Text Solution

Verified by Experts

The situation is shown in figure 6.26.
The oscillations $y_{1}$ and $y_{2}$ have amplitudes $A_{1} = 0.03 m$ and $A_{2} = 0.02$ m respectively.
The frequency of both sources is $n = \frac{ω}{2 π} = \frac{1}{2} = 0.5 H z$
Now wavelength of each wave $λ = \frac{v}{n} = \frac{1.5}{0.5} = 3.0 m$
The path differnece for all points $P_{2}$ to the right of $S_{2}$ is $Δ - (S_{1} P_{2} - S_{2} P_{2}) - S_{1} S_{2} = 2 m$ Phase difference $ϕ = \frac{2 π}{λ} \times$ path difference
= $\frac{2 π}{3} \times 2.0 = \frac{4 π}{3}$
The resultant amplitude for this point is given by $R = \sqrt{A_{1}^{2} + A_{2}^{2} + 2 A_{1} A_{2} cos ϕ}$
$\sqrt{{(0.03)}^{2} + {(0.02)}^{2} + 2 \times 0.03 \times 0.002 \times cos (4 π / 3)}$
Solving we get
$R = 0.0265 m$

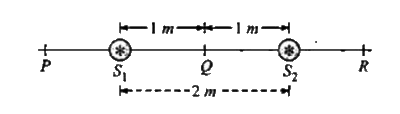
The path difference for all points p, to the left of $S_{1}$
$Δ = (S_{2} p - S_{1} P) = S_{1} S_{2} = 2.0 m$
Hence the resultant amplitude for all points to the of $S_{1}$ is also 0.0265 m $< b r > F or a p \oint Q m a y b e t w e e n$ S_(1) $and$ S_(2) $t h e p a t h d \Leftrightarrow e r e n c e i s z e r o i . e .$ phi = 0 $. H e n c e c o n s t r u c t i v e \int e r f e r \neq t a k e s p l a c e a t Q t h u s a m p l i t u d e a t t h i s p \oint i s max i μ m and g i v e n a s < b r >$ R = sqrt(A_(1)^(2) + A_(2)^(2) + 2A_(1)A_(2))

Updated on:21/07/2023

A particles executes SHM according to the equation y = 2 sin $2 π t$ , where y is displacement in m and t is time in s. The distance covered by the particle in 4 s of its motion is
A0
B2 m
C8 m
D32 m
The equation of progressive wave is $y = 0.2 sin 2 π$ $[\frac{t}{0.01} - \frac{x}{0.3}]$ , where x and y are in metre and t is in second. The velocity of propagation of the wave is
A30 m/s
B40 m/s
C300 m/s
D400 m/s
Two parallel S.H.M.s have equations $y_{1} = A_{1} sin (ω t + 2 π)$ and $y_{2} = A_{2} sin (\frac{ω}{t} + 4 π)$ . The amplitude of the resultant motion is
A $+ = (A_{1} + A_{2})$
B $+ = (A_{1} - A_{2})$
C $\sqrt{A_{1}^{2} + A_{2}^{2} + A_{1} A_{2}}$
D $\sqrt{A_{1}^{2} + A_{2}^{2}}$