Fill in the blanks in each of the followings:
xx→2−=_______
The correct Answer is:1
To solve the problem, we need to evaluate the limit of the greatest integer function (also known as the floor function) as x approaches 2 from the left (denoted as 2−).
1. Understanding the Greatest Integer Function:
The greatest integer function, denoted as ⌊x⌋, gives the largest integer less than or equal to x. For example:
- ⌊1.3⌋=1
- ⌊1.9⌋=1
- ⌊2⌋=2
2. Evaluating the Limit:
We need to find limx→2−⌊x⌋. This means we are looking at values of x that are approaching 2 from the left side (values slightly less than 2).
3. Choosing Values:
Let's consider some values of x approaching 2 from the left:
- If x=1.9, then ⌊1.9⌋=1
- If x=1.99, then ⌊1.99⌋=1
- If x=1.999, then ⌊1.999⌋=1
4. Conclusion:
As x gets closer to 2 from the left, the greatest integer function remains 1. Therefore, we conclude that:
limx→2−⌊x⌋=1
Final Answer:
limx→2−⌊x⌋=1
The greatest integer function, denoted as ⌊x⌋, gives the largest integer less than or equal to x. For example:
- ⌊1.3⌋=1
- ⌊1.9⌋=1
- ⌊2⌋=2
We need to find limx→2−⌊x⌋. This means we are looking at values of x that are approaching 2 from the left side (values slightly less than 2).
Let's consider some values of x approaching 2 from the left:
- If x=1.9, then ⌊1.9⌋=1
- If x=1.99, then ⌊1.99⌋=1
- If x=1.999, then ⌊1.999⌋=1
As x gets closer to 2 from the left, the greatest integer function remains 1. Therefore, we conclude that:
limx→2−⌊x⌋=1
limx→2−⌊x⌋=1
Topper's Solved these Questions
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - LIMITS AND DERIVATIVES EXERCISE - SHORT ANSWER TYPE QUESTIONS10videos
LIMITS AND DERIVATIVES
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - LIMITS AND DERIVATIVES EXERCISE - LONG ANSWER TYPE QUESTIONS33videos
LIMITS AND DERIVATIVES
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - INTRODUCTION TO THREE-DIMENSIONAL COORDINATE GEOMETRYEXERCISE - SHORT ANSWER TYPE QUESTIONS20videos
INTRODUCTION TO THREE-DIMENSIONAL COORDINATE GEOMETRY
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - LINEAR INEQUALITIESEXERCISE - LONG ANSWER TYPE QUESTIONS (Solve to the following system of inequalities and represent solution on number line:)3videos
LINEAR INEQUALITIES
Similar Questions
Fill in the blanks in each of the followings:
limx→2+[x]=_____View SolutionFill in the blanks in each of the followings:
limx→2+|x|x=______View SolutionFill in the blanks in the following entries:
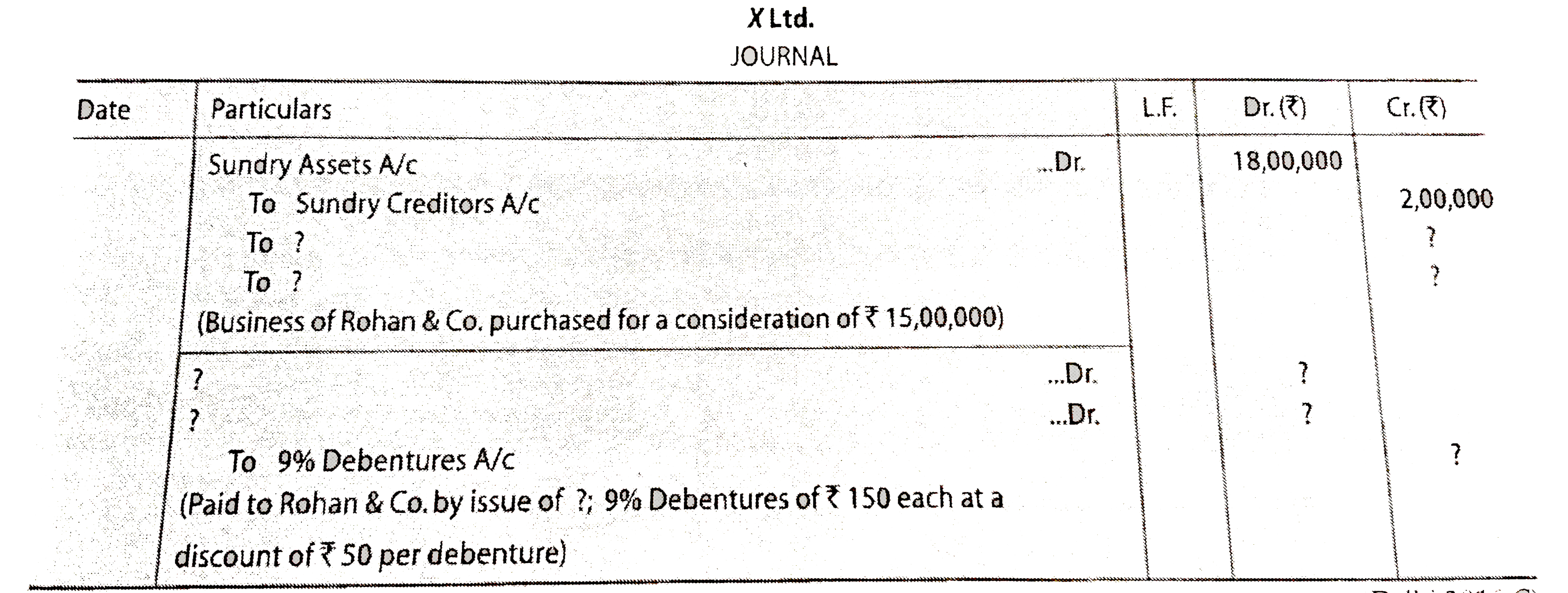 View Solution
View SolutionFill in the blanks in the following entries:
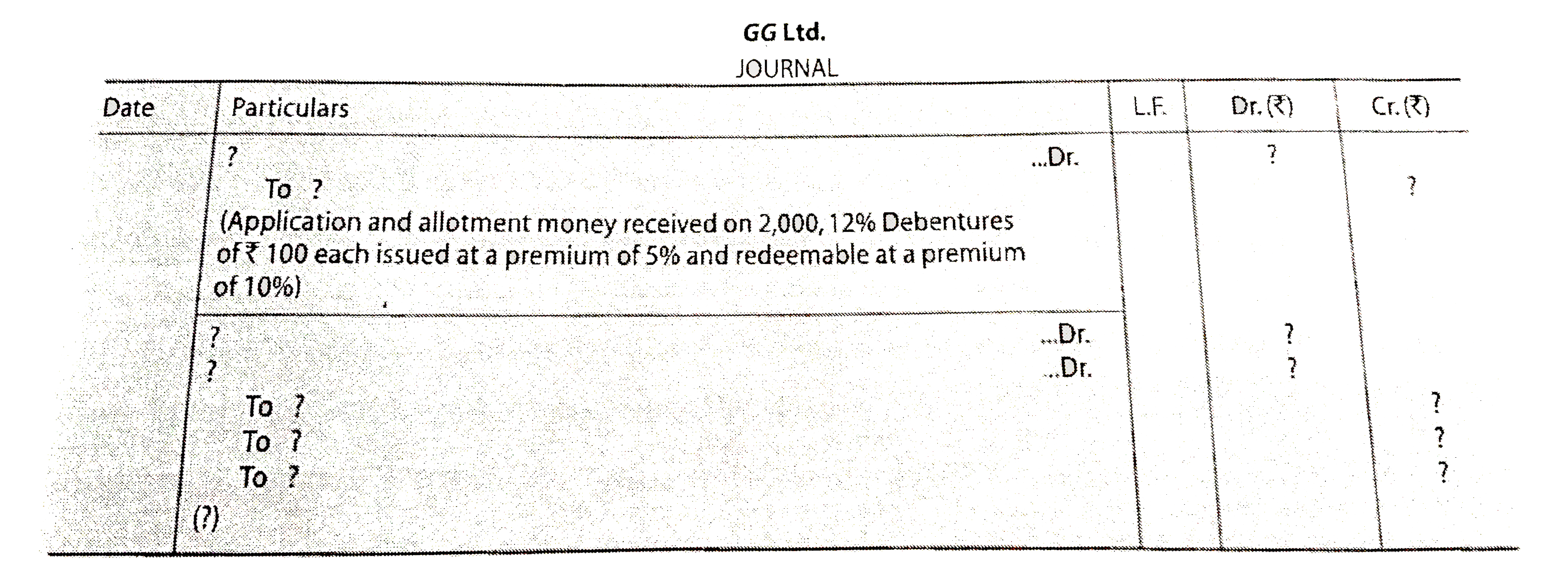 View Solution
View SolutionFill in the blanks in following table:
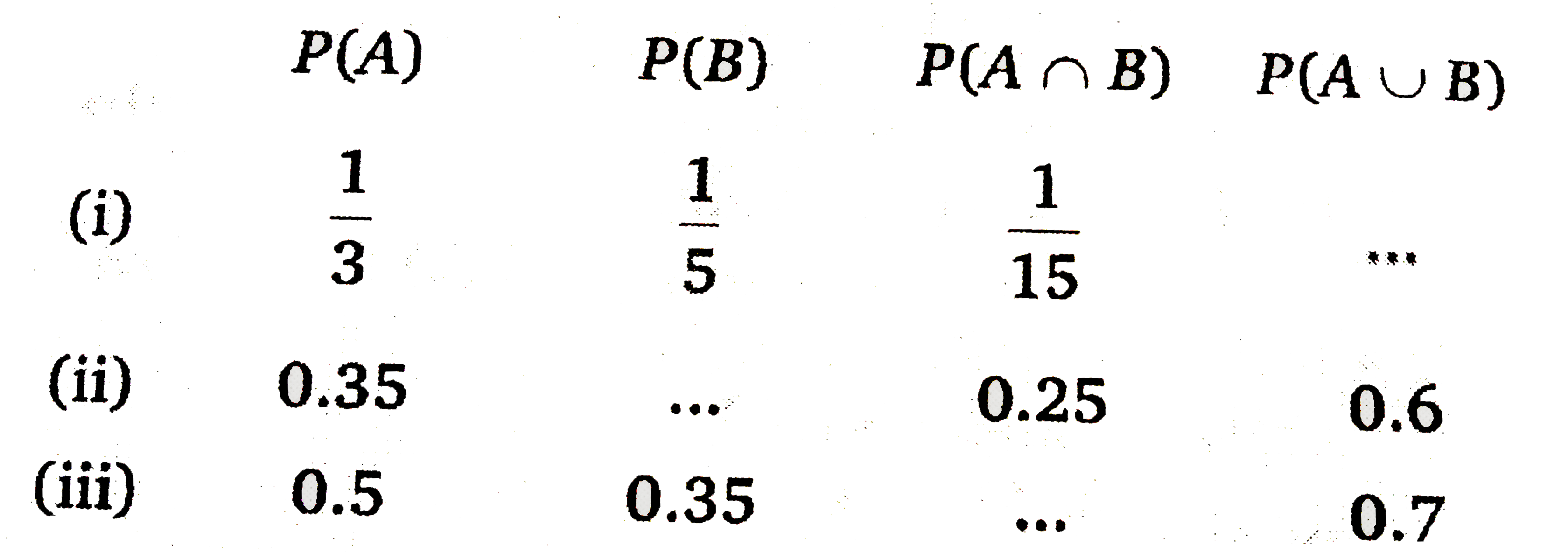 View Solution
View SolutionFill in the blanks:
12=20View SolutionFill in the blanks to make each of the following a true statement :
(i) A∪A=……..
(ii) ϕ∩A=……..
(iii) A∩A =…...
(iv) U∩A=………View SolutionFill in the blanks to make each of the following a true statement (i) 458+639=639+
View SolutionFill in the blanks in each of the following so that the resulting statement becomes true. The value of ∫dx√1−√x= ______.
View SolutionFill in the blanks in each of the following so that the resulting statement becomes true. The number of terms in the expansion of (3a+2b+c)12is ____
View Solution

