Differentiate between the following (with examples)
State function and path function.
Step-by-Step Solution
1. Definition of State Function: A state function is a property whose value depends only on the state of the system, not on how that state was reached. It is determined by the initial and final conditions of the system.
- Example: Internal energy (U), enthalpy (H), and entropy (S) are common examples of state functions. The change in internal energy (ΔU), change in enthalpy (ΔH), and change in entropy (ΔS) are all state functions.
2. Characteristics of State Functions:
- They are independent of the path taken to reach the final state.
- The change in the state function can be calculated by knowing only the initial and final states.
3. Definition of Path Function: A path function is a property whose value depends on the specific path taken to reach from one state to another. It is not solely determined by the initial and final states.
- Example: Heat (q) and work (w) are examples of path functions. The amount of heat added to or work done by the system depends on the specific process or path taken.
4. Characteristics of Path Functions:
- They are dependent on the path taken between the initial and final states.
- The values can vary even if the initial and final states are the same, depending on the process.
5. Comparison:
- State functions depend only on the state of the system (initial and final), while path functions depend on the route taken to get from one state to another.
- State functions are represented by symbols like ΔU, ΔH, and ΔS, while path functions are represented by q and w.
Summary Table
| Feature | State Function | Path Function |
|---------------------|----------------------------------------|-------------------------------------|
| Definition | Depends only on initial and final state| Depends on the path taken |
| Examples | Internal energy (ΔU), Enthalpy (ΔH) | Heat (q), Work (w) |
| Path Independence | Yes | No |
- They are independent of the path taken to reach the final state.
- The change in the state function can be calculated by knowing only the initial and final states.
- They are dependent on the path taken between the initial and final states.
- The values can vary even if the initial and final states are the same, depending on the process.
- State functions depend only on the state of the system (initial and final), while path functions depend on the route taken to get from one state to another.
- State functions are represented by symbols like ΔU, ΔH, and ΔS, while path functions are represented by q and w.
|---------------------|----------------------------------------|-------------------------------------|
| Definition | Depends only on initial and final state| Depends on the path taken |
| Examples | Internal energy (ΔU), Enthalpy (ΔH) | Heat (q), Work (w) |
| Path Independence | Yes | No |
Topper's Solved these Questions
- CHEMICAL THERMODYNAMICSBook:CBSE COMPLEMENTARY MATERIALChapter:CHEMICAL THERMODYNAMICSExercise:5-Marks Questions
Explore 8 Videos
- CHEMICAL THERMODYNAMICSBook:CBSE COMPLEMENTARY MATERIALChapter:CHEMICAL THERMODYNAMICSExercise:Hots Questions
Explore 3 Videos
- CHEMICAL THERMODYNAMICSBook:CBSE COMPLEMENTARY MATERIALChapter:CHEMICAL THERMODYNAMICSExercise:2-Marks Questions
Explore 19 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTURE Book:CBSE COMPLEMENTARY MATERIALChapter:CHEMICAL BONDING AND MOLECULAR STRUCTURE Exercise:UNIT TEST
Explore 13 Videos
- CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES Book:CBSE COMPLEMENTARY MATERIALChapter:CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIES Exercise:5 - MARKS QUESTIONS
Explore 6 Videos
Similar Questions
Differentiate between the following acids :
(a)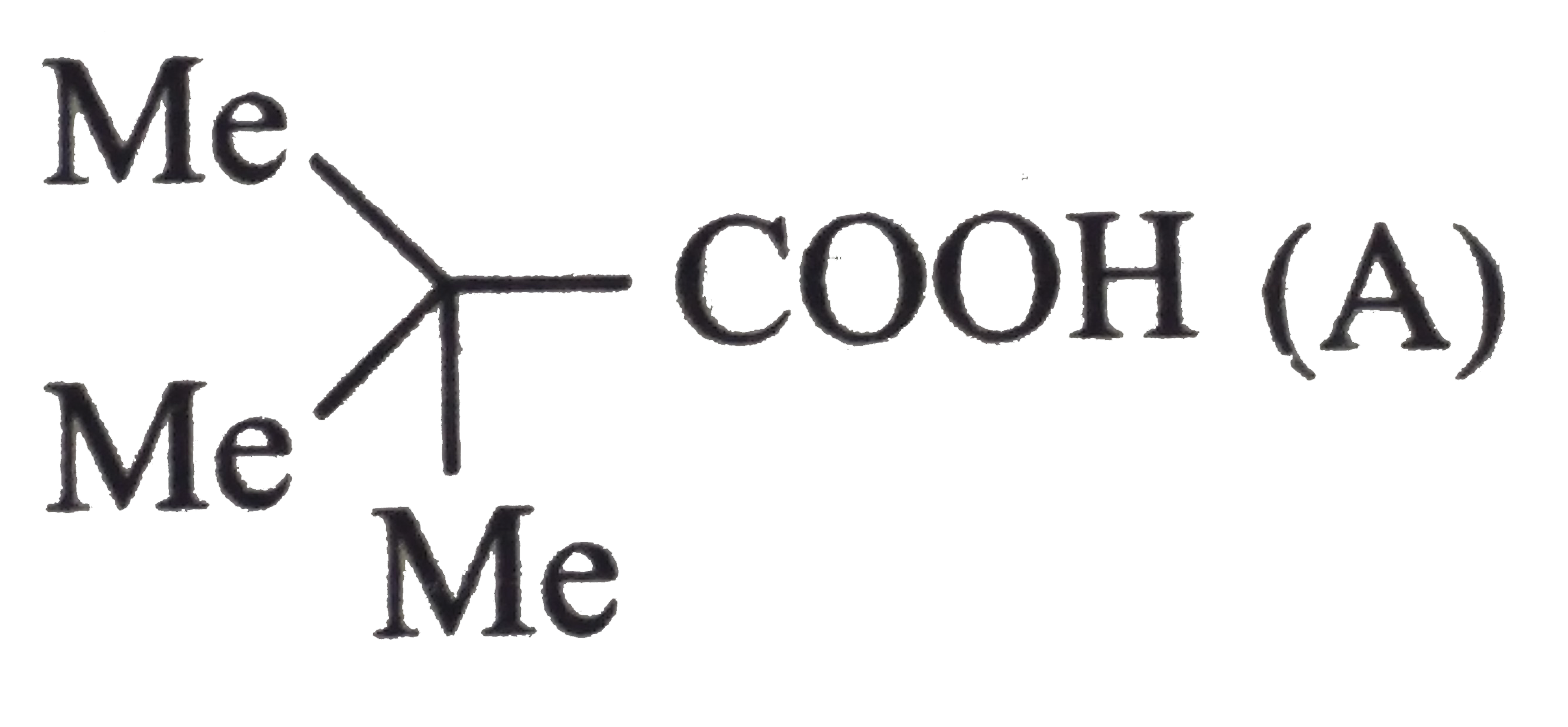
(b)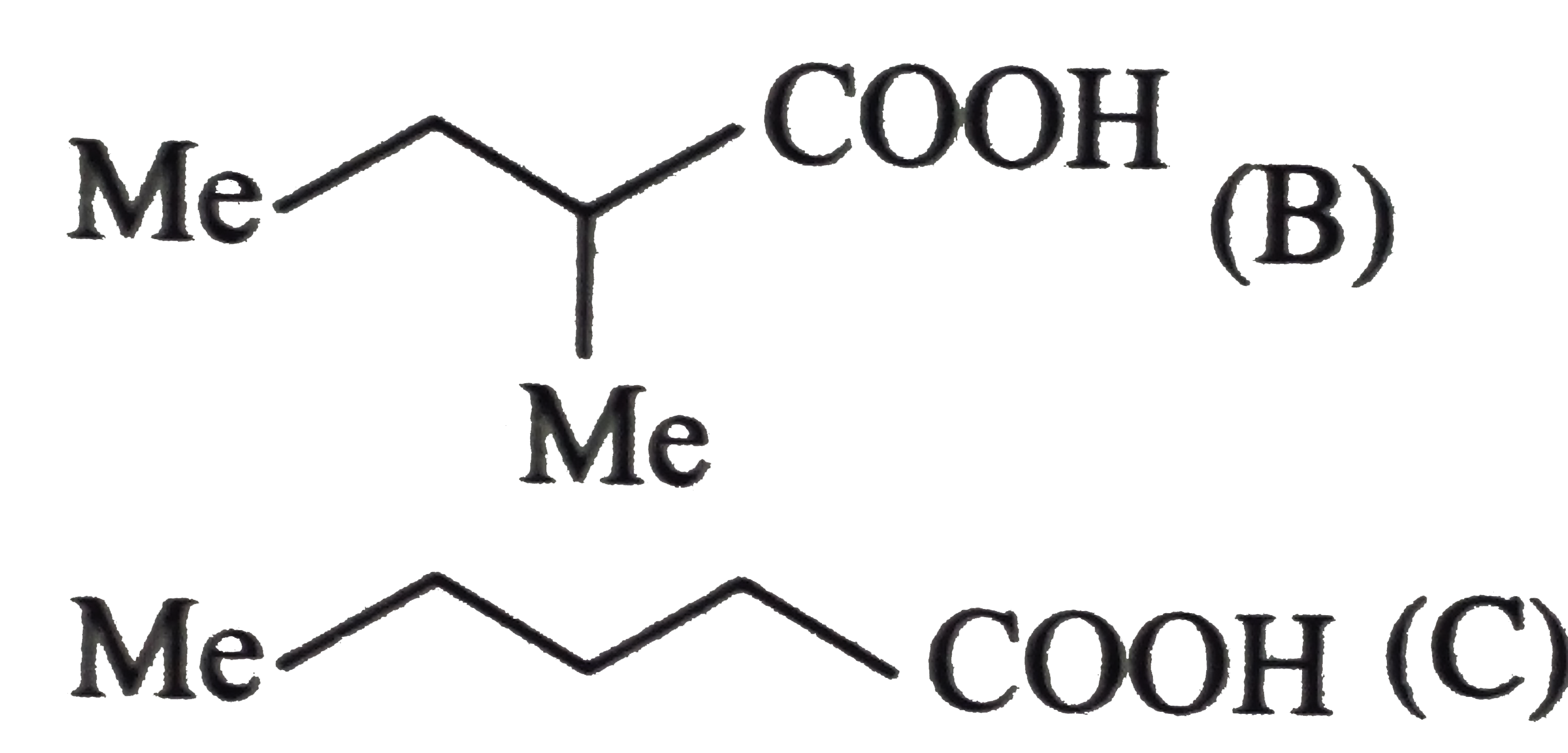
View SolutionDifferentiate between the following.
Flora and fauna
View SolutionDifferentiate between the following.
Prothalius and protonema
View SolutionDifferentiate between the following
Tendons and ligaments.
View SolutionDifferentiate between the following.
Apogamy and apospory
View SolutionDifferentiate between the following
(a) Exocrine and endocrine gland.
View SolutionDifferentiate between the following with suitable examples :
(i) mineral and ore
(ii) corrosion and rancidity
(iii) malleability and ductility
View SolutionDifferentiate between the following.
Zoo and wildlife sanctuary
View SolutionDifferentiate between the following :
Amylose and Amylopectin
View SolutionDifferentiate between the following.
Gametophyte and sporophyte
View Solution