The dimensions of intensity of wave are
A
[L°MT–3]
B
[L1M2T–2]
C
[L2MT–2]
D
[L2M2T–3]
Video Solution
Text Solution
The correct Answer is:A
Intensity = energyarea×time
=[(McancelL^(2)T^(-2))/(cancelL^(2)T)]=[MT^(-3)]=[ML^(@)T^(-3)]
|
Topper's Solved these Questions
- DIMENSIONS AND MEASUREMENT BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - DIMENSIONS AND MEASUREMENT EXERCISE - NUMERICALS
20 Videos
- ANNUAL EXAM.-2018-19BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ANNUAL EXAM.-2018-19EXERCISE - Questions
34 Videos
- GRAVITATIONBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - GRAVITATIONEXERCISE - MULTIPLE CHOICE QUESTIONS
19 Videos
Similar Questions
Knowledge Check
The dimensions of intensity of wave are
A[L°MT–3]B[L1M2T–2]C[L2MT–2]D[L2M2T–3]The unit of intensity of a wave is Wm2? What are dimensions of intensity of wave?
A[MT−3]B[AML0T−2]C[M0L−1T−2]DNone of theseThe dimensions of magnetic intensity are
A[LI]B[L2I]C[L−1I]D[L−2I]
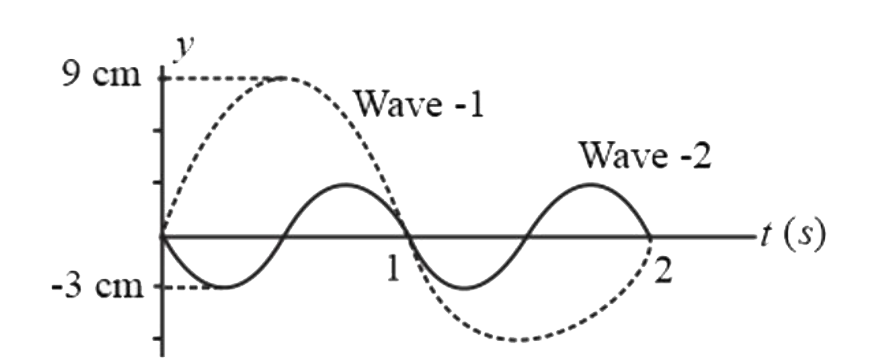
Displacement - time graphs for two waves, wave - 1 and wave - 2 are shown here. The ratio of the intensity of wave-1 to that of wave - 2 is
View SolutionTwo coherent sources produce waves of different intensities which interfere. A fter interference, the ratio of the maximum intensity to the minimum intensity is 16. The intensity of the waves are in the ratio :
View SolutionTwo coherent sources produce waves of different intensities which interfere. A fter interference, the ratio of the maximum intensity to the minimum intensity is 16. The intensity of the waves are in the ratio :
View SolutionThe dimensions of electric intensity are
View SolutionThe ratio of intensity of wave and energy density gives
View Solution

_E01_106_Q01.png)