If the length of direct common tangent and transverse common tangent of two circles with integral radii are 3 units and 1 unit respectively, then the reciprocal of the square of the distance between the centres of the circles is equal to
The correct Answer is:0.1
To solve the problem, we need to find the reciprocal of the square of the distance between the centers of two circles given the lengths of their direct and transverse common tangents.
1. Understanding the Problem:
We have two circles with radii R1 and R2. The lengths of the direct common tangent (DCT) and the transverse common tangent (TCT) are given as 3 units and 1 unit respectively.
2. Using the Formulas:
The formulas for the lengths of the direct common tangent (DCT) and the transverse common tangent (TCT) between two circles are:
- For DCT:
LDCT=√d2−(R1+R2)2
- For TCT:
LTCT=√d2−(R1−R2)2
where d is the distance between the centers of the circles.
3. Setting Up the Equations:
Given:
- LDCT=3
- LTCT=1
We can set up the equations:
√d2−(R1+R2)2=3(1)
√d2−(R1−R2)2=1(2)
4. Squaring Both Equations:
Squaring equation (1):
d2−(R1+R2)2=9⇒d2=9+(R1+R2)2(3)
Squaring equation (2):
d2−(R1−R2)2=1⇒d2=1+(R1−R2)2(4)
5. Equating the Two Expressions for d2:
From equations (3) and (4):
9+(R1+R2)2=1+(R1−R2)2
6. Simplifying the Equation:
Rearranging gives:
(R1+R2)2−(R1−R2)2=1−9
(R1+R2−(R1−R2))(R1+R2+(R1−R2))=−8
(2R2)(2R1)=−8⇒4R1R2=−8⇒R1R2=2
7. Finding Possible Values for R1 and R2:
Since R1 and R2 are integral radii, the possible pairs (R1,R2) are (2,1) or (1,2).
8. Substituting Back to Find d2:
Using R1=2 and R2=1:
d2=9+(2+1)2=9+9=18
9. Finding the Reciprocal of the Square of the Distance:
The reciprocal of the square of the distance between the centers is:
1d2=118
Final Answer:
The reciprocal of the square of the distance between the centers of the circles is 118.
We have two circles with radii R1 and R2. The lengths of the direct common tangent (DCT) and the transverse common tangent (TCT) are given as 3 units and 1 unit respectively.
The formulas for the lengths of the direct common tangent (DCT) and the transverse common tangent (TCT) between two circles are:
- For DCT:
LDCT=√d2−(R1+R2)2
- For TCT:
LTCT=√d2−(R1−R2)2
where d is the distance between the centers of the circles.
Given:
- LDCT=3
- LTCT=1
√d2−(R1+R2)2=3(1)
√d2−(R1−R2)2=1(2)
Squaring equation (1):
d2−(R1+R2)2=9⇒d2=9+(R1+R2)2(3)
d2−(R1−R2)2=1⇒d2=1+(R1−R2)2(4)
From equations (3) and (4):
9+(R1+R2)2=1+(R1−R2)2
Rearranging gives:
(R1+R2)2−(R1−R2)2=1−9
(R1+R2−(R1−R2))(R1+R2+(R1−R2))=−8
(2R2)(2R1)=−8⇒4R1R2=−8⇒R1R2=2
Since R1 and R2 are integral radii, the possible pairs (R1,R2) are (2,1) or (1,2).
Using R1=2 and R2=1:
d2=9+(2+1)2=9+9=18
The reciprocal of the square of the distance between the centers is:
1d2=118
The reciprocal of the square of the distance between the centers of the circles is 118.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The length of the direct common tangent of two circles of radii 2 cm and 8 cm is 8 cm. Then the distance between the centres of the circles is
A36 cmB6 cmC64 cmD8 cm - Question 2 - Select One
AC is transverse common tangent to two circles with centres P and Q and radii 6 cm and 3 cm at the point A and C respectively. If AC cuts PQ at the point B and AB= 8cm then the length of PQ is:
A13 cmB12 cmC10 cmD15 cm - Question 3 - Select One
The length of the direct common tangent of two circles of radii 2 cm and 8 cm is 8 cm. Then the distance between the centres of the circles is
A8 cmB10 cmC6 cmD12 cm
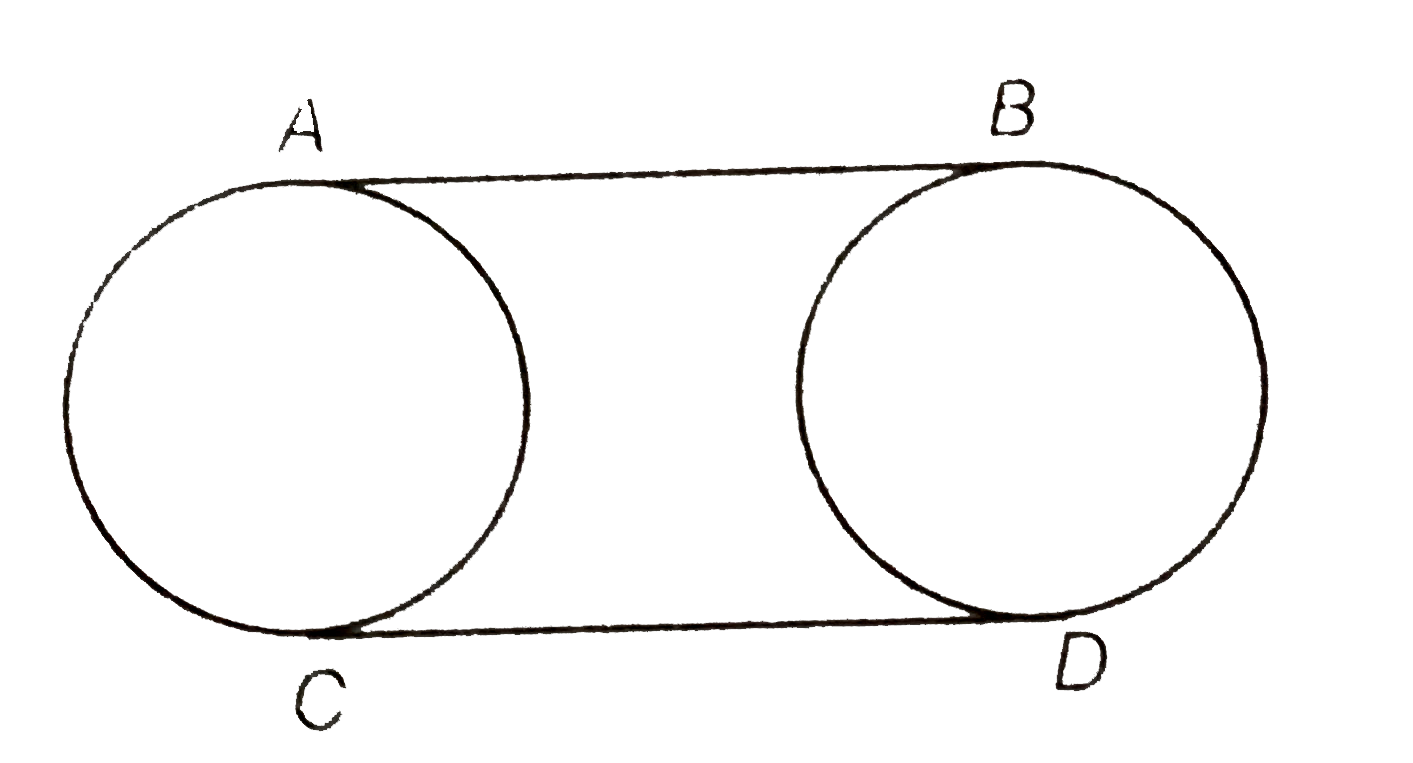
In figure, AB and CD are common tangents to two circles of equal radii. Prove that AB=CD.
 View Solution
View SolutionThe point of intersection of direct common tangents and transverse common tangents to two circles divide the line segmenta joining the two centres externally and internally respectively in the ratio of their radii.
View SolutionTwo circles of radii 4cm and 14cm have a common external tangent of length 24cm .Find the distance between the centres of these circles (in cm ).
View SolutionCommon Tangents of Two Circles - Direct and Transverse Common Tangent
View SolutionTwo circles of radius 4 units and 3 units are at some distance such that the length of the transverse common tangent and the length of their direct common tangent are in the ratio 1 : 2. What is the distance between the centres of those circles.
View Solution

