The time period of a spring - mass system is T. If this spring is cut into two parts, whose lengths are in the ratio 1:2, and the same mass is attached to the longer part, the new time period will be
√13T
√23T
√3T2
√3T
The correct Answer is:B
To solve the problem, we need to determine the new time period of a spring-mass system after the spring is cut into two parts in the ratio of 1:2, and the same mass is attached to the longer part.
1. Understand the Time Period of a Spring-Mass System:
The time period T of a spring-mass system is given by the formula:
T=2π√mk
where m is the mass attached to the spring and k is the spring constant.
2. Cutting the Spring:
We are given that the spring is cut into two parts in the ratio 1:2. Let the total length of the spring be L. Then, the lengths of the two parts can be defined as:
- L1=L3 (shorter part)
- L2=2L3 (longer part)
3. Finding the Spring Constant:
The spring constant k is inversely proportional to the length of the spring. If the original spring constant is k, then for the shorter part k1 and the longer part k2, we have:
k1L1=k2L2
Rearranging gives:
k2=k1L1L2
Since L1=L3 and L2=2L3, substituting these into the equation gives:
k2=k⋅L32L3=k2
4. Calculating the New Time Period:
Now, we attach the same mass m to the longer part of the spring, which has the spring constant k2=32k. The new time period T′ is given by:
T′=2π√mk2=2π√m32k=2π√2m3k
5. Relating New Time Period to Original Time Period:
We can relate this new time period to the original time period T:
T′=√23T
Final Answer:
The new time period T′ of the spring-mass system when the mass is attached to the longer part of the spring is:
T′=√23T
The time period T of a spring-mass system is given by the formula:
T=2π√mk
where m is the mass attached to the spring and k is the spring constant.
We are given that the spring is cut into two parts in the ratio 1:2. Let the total length of the spring be L. Then, the lengths of the two parts can be defined as:
- L1=L3 (shorter part)
- L2=2L3 (longer part)
The spring constant k is inversely proportional to the length of the spring. If the original spring constant is k, then for the shorter part k1 and the longer part k2, we have:
k1L1=k2L2
Rearranging gives:
k2=k1L1L2
Since L1=L3 and L2=2L3, substituting these into the equation gives:
k2=k⋅L32L3=k2
Now, we attach the same mass m to the longer part of the spring, which has the spring constant k2=32k. The new time period T′ is given by:
T′=2π√mk2=2π√m32k=2π√2m3k
We can relate this new time period to the original time period T:
T′=√23T
The new time period T′ of the spring-mass system when the mass is attached to the longer part of the spring is:
T′=√23T
Topper's Solved these Questions
Similar Questions
Knowledge Check
Time period of a spring mass system is T.If this spring is cut into two parts whose lengths are in ratio 1:3 and the same mass is attached to the longer part, the new time period will be
A√32TBT√3C√3T2D√3TTime period of a spring mass system can be changed by
Achanging the massBacclerating the point of suspension of the blockCcutting the spring (i.e.changing the length of the spring)Dimmersing the mass in a liquidA spring of force constant k is cut into two pieces whose lengths are in the ratio 1:2. The force constant of the longer piece?
Ak/2B3k/2C2kD3k
Time period of a block with a spring is T0. Now ,the spring is cut in two parts in the ratio 2:3. Now find the time period of same block with the smaller part of the spring.
View SolutionThe time period of a mass suspended from a spring is T. If the spring is cut into four equal parts and the same mass is suspended from one of the parts, then the new time period will be:
View SolutionA mass m performs oscillations of period T when hanged by spring of force constant K . If spring is cut in two parts and arranged in parallel and same mass is oscillated by them, then the new time period will be
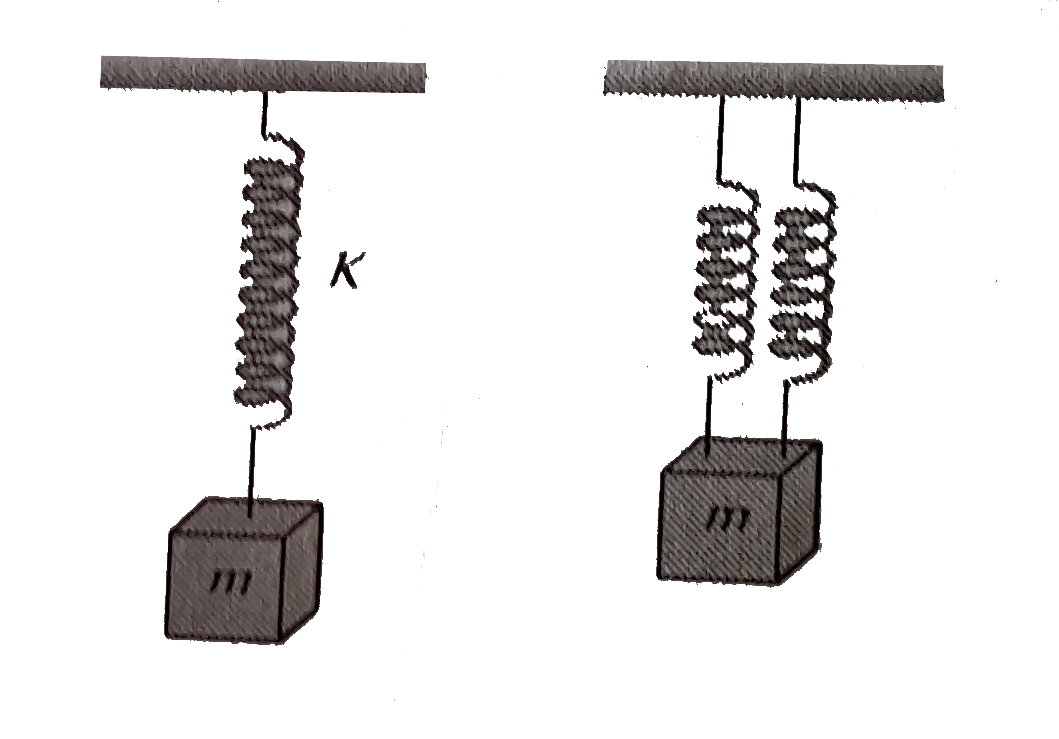
View SolutionThe time period of a mass suspended from a spring is T if the spring is cut in to equal part and the same mass is suspended from one of the pert then the time period will be
View SolutionA uniform spring has certain mass suspended from it and its period for vertical oscillation is T1. The spring is now cut into two parts having the lengths in the ratio of 1:2 and same mass is suspended with those two spring as shown in figure. Now time period is T2. The ratio T1/T2 is

View Solution
