Suppose the earth was covered by an ocean of uniform depth h,(h<<R) Let σ be density of ocean and p be mean density of earth. Let Δg be the approximate difference of value of net acceleration duet to gravity between the bottom of the ocean and top (Δg=g+(top)−gbottom). Choose the correct option.
Δg=43πGh[2ρ−3σ]
Δg=43Gπh[3σ−2ρ]
Δg=43Gπh[2σ−3ρ]
Δg=43Gπh[3ρ−3σ]
The correct Answer is:B
Step by step video, text & image solution for Suppose the earth was covered by an ocean of uniform depth h,(hltltR) Let sigma be density of ocean and p be mean density of earth. Let Deltag be the approximate difference of value of net acceleration duet to gravity between the bottom of the ocean and top (Deltag=g+("top")-g_("bottom")). Choose the correct option. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
At a depth h1=R2 from the surface of the earth acceleration due to gravity is g1. It’s value changes by Δg1 when one moves down further by 1 km. At a height h2 above the urface of the earth acceleration due to gravity is g9. It’s value changes by Δg2when one moves up further by 1 km. If Δg1=Δg2 find h2. Assume the earth to be a uniform phere of radius R.
View SolutionA hypothetical planet has an ocean of water which is 50km deep. The top 5km is frozen as ice (i.e., 45km is water). Radius and average density of the planet are both half the respective values for the earth. There is no atmospehre. Obtain an estimate of the pressure at the bottom of the ocean.
View Solution
Knowledge Check
- Question 1 - Select One
Suppose the earth was covered by an oceam of uniform depth h,(h<<R) Let σ be densityof ocean and p be mean density of earth. Let Δg be the approxemate difference of value of net acceleration duet to gravity between the bottom of the ocean and top (Δg=g+(top)−gbottom). Choose the correct option.
AΔg=43πGh[2p−3σ]BΔg=43Gπh[3σ−2p]CΔg=43Gπh[2σ−3σ]DΔg=43Gπh[3p−3σ] - Question 2 - Select One
Suppose the earth was covered by an ocean of uniform depth h,(h<<R) Let σ be density of ocean and p be mean density of earth. Let Δg be the approximate difference of value of net acceleration duet to gravity between the bottom of the ocean and top (Δg=g+(top)−gbottom). Choose the correct option.
AΔg=43πGh[2ρ−3σ]BΔg=43Gπh[3σ−2ρ]CΔg=43Gπh[2σ−3ρ]DΔg=43Gπh[3ρ−3σ] - Question 3 - Select One or More
One end of a string of length L is tied to the ceiling of a lift acceleration upwards with an acceleration 2g. The other end of the string is free. The linear mass density of the string varies linearly from 0→λ from bottom to top. Choose the correct option(s)
AThe velocity of the wave in the string will be 0.BThe acceleration (relative to string) of the wave on the string will be 3g/4 every where.CThe time taken by a pulse to reach from bottom to will be √8L/3g.DThe time taken by a pulse to reach from bottom to top will be √4L/3g.
Choose the correct alternative
(a)Acceleration due to gravity increase/decrease with increasing altitude.
(b) Acceleration due to gravity increase/decrease with increasing depth (assume the earth to be a sphere of uniform density).
(c ) Acceleration due to gravity is independer of mass of the earth/mass of the body.
(d) The formula −GMm(1r2−1r1) is more/less accurate than the formula mg(r2−r1) for the difference of potential energy between two points r2 and r1 distance away from the centre of earth.View SolutionA cylindrical furnace has height (H) and diameter (D) both 1 m. It is maintained at temperature 360 K. The air gets heated inside the furnace at constant pressure P_α, and its temperature becomes T = 360K The hot air with density ρ rises up a vertical chimney of diameter d = 0.1 m and height h = 9 m above the furnace and exits the chimney (see the figure). As a result, atmospheric air of density ρα=1.2kgm−3, pressure Pα, and temperature Tα = 300K enters the furnace. Assume air as an ideal gas, neglect the variations in ρ and T inside the chimney and the furnace. Also ignore the viscous effects.
[Given: The acceleration due to gravity g = 10ms−2 and π = 3.14 ]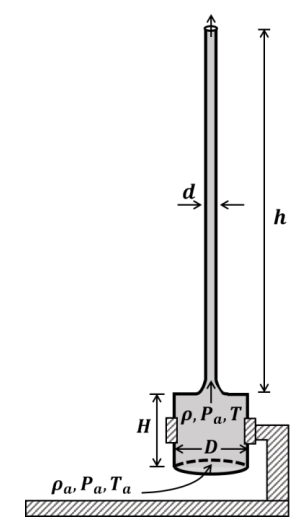
When the chimney is closed using a cap at the top, a pressure difference ΔP develops between the top and the bottom surfaces of the cap. If the changes in the temperature and density of the hot air, due to the stoppage of air flow, are negligible then the value of ΔP is _________Nm^(-2)View SolutionAn engineer is designing a spring to be placed at the bottom of an elevator shaft, if the elevator cable should break at a height h above the top of the spring, calculate the required value of the spring constant k, so that passsengers undergo an acceleration of no more than 10 g when brought to rest, Let M be the total mass of the elevator and passengers
View SolutionA beaker contains a fluid of density ρkg/m3, specific heat SJ/kg∘C and viscosity η. The beaker is filled up to height h. To estimate the rate of heat transfer per unit area (Q/A) by convection when beaker is put on a hot plate, a student proposes that it should depend on η,(SΔθh) and (1ρg) when Δθ(in∘C) is the difference in the temperature between the bottom and top of the fluid. In that situation the correct option for (Q/A) is
View SolutionLet gh and gd the acceleration due to gravity at height h above the earth s surface and at depth d below the earth surface respecitively if gh=gd then the releation between h and d is
View Solution

