The distance of the y - axis from from the center of the circle which lies in the first quadrant (see figure) and tangent to the lines y=12x,y=4 and the x-axis is
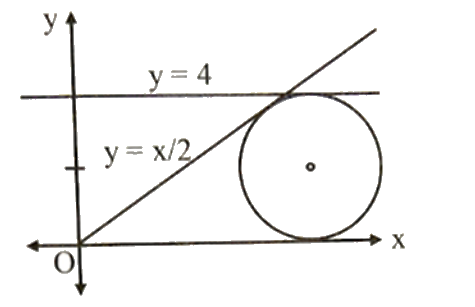
4+2√5 units
4−8√55 units
2+6√55 units
4−2√5 units
The correct Answer is:A
Step by step video & image solution for The distance of the y - axis from from the center of the circle which lies in the first quadrant (see figure) and tangent to the lines y=(1)/(2)x,y =4 and the x-axis is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
Area lying in the first quadrant and bounded by the circle x2+y2=4 the line x=√3y and x-axis , is
AπBπ/2Cπ/3DNone of theseThe area lying in the first quadrant and bounded by the circle x2+y2=4 and the line x=√3y is :
Aπ3Bπ2CπDπ5Area lying in the first quadrant and bounded by the circle x2+y2=4 the line x=√3y and x-axis , is
AπBπ/2Cπ/3Dπ/4
Find the area of the region in the first quadrant enclosed by the x-axis, the line y=x, and the circle x2+y2=32.
View SolutionFind the area of the region in the first quadrant enclosed by the x-axis, the line y=x and the circle x2+y2=32.
View SolutionThe diameter of the circle, whose centre lies on the line x+y=2 in the first quadrant and which touches both the lines x=3andy=2, is ..........
View SolutionFind the area of the region in the first quadrant enclosed by x−aξs, the line x=√3 y and the circle x2+y2=4.
View SolutionFind the area of region in the first quadrant enclosed the X-axis, the line y =x and the circlex2+y2=32.
View Solution
