A car is standing 200m behind a bus , which is also at rest . The two. Start moving at the same instant but with different forward accelerations. The bus has acceleration 2ms−2 and The car has acceleration 4ms−2 The car will catch up will the bus after time :
√120s
15 s
√110s
10√2s
The correct Answer is:D
To solve the problem of when the car will catch up with the bus, we can use the equations of motion. Let's break it down step by step.
Step 1: Understand the initial conditions
- The bus is at rest and has an acceleration of ab=2m/s2.
- The car is also at rest but has a higher acceleration of ac=4m/s2.
- The initial distance between the car and the bus is d=200m.
Step 2: Write the equations of motion
Since both vehicles start from rest, we can use the second equation of motion:
s=ut+12at2
where s is the distance traveled, u is the initial velocity (which is 0 for both), a is the acceleration, and t is the time.
For the bus:
sb=0⋅t+12⋅2⋅t2=t2
For the car:
sc=0⋅t+12⋅4⋅t2=2t2
Step 3: Set up the equation for when the car catches up
The car will catch up with the bus when the distance traveled by the car minus the distance traveled by the bus equals the initial distance between them:
sc−sb=200
Substituting the expressions for sc and sb:
2t2−t2=200
This simplifies to:
t2=200
Step 4: Solve for time t
Taking the square root of both sides:
t=√200=√100×2=10√2seconds
Final Answer
The car will catch up with the bus after t=10√2 seconds.
---
- The bus is at rest and has an acceleration of ab=2m/s2.
- The car is also at rest but has a higher acceleration of ac=4m/s2.
- The initial distance between the car and the bus is d=200m.
Since both vehicles start from rest, we can use the second equation of motion:
s=ut+12at2
where s is the distance traveled, u is the initial velocity (which is 0 for both), a is the acceleration, and t is the time.
sb=0⋅t+12⋅2⋅t2=t2
sc=0⋅t+12⋅4⋅t2=2t2
The car will catch up with the bus when the distance traveled by the car minus the distance traveled by the bus equals the initial distance between them:
sc−sb=200
Substituting the expressions for sc and sb:
2t2−t2=200
This simplifies to:
t2=200
Taking the square root of both sides:
t=√200=√100×2=10√2seconds
The car will catch up with the bus after t=10√2 seconds.
Topper's Solved these Questions
Similar Questions
Figure 2.E6 shows the speed-time graph of a bus.
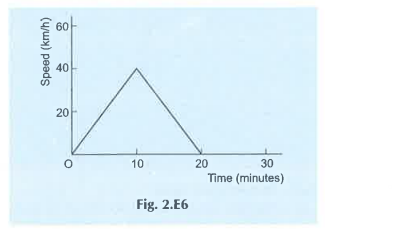
In which period is the bus accelerating ?
View SolutionA scooter starts from rest have an acceleration of 1ms−2 while a car 150 m behind it starts from rest with an acceleration of 2ms−2. After how much time the car catches up with the scooter ?
View Solution
Knowledge Check
A car is standing 800 m behind a bus, which is also at rest. The two start moving at the same instant but the different forward accelerations. The bus has acceleration 4m/s2 and the car has acceleration 8m/s2. The car will catch up with the bus after a time of:
A20sB10sC5sD15sA racing car has a uniform acceleration of 4m/s2. What distance will it cover in 10s after start ?
A200 mB500 mC900 mD400 mA car is standing 100 m behind a bus. The bus and the car both start moving simultaneously with acceleration of 4m/s2 and 2m/s2 respectively. Find the time after which the car will be able to catch the bus.
A10 sB20 sC15 sDnone
Two cars A and B are at rest at same point initially. If A starts with uniform velocity of 40 m/sec and B starts in the same direction with constant acceleration of4m/s2,then B will catch A after :
View SolutionA bus moving along a straight line at 20 m/s undergoes an acceleration of 4 m/s2. After 2 seconds, its speed will be :
View SolutionA man is 25 m behind a bus, when bus starts accelerating at 2ms−2 and man starts moving with constant velocity of 10ms−1. Time taken by him to board the bus is
View SolutionTwo cars A and B are at rest at the origin O. If A starts with a uniform velocity of20m/s and B starts in the same direction with a constant acceleration of 2m/s2, then the cars will meet after time
View SolutionA bus begins to move with an acceleration of 1ms−1 . A man who is 148m behind the bus starts running at 10ms−1 to catch the bus, the man will be able to catch the bus after .
View Solution
