If C the concentration of a weak electrolyte , α is the degree of ionization and Ka is the acid ionization constant , then the correct relationship between α , C and Ka is
a2=√KaC
a2=√CKa
α=√CKa
α=√KaC
The correct Answer is:D
To derive the relationship between the concentration (C), degree of ionization (α), and the acid ionization constant (K_a) for a weak electrolyte, we can follow these steps:
Step 1: Write the dissociation equation
For a weak acid (HA), the dissociation can be represented as:
HA⇌H++A−
Step 2: Define the concentration and degree of ionization
Let:
- C = initial concentration of the weak acid (HA)
- α = degree of ionization (the fraction of the acid that dissociates)
Step 3: Express the concentrations at equilibrium
At equilibrium, the concentrations can be expressed as:
- Concentration of HA = C(1−α)
- Concentration of H+ = Cα
- Concentration of A− = Cα
Step 4: Write the expression for the acid dissociation constant (K_a)
The acid dissociation constant Ka is given by:
Ka=(H+)(A−)(HA)
Substituting the equilibrium concentrations into this expression:
Ka=(Cα)(Cα)C(1−α)
Step 5: Simplify the equation
This simplifies to:
Ka=Cα21−α
Step 6: Make an approximation for weak acids
Since α is small for weak acids, we can approximate 1−α≈1. Thus, the equation simplifies to:
Ka≈Cα2
Step 7: Solve for α
Rearranging the equation gives us:
α2=KaC
Taking the square root of both sides:
α=√KaC
Conclusion
The correct relationship between the degree of ionization (α), concentration (C), and the acid ionization constant (K_a) is:
α=√KaC
For a weak acid (HA), the dissociation can be represented as:
HA⇌H++A−
Let:
- C = initial concentration of the weak acid (HA)
- α = degree of ionization (the fraction of the acid that dissociates)
At equilibrium, the concentrations can be expressed as:
- Concentration of HA = C(1−α)
- Concentration of H+ = Cα
- Concentration of A− = Cα
The acid dissociation constant Ka is given by:
Ka=(H+)(A−)(HA)
Ka=(Cα)(Cα)C(1−α)
This simplifies to:
Ka=Cα21−α
Since α is small for weak acids, we can approximate 1−α≈1. Thus, the equation simplifies to:
Ka≈Cα2
Rearranging the equation gives us:
α2=KaC
α=√KaC
The correct relationship between the degree of ionization (α), concentration (C), and the acid ionization constant (K_a) is:
α=√KaC
Topper's Solved these Questions
Similar Questions
Knowledge Check
For solution of weak electrolytic, the degree of ionization
AWill be reciprocal to the dilutionBWill be proportional to dilutionCWill be proportional to the square root of dilutionDWill be proportional to concentration of electrolyteIf α is the degree of ionization, C the concentration of a weak electrolyte and Ka the acid ionization constant , then the correct relationship between α and C is
Aα2=√KaCBα2=√CKaCα=√KaCDα=√CKaIf α is the degree of ionization, C the concentration of a weak electrolyte and Ka the acid ionization constant , then the correct relationship between α and C is
Aα2=√KaCBα2=√CKaCα=√KaCDα=√CKa
For solution of weak electrolytic, the degree of ionization
View SolutionFor a 'C'M concentarted solution of a weak electrolyte AxByα(degree of dissociation) is
View Solution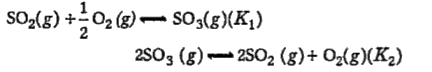
The correct relationship between equilibrium constantsK1 and K2 is
View SolutionIf the degree of ionization of water is 1.8×10−9 at 298K. Its ionization constant will be
View SolutionIf the concentration of the weak monoprotic acid HA is C mmol L−1 and its ionization constant is Ka, then
View Solution
