A particle of mass 3 kg , attached to a spring with force constant 48Nm−1execute simple harmonic motion on a frictionless horizontal surface. The time period of oscillation of the particle, is seconds , is
π4
π2
2π
8π
The correct Answer is:B
To find the time period of oscillation for a particle attached to a spring, we can use the formula for the time period T of a simple harmonic oscillator:
T=2π√mk
where:
- T is the time period,
- m is the mass of the particle,
- k is the spring constant.
Step 1: Identify the values given in the problem
- Mass of the particle, m=3kg
- Spring constant, k=48N/m
Step 2: Substitute the values into the formula
Now we will substitute the values of m and k into the formula:
T=2π√348
Step 3: Simplify the fraction inside the square root
First, simplify 348:
348=116
Step 4: Calculate the square root
Now, take the square root of 116:
√116=14
Step 5: Substitute back into the formula
Now substitute back into the formula for T:
T=2π⋅14
Step 6: Simplify the expression
Now simplify:
T=2π4=π2
Step 7: Final answer
Thus, the time period of oscillation of the particle is:
T=π2seconds
- T is the time period,
- m is the mass of the particle,
- k is the spring constant.
- Mass of the particle, m=3kg
- Spring constant, k=48N/m
Now we will substitute the values of m and k into the formula:
First, simplify 348:
Now, take the square root of 116:
Now substitute back into the formula for T:
Now simplify:
Thus, the time period of oscillation of the particle is:
Topper's Solved these Questions
Similar Questions
Knowledge Check
A 0.20kg object mass attached to a spring whose spring constant is 500N/m executes simple harmonic motion. If its maximum speed is 5.0m/s, the amplitude of its oscillation is
A0.0020mB0.10mC0.20mD25mThe velocity of a particle executing simple harmonic motion is
Aω2√A2+x2Bω√A2−x2Cω√A2+x2Dω2√A2−x2A particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is
 A2π5sB2√2π5sC√2π5sD4π5s
A2π5sB2√2π5sC√2π5sD4π5s
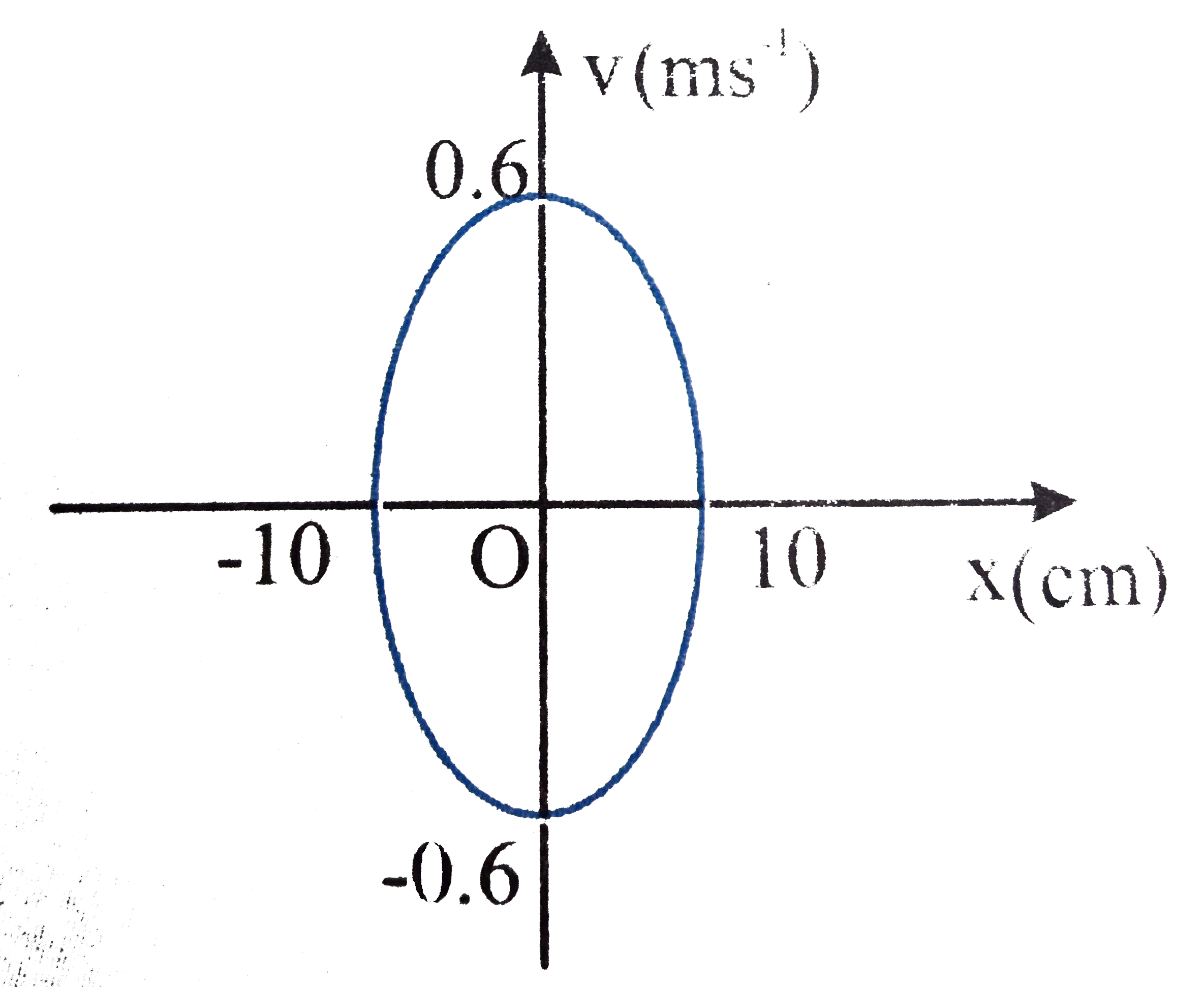
Figure shows the graph of velocity versus displacement of a partciel executing simple harmonic motion. Find the period of oscillation of the particle.
View SolutionFor a particle executing simple harmonic motion, the acceleration is proportional to
View SolutionA particle undergoes simple harmonic motion having time period T. The time taken in 3/8th oscillation is
View SolutionIf a particle is executing simple harmonic motion, then acceleration of particle
View SolutionA particle executes simple harmonic motion according to equation 4d2xdt2+320x=0. Its time period of oscillation is :-
View Solution

