A college offer 7 courses in the morning and 5 in the evening. Find the possible number of choices with the student. If he wants to study one course in the morning and one in the evening.
The correct Answer is:35
To solve the problem of finding the total number of choices a student has when selecting one course in the morning and one in the evening, we can follow these steps:
1. Identify the Number of Courses:
- The college offers 7 courses in the morning.
- The college offers 5 courses in the evening.
2. Choosing a Course in the Morning:
- The student can choose 1 course from the 7 morning courses. This can be represented mathematically as 7C1, which is simply 7.
3. Choosing a Course in the Evening:
- The student can choose 1 course from the 5 evening courses. This can be represented mathematically as 5C1, which is simply 5.
4. Calculating Total Choices:
- Since the student needs to choose one course from the morning and one from the evening, we multiply the number of choices:
Total Choices=7C1×5C1=7×5
5. Final Calculation:
- Performing the multiplication gives:
7×5=35
Thus, the total number of choices the student has is 35.
- The college offers 7 courses in the morning.
- The college offers 5 courses in the evening.
- The student can choose 1 course from the 7 morning courses. This can be represented mathematically as 7C1, which is simply 7.
- The student can choose 1 course from the 5 evening courses. This can be represented mathematically as 5C1, which is simply 5.
- Since the student needs to choose one course from the morning and one from the evening, we multiply the number of choices:
Total Choices=7C1×5C1=7×5
- Performing the multiplication gives:
7×5=35
Topper's Solved these Questions
- PERMUTATIONS & COMBINATIONSBOOK - FIITJEECHAPTER - PERMUTATIONS & COMBINATIONSEXERCISE - SOLVED PROBLEMS (OBJECTIVE)
24 Videos
- PERMUTATIONS & COMBINATIONSBOOK - FIITJEECHAPTER - PERMUTATIONS & COMBINATIONSEXERCISE - EXERCISE 3
3 Videos
- PARABOLABOOK - FIITJEECHAPTER - PARABOLAEXERCISE - NUMERICAL BASED
5 Videos
- PROBABILITYBOOK - FIITJEECHAPTER - PROBABILITYEXERCISE - Exercise 7
2 Videos
Similar Questions
A college offers 6 courses in the morning and 4 in the evening. Find the possible number of choices with the student if he wants to study one course in the morning and one in the evening.
View SolutionA college offers 7 courses in the morning and 5 in the evening. Find the number of ways a student can select exactly one course, either in the morning or in the evening.
View Solution
Knowledge Check
A college offers 10 courses in the morning and 7 in the evening. Find the number of ways a student can select exactly one course, either in the morning or in the evening.
A10B29C30D17A college offers 10 courses in the morning and 7 in the evening. Find the number of ways a student can select exactly one course, either in the morning or in the evening
A10B29C30D17Statement : More number of students passing SSC examination has resulted into frustration among children for not getting admissions into colleges of their choice.
Courses of Action: I. Government should permit the colleges to increase the number of seats. II. Children and their parents should be counselled for being flexible on the choice of college.Aif only I followsBif only II followsCif either I or II followsDif neither I nor II follows
A college offers 6 courses in the morning and 4 in the evening. Find the number of ways a student can select exactly one course, either in the morning or in the evening.
View SolutionNaresh walked 2 km 35 m in the morning and 1 km 7 m in the evening. How much distance did he walk in all?
View SolutionX jogs a total of 7.5 km in a day. He jogged 25/6 km in the morning. How much did he jog in the evening?
View SolutionA bus covers a distance of 250 km from Delhi to Jaipur towards West in 5 hours in the morning and returns to Delhi in the evening covering the same distance of 250 km in the same time of 5 hours. Find (a) average speed, and (b) average velocity, of the bus for the whole journey.
View SolutionThe following graph below shows the number of students (in thousands) who were admitted to three different courses in 5 years in a university. Study the graph and answer the question.
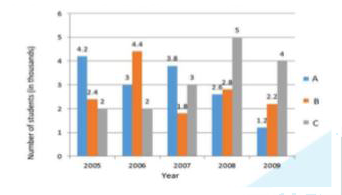
What is the ratio of the number of students who were admitted to course A in the year 2007 to that of students who were admitted to course C in the year 2009?
View Solution
