Find the equation of the circle whose centre is at (3,−1) and which cuts off a chord of length 6units on the line 2x−5y+18=0.
Video Solution
Text Solution
Given centre of the circle is (3,−1)
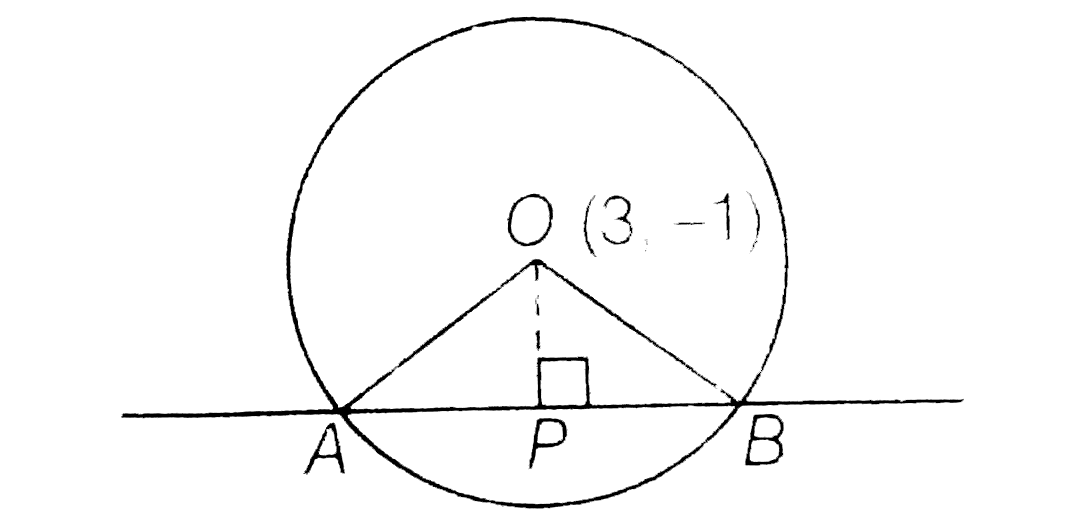
Now, OP=∣∣∣6+5+18√4+25∣∣∣=29√29=√29
In ΔOPB,OB2=OP2+PB2 [∵AB=6⇒PB=3]
⇒OB2=29+9⇒OB2=38
So, theradius of circle is √38
∴ Equation of the circle with radiusr=√38 and centre (3,−1) is
⇒(x−3)2+(y−1)2=38
⇒x2−6x+9+y2+2y+1=38
x2+y2−6x+2y=28
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NCERT EXEMPLARCHAPTER - CONIC SECTIONSEXERCISE - True/False8videos
CONIC SECTIONS
- BOOK - NCERT EXEMPLARCHAPTER - CONIC SECTIONSEXERCISE - Fillers6videos
CONIC SECTIONS
- BOOK - NCERT EXEMPLARCHAPTER - CONIC SECTIONSEXERCISE - Objective type13videos
CONIC SECTIONS
- BOOK - NCERT EXEMPLARCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - OBJECTIVE TYPE QUESTIONS16videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - NCERT EXEMPLARCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - Fillers16videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
Similar Questions
Knowledge Check
- Question 1 - Select One
Equation of circle centred at (3,-2) , which cuts off a chord
of length 6 from the line 4x−3y+2=0 , isA(x−3)2+(y+2)2=5B(x+3)2+(y−2)2=5Cx2+y2−6x+4y−12=0Dx2+y2−4x+6y+12=0 - Question 2 - Select One
Radius of the circle with centre (3,1) and cutting a chord of length 6 on the line 2x+5y+18=0 is
A6B7C√38D2√5
Find the equation of the circle , centred at (1,4) , which
cuts off of a chord of length 4 units on the line 3x+4y+1=0View SolutionFind the equation of the circle with center at (3,−1) and which cuts off an intercept of length 6 from the line 2x−5y+18=0
View SolutionFind the equation of a circle whose centre is (2,-1) and touches the line x-y-6=0.
View SolutionFind the equation of a circle whose centre is at (4,−2) and 3x−4y+5=0 is tangent to circle.
View SolutionFind the equation of a circle whose centre is at (4,−2) and 3x−4y+5=0 is tangent to circle.
View SolutionFind the equation of circle whose centre is the point (1,−3) and touches the line 2x−y−4=0
View Solution

