Find the equation of a circle with origin as centre and which circumscribes equilateral triangle whose median of length $3 a$

A

$x^{2} + y^{2} = 9 a^{2}$

B

$x^{2} + y^{2} = 16 a^{2}$

C

$x^{2} + y^{2} = 4 a^{2}$

D

$x^{2} + y^{2} = a^{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Given that, length of the median AD=3a
$∵$ Radius of the circle= $\frac{3}{2} \times$ Length of median
$= \frac{2}{3} \times 3 a = 2 a$

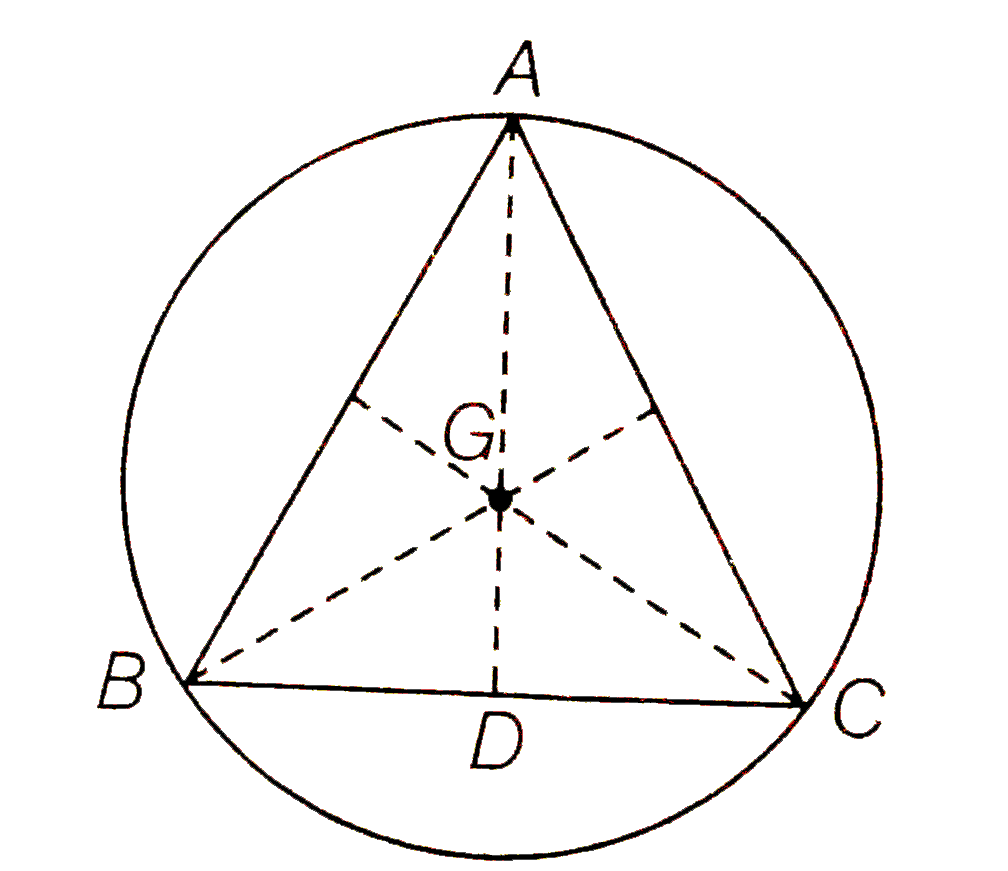
So, the equation of the circle is $x^{2} + y^{2} = 4 a^{2}$ .

|

Updated on:21/07/2023

Class 11 MATHS PARABOLA

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The equation of the circle with origin as centre passing the vertices of an equilateral triangle whose median is of length 3a is
A $x^{2} + y^{2} = 9 a^{2}$
B $x^{2} + y^{2} = 16 a^{2}$
C $x^{2} + y^{2} = a^{2}$
Dnone of the above
Question 2 - Select One
The equation of the circle with origin as centre passing the vertices of an equilateral triangle whose median is of length 3a is
A $x^{2} + y^{2} = 9 a^{2}$
B $x^{2} + y^{2} = 16 a^{2}$
C $x^{2} + y^{2} = a^{2}$
Dnone of the above
Question 3 - Select One
The equation of a circle with origin as centre and passing through the vertices of an equilateral triangle whose median is of length 3a, is
A $x^{2} + y^{2} = 9 a^{2}$
B $x^{2} + y^{2} = 16 a^{2}$
C $x^{2} + y^{2} = 4 a^{2}$
D $x^{2} + y^{2} = a^{2}$

NCERT EXEMPLAR-CONIC SECTIONS-Objective type

Home
Ask Doubt
Profile