Feasible region (shaded) for a LPP is shown in following figure. Maximise Z=5x+7y.
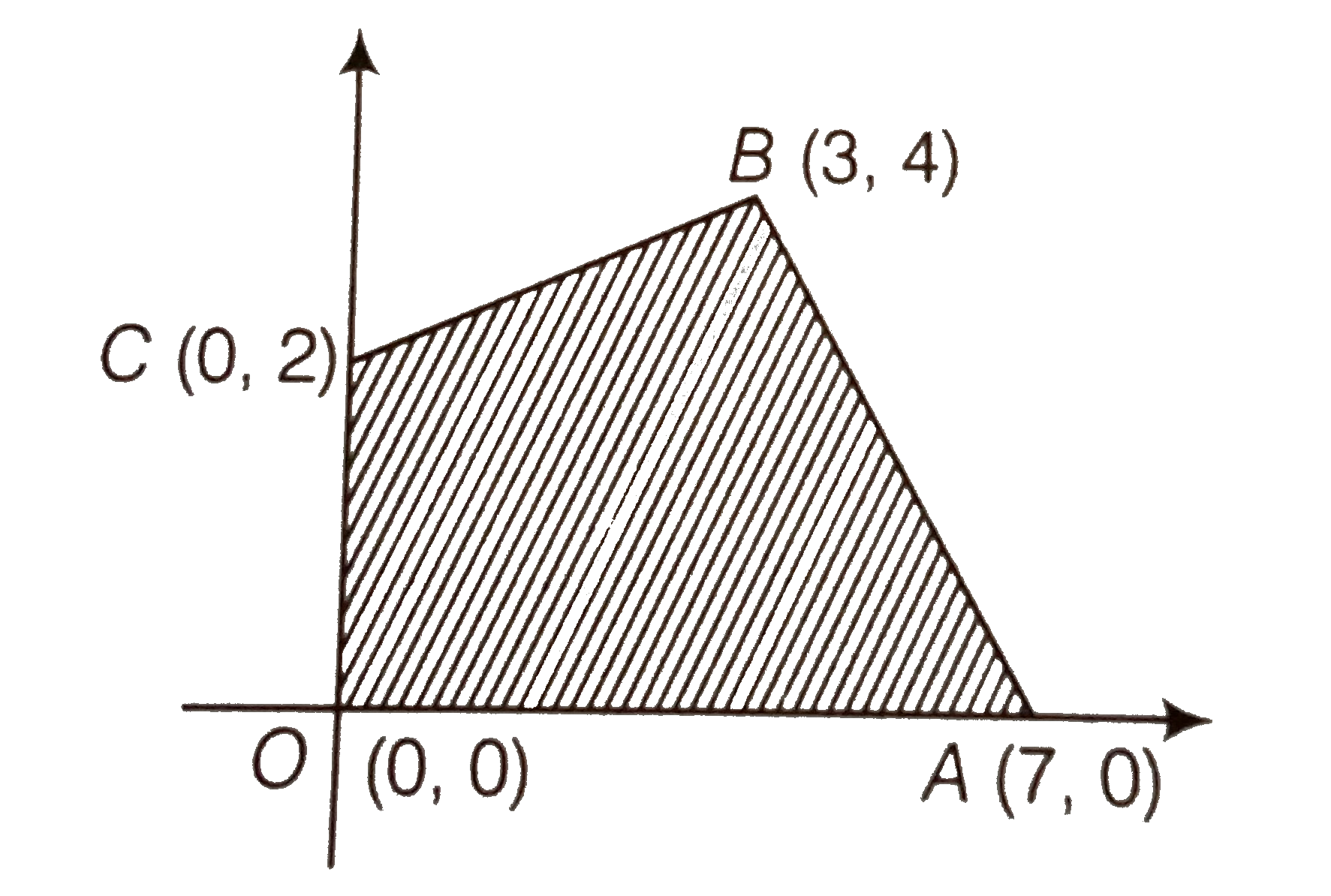
The correct Answer is:N/a
The shaded region is bounded and has corrdinates of corner points as (0,0), (7,0),(3,4) and (0,2). Also, Z=5x+7y.
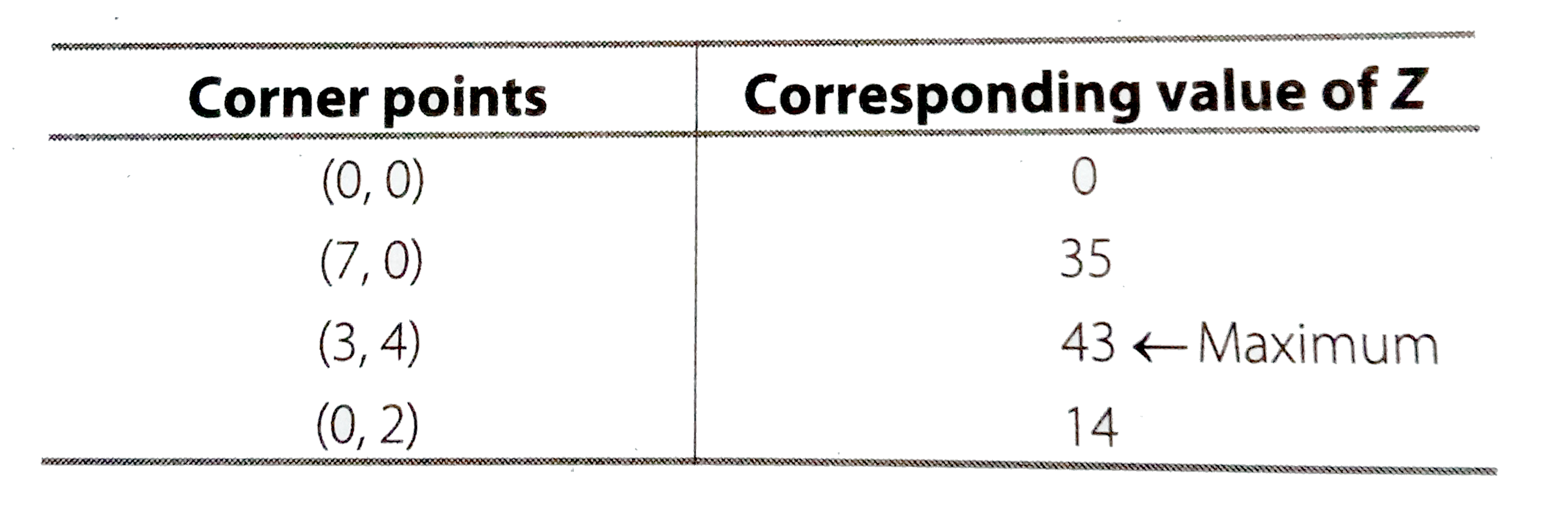
Hence, the maximum value of Z is 43 at (3,4).
Topper's Solved these Questions
Similar Questions
Determine the maximum value of Z=3x+4y, if the feasible reigon (shaded) for a LPP is shown in following figure
 View Solution
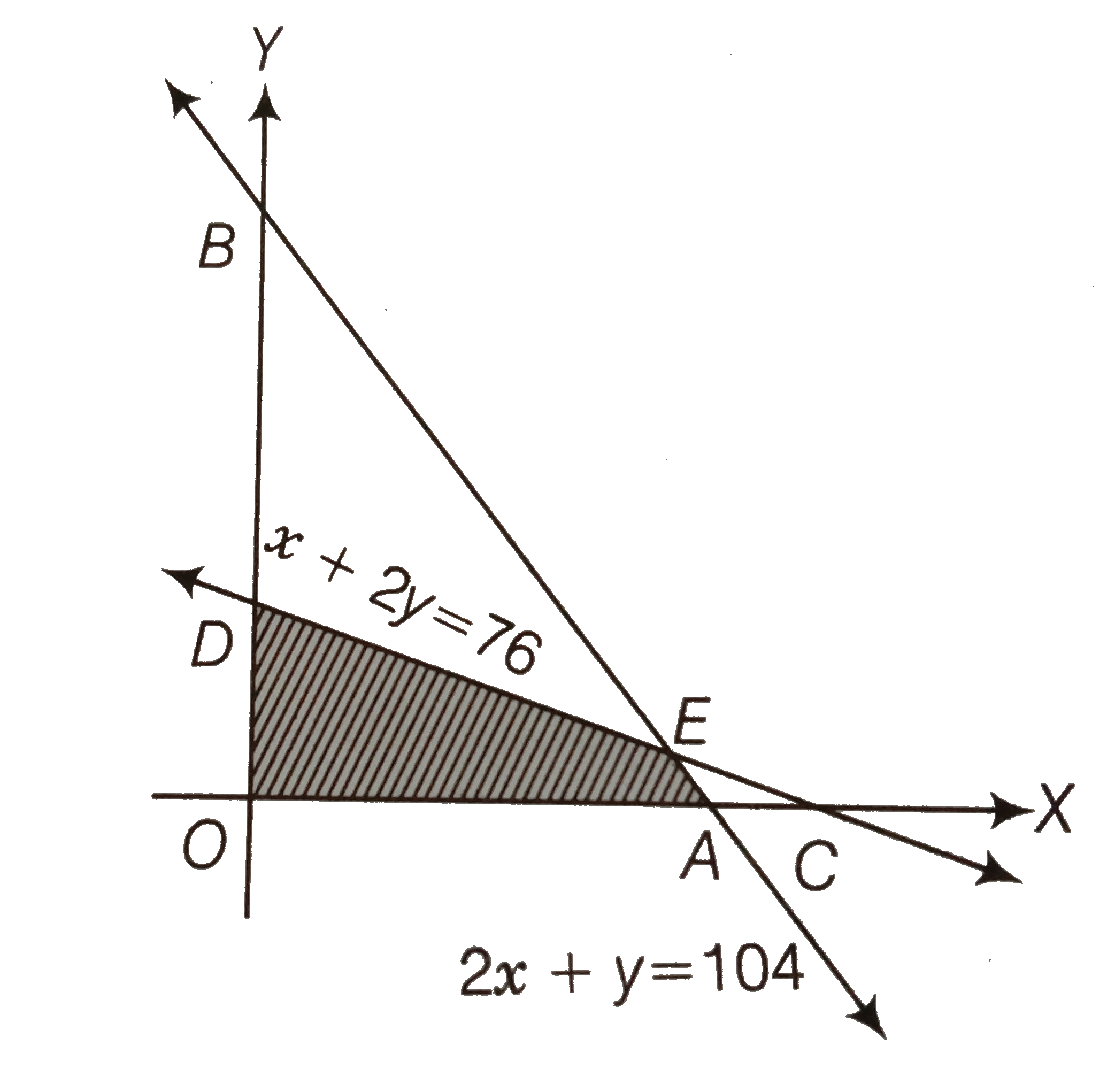
View SolutionThe feasible region for a LPP is shown in the following figure. Evaluate Z=4x+y at each of the corner points of the region. Find the minimum value of Z, if it exists
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The feasible region for an LPP is shown in the following figure. Let F=3x−4y be the objective function. Maximum value of F is
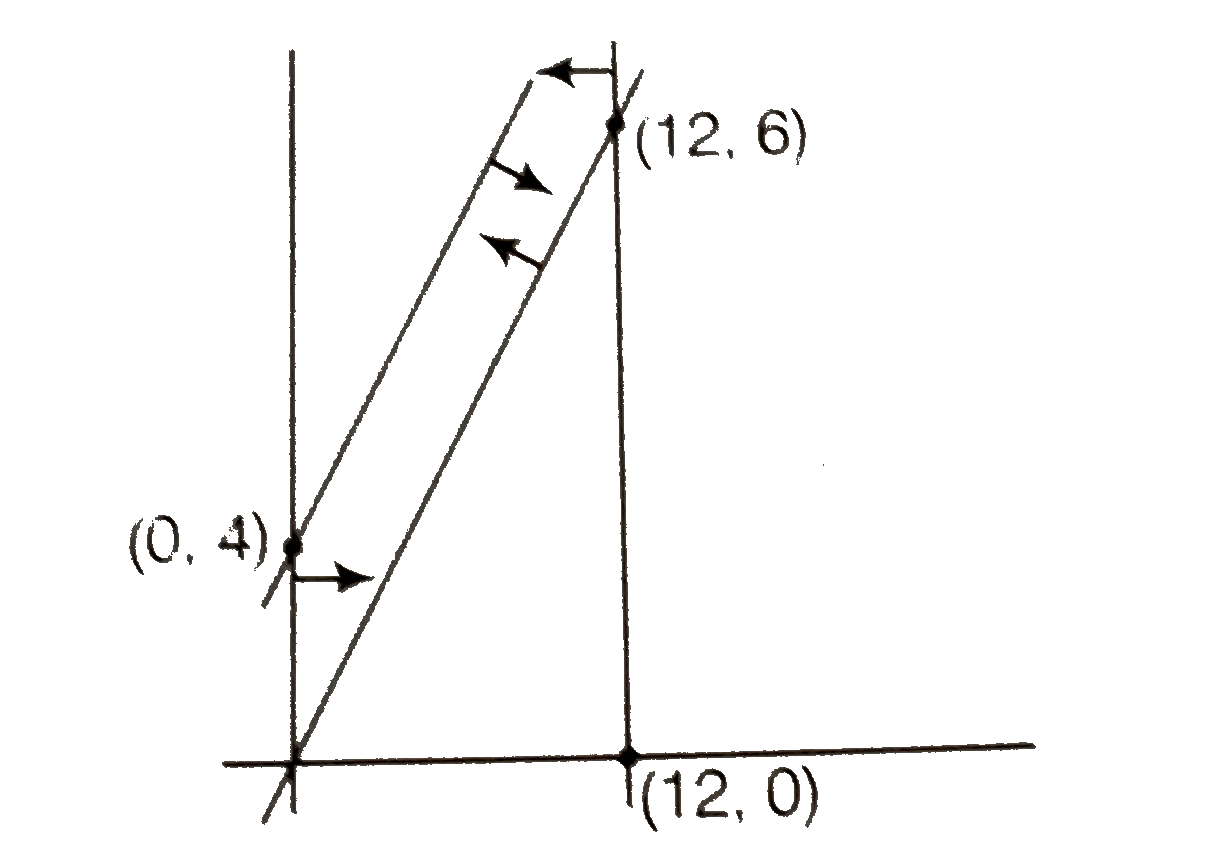 A0B8C12D−18
A0B8C12D−18 - Question 2 - Select One
The feasible region for an LPP is shown in following figure. Find the minimum value of Z=11x+7y.
 A22B23C24D21
A22B23C24D21 - Question 3 - Select One
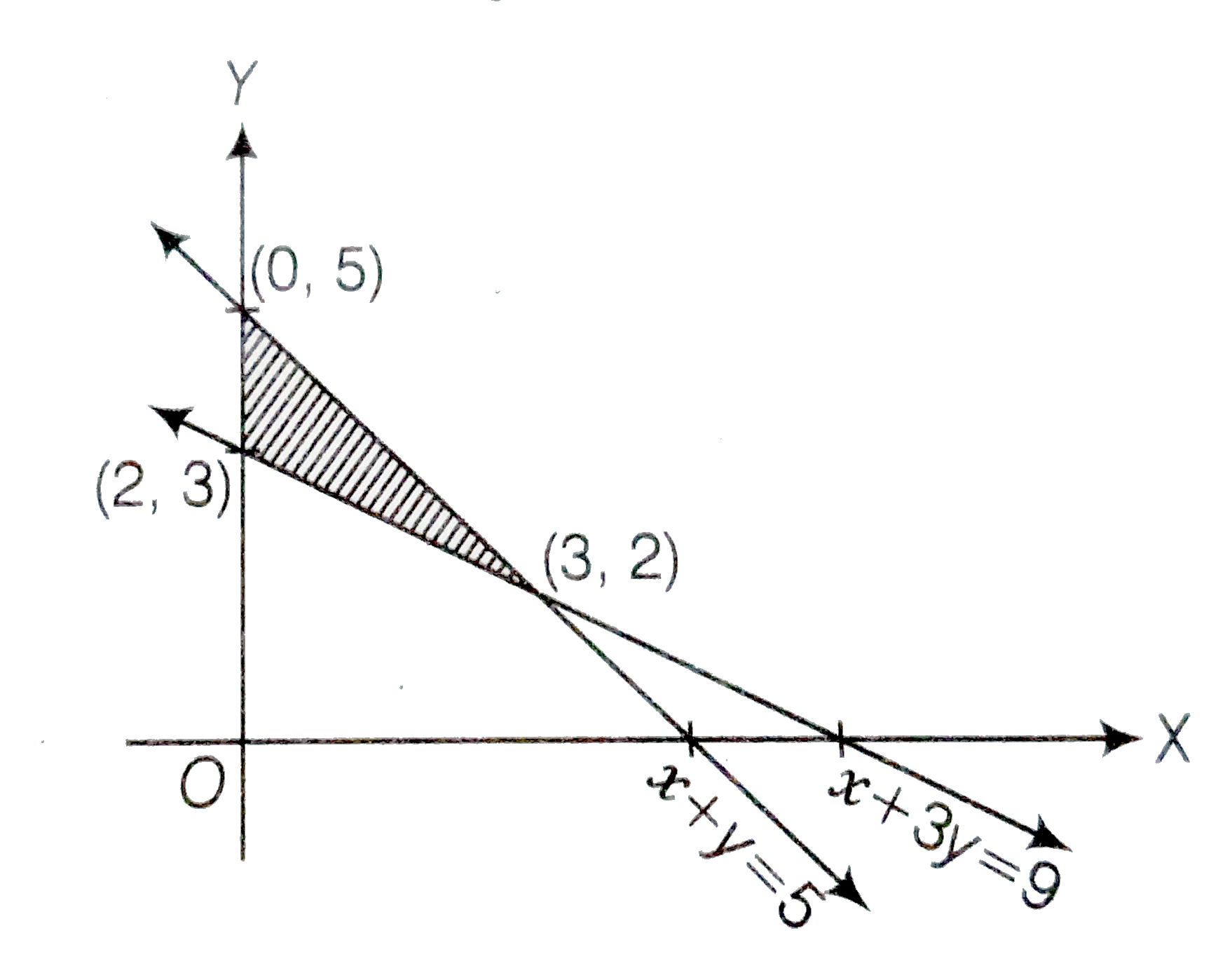
The feasible solution for a LPP is shown in following figure. Let Z=3x−4y be the objective function, Minimum of Z occurs at
 A(0,0)B(0,8)C(5,0)D(4,10)
A(0,0)B(0,8)C(5,0)D(4,10)
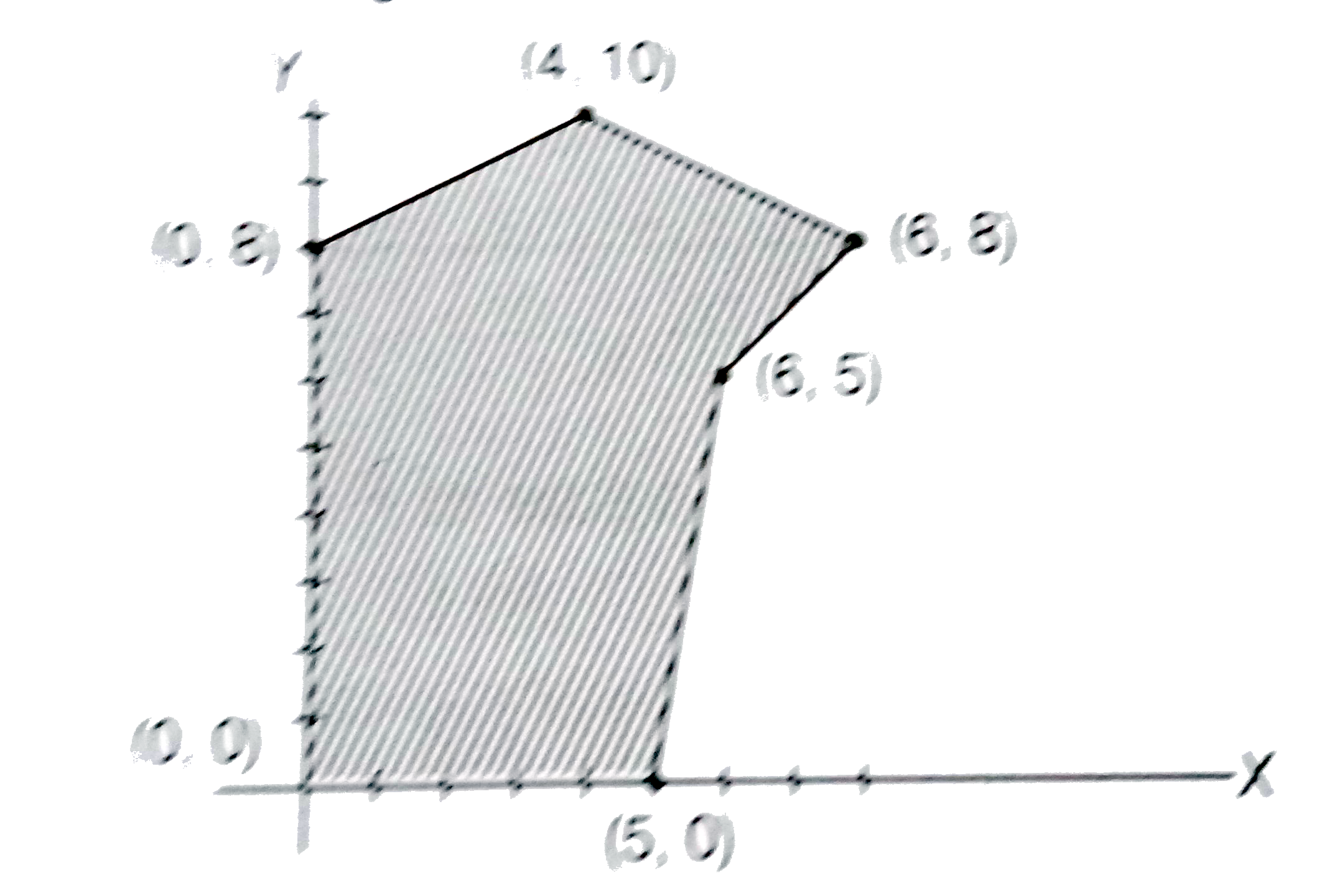
The maximum value of Z = 4x + 3y, if the feasible region for an LPP is shown in following figure, is
 View Solution
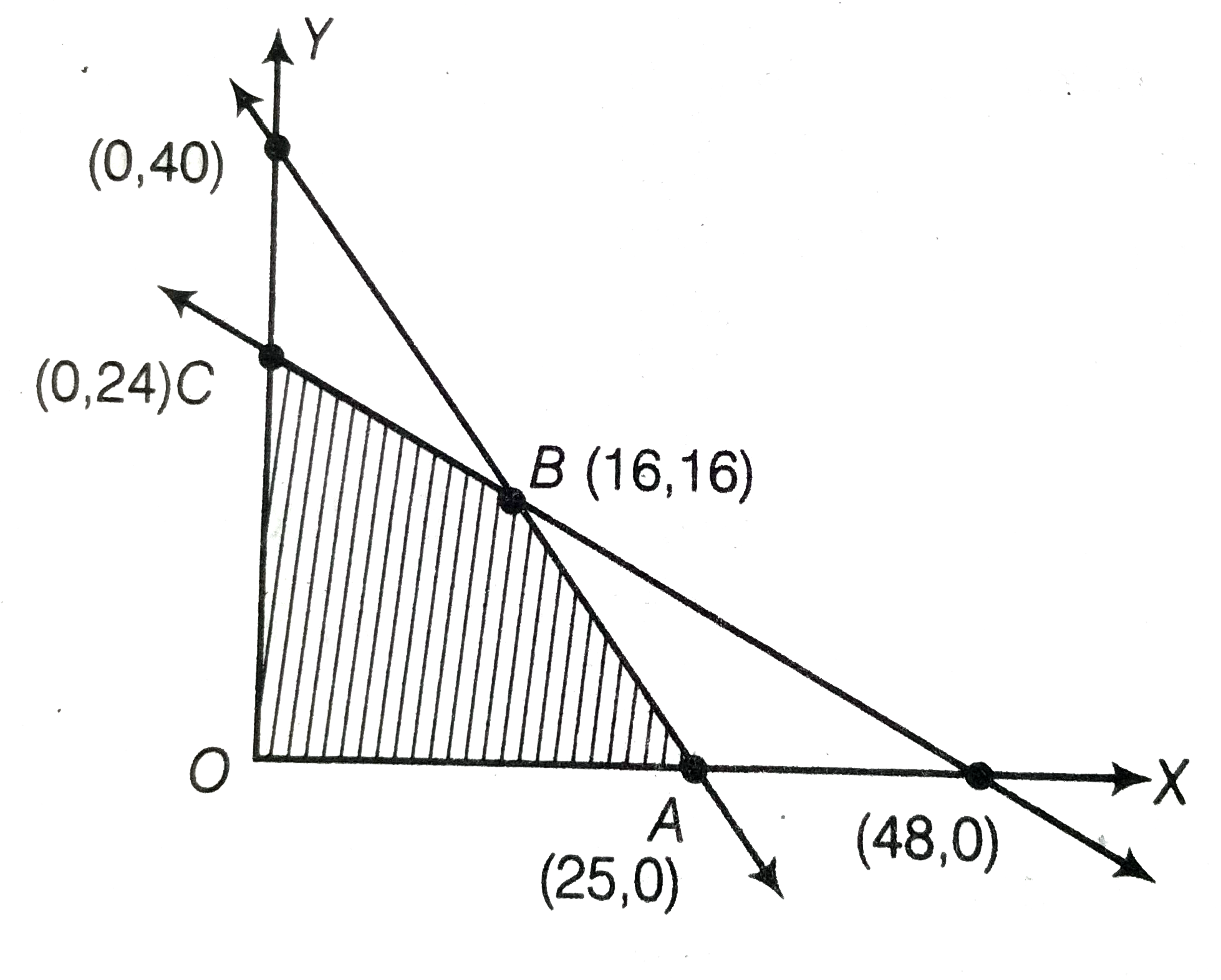
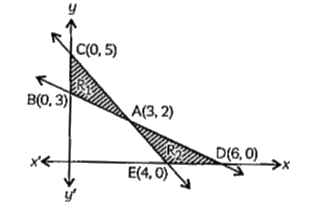
View SolutionThe feasible regions for two LPP is show in the following figure.
Based on the given information, answer the following questions:
If R2 is the feasible region and the objective function is Z2=4x+3y, then the minimum value of Z2 occurs at:
 View Solution
View SolutionThe feasible region for an LPP is always a..polygon
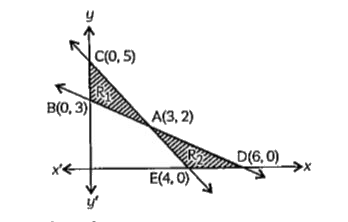
View SolutionThe feasible regions for two LPP is show in the following figure.
Based on the given information, answer the following questions:
If R1 is the feasible region, and the ojective function is Z1=2x−y, then the maximum value of Z1 occurs at:
 View Solution
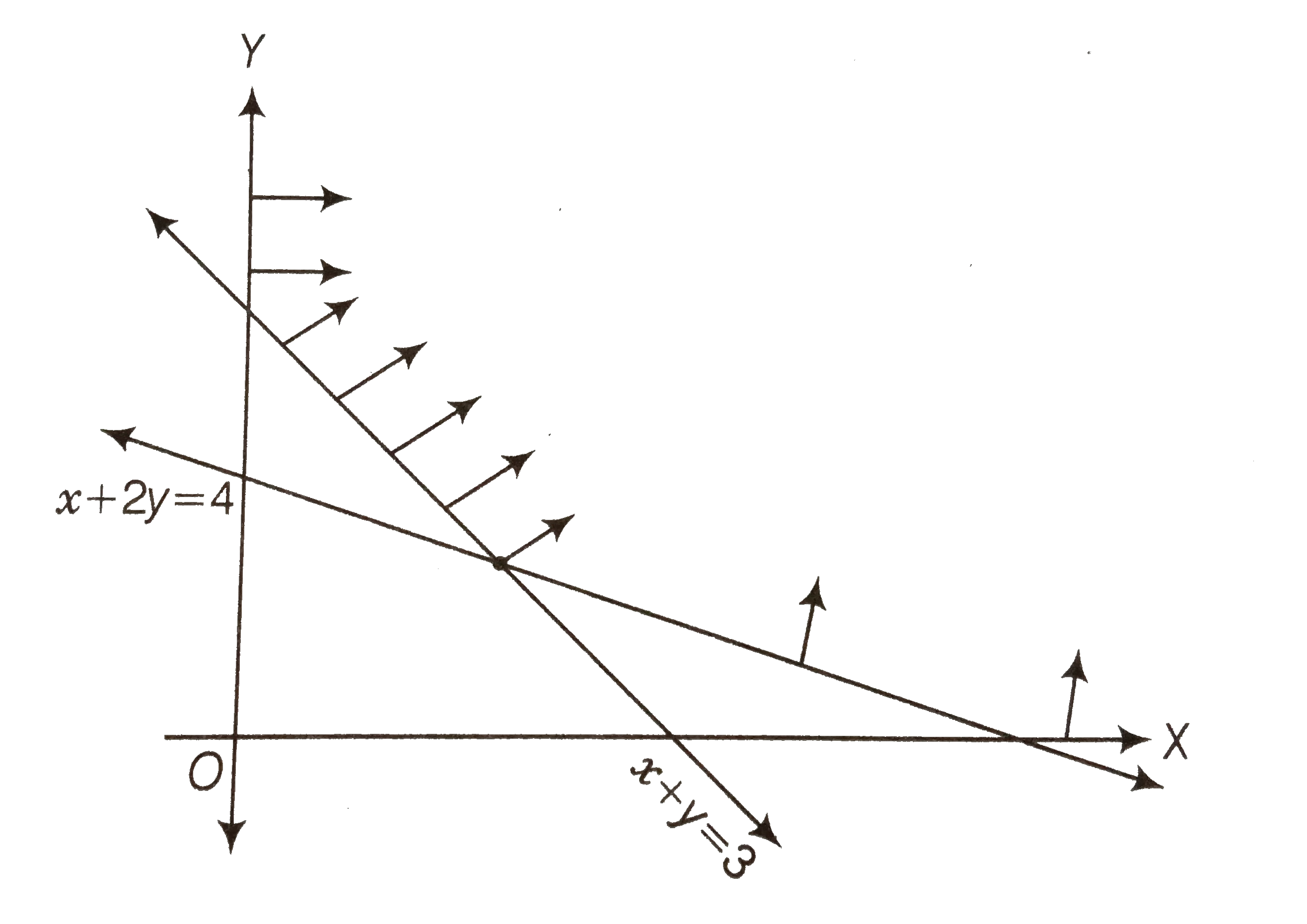
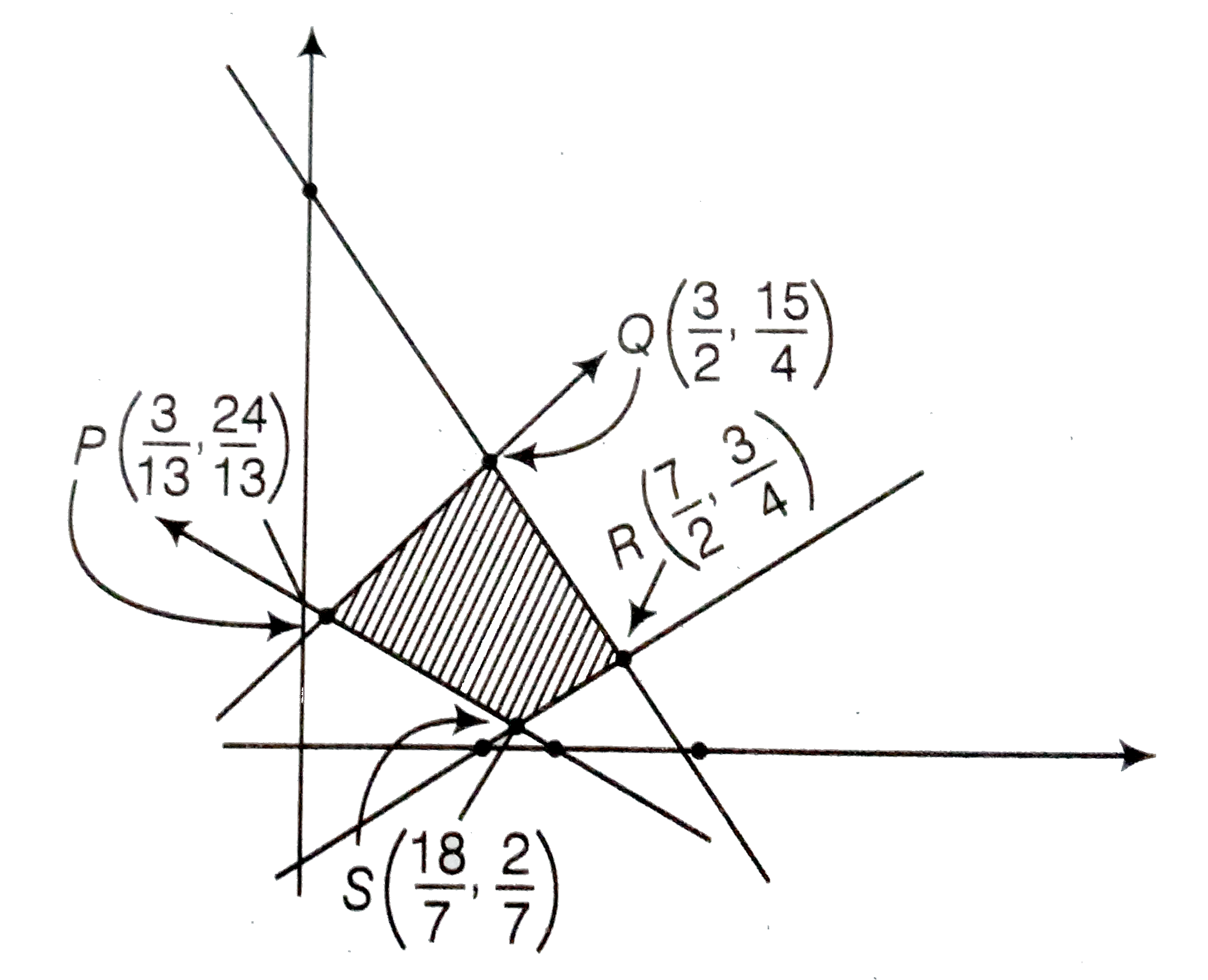
View SolutionIn the following the feasible region (shaded) for a LPP is shown Determine the maximum and minimum value of Z=x+2y
 View Solution
View Solution

