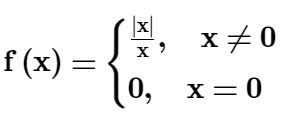If f(x)={xnsin(1x2)x≠00x=0, (n∈I), then (a) limx→0f(x) exists for n>1 (b) limx→0f(x) exists for n<0 (c) limx→0f(x) does not exist for any value of n (d) limx→0f(x) cannot be determined
Video Solution
|
Answer
Step by step video & image solution for If f(x)={(x^nsin(1/(x^2)),x!=0), (0,x=0):}, (n in I), then (a) lim_(xrarr0)f(x) exists for n >1 (b) lim_(xrarr0)f(x) exists for n<0 (c) lim_(xrarr0)f(x) does not exist for any value of n (d) lim_(xrarr0)f(x) cannot be determined by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If f(x)={xsin(1x)x≠00x=0 Then, limx→0f(x)
Ais equal to 1Bis equal to−1Cis equal to 0Ddoes not existIf limx→0ϕ(x)=a3,a≠0, then limx→0ϕ(xa) is
Aa2B1a3C1a2Da3If limx→0ϕ(x)=a3,a≠0, then limx→0ϕ(xa) is
Aa2B1a3C1a2Da3