A rectangle has two lines of symmetry, each one of which is the line joining the midpoints of opposite sides.Also a rectangle has rotational symmetry of order 2, when rotated through 180∘ and 360∘.
To solve the problem regarding the lines of symmetry and rotational symmetry of a rectangle, we can break it down into clear steps:
1. Understanding Lines of Symmetry:
- A rectangle has two lines of symmetry. These lines are drawn by connecting the midpoints of opposite sides.
- For example, if we have a rectangle ABCD, the line of symmetry can be drawn from the midpoint of side AB to the midpoint of side CD, and another from the midpoint of side AD to the midpoint of side BC.
2. Drawing the Lines of Symmetry:
- Identify the midpoints of each side of the rectangle.
- Draw a vertical line connecting the midpoints of the left and right sides (AD and BC).
- Draw a horizontal line connecting the midpoints of the top and bottom sides (AB and CD).
- These two lines divide the rectangle into symmetrical halves.
3. Understanding Rotational Symmetry:
- Rotational symmetry refers to the property of a shape that looks the same after a certain amount of rotation.
- A rectangle has a rotational symmetry of order 2. This means that it can be rotated by 180 degrees and 360 degrees and still look the same.
4. Demonstrating Rotational Symmetry:
- When you rotate the rectangle 180 degrees, each corner moves to the position of the opposite corner, and the rectangle appears unchanged.
- When you rotate it 360 degrees, it completes a full turn and returns to its original position.
5. Additional Rotations:
- If you rotate the rectangle by 90 degrees, it will not look the same as the original rectangle.
- Similarly, rotating it by 270 degrees will also not result in the original rectangle.
Conclusion:
- A rectangle has two lines of symmetry and a rotational symmetry of order 2, specifically at 180 degrees and 360 degrees.
- A rectangle has two lines of symmetry. These lines are drawn by connecting the midpoints of opposite sides.
- For example, if we have a rectangle ABCD, the line of symmetry can be drawn from the midpoint of side AB to the midpoint of side CD, and another from the midpoint of side AD to the midpoint of side BC.
- Identify the midpoints of each side of the rectangle.
- Draw a vertical line connecting the midpoints of the left and right sides (AD and BC).
- Draw a horizontal line connecting the midpoints of the top and bottom sides (AB and CD).
- These two lines divide the rectangle into symmetrical halves.
- Rotational symmetry refers to the property of a shape that looks the same after a certain amount of rotation.
- A rectangle has a rotational symmetry of order 2. This means that it can be rotated by 180 degrees and 360 degrees and still look the same.
- When you rotate the rectangle 180 degrees, each corner moves to the position of the opposite corner, and the rectangle appears unchanged.
- When you rotate it 360 degrees, it completes a full turn and returns to its original position.
- If you rotate the rectangle by 90 degrees, it will not look the same as the original rectangle.
- Similarly, rotating it by 270 degrees will also not result in the original rectangle.
- A rectangle has two lines of symmetry and a rotational symmetry of order 2, specifically at 180 degrees and 360 degrees.
Topper's Solved these Questions
- REFLECTION AND ROTATIONAL SYMMETRYBOOK - RS AGGARWALCHAPTER - REFLECTION AND ROTATIONAL SYMMETRYEXERCISE - Exercise A(MCQ)
10 Videos
- REFLECTION AND ROTATIONAL SYMMETRYBOOK - RS AGGARWALCHAPTER - REFLECTION AND ROTATIONAL SYMMETRYEXERCISE - Exercise A(True / False)
7 Videos
- RATIONAL NUMBERSBOOK - RS AGGARWALCHAPTER - RATIONAL NUMBERSEXERCISE - TEST PAPER -(4)
17 Videos
- SIMPLE INTEREST BOOK - RS AGGARWALCHAPTER - SIMPLE INTEREST EXERCISE - test perper 12 D
1 Videos
Similar Questions
Knowledge Check
A square has a rotational symmetry of order
A1B2C3D4Which has no rotation of symmetry ?
AhexagonalBOrthochromicCCubicDTriclinicWhich has no rotaition of symmetry ?
AHexagonalBOrthorhombicCCubicDTriclinic
A kite has two lines of symmetry.
View SolutionThe given figure has rotational symmetry of order
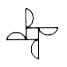
View SolutionA square has 4 lines of symmetry, namely the diiagonals and the lines joining the midpoints of its opposite sides. true or false?
View SolutionA square has four lines of symmetry. Namely. The diagonals and the lines joining the midpoints of its opposite sides. true or false?
View SolutionThe rectangle has rotational symmetry of order (a) 1 (b) 2 (c) 3 (d) 4
View Solution
