Find out the maximum possible output for a firm with zero unit of L and 10 units of K when its production function is : Q=5L+2K.
Given : Q=5L+2K. And L=0 units, K=10 units
Putting the values of L and K in the f=given production, we get:
Q=5(0)+2(10)
Q or Maximum output =20 units.
Topper's Solved these Questions
- PRODUCTION FUNCTIONBOOK - SANDEEP GARGCHAPTER - PRODUCTION FUNCTIONEXERCISE - Very Short Answer Type Question
25 Videos
- PRODUCTION FUNCTIONBOOK - SANDEEP GARGCHAPTER - PRODUCTION FUNCTIONEXERCISE - REVISION EXERCISE
40 Videos
- PRODUCTION FUNCTIONBOOK - SANDEEP GARGCHAPTER - PRODUCTION FUNCTIONEXERCISE - TRUE AND FALSE
30 Videos
- PRODUCER'S EQUILIBRIUMBOOK - SANDEEP GARGCHAPTER - PRODUCER'S EQUILIBRIUMEXERCISE - Unsolved Practicals
12 Videos
- REVENUE BOOK - SANDEEP GARGCHAPTER - REVENUE EXERCISE - UNSOLVED PRACTICALS
21 Videos
Similar Questions
Let the production function of a firm be : Q=5L½K½. Find out the maximum possible output that the firm can produce with 100 units of L and 100 units of L and 100 units of K.
View SolutionLet the production function of a firm be : Q=2L2K2. Find out the maximum possible output that the firm can produce with 5 units of L and 2 units of K. What is the maximum possible output that the firm can produce with zero unit of L and 10 units of K ?
View Solution
Knowledge Check
The maximum possible output for a firm with two units of labour (L) and ten units of capital (K), if its production function is given as : 5L+2K
A0 unitsB30 unitsC200 unitsD50 unitsTC of a firm increases by Rs. 250 when its output level increases from 50 units to 60 units. MC of the firm:
ARs. 5BRs. 4.16CRs. 25Dnone of these
Let the production function of a firm be Q=2L2K2. Find out the maximum possible output that the firm can produce with 5 units of L and 2 units of K. What is the maximum possible output that the firm can produce with zero unit of L and 10 units of K?
View SolutionLet the production function of a firm be Q=5L1/2K1/2 . Find the maximum possible output that the firm can produce with 100 units of L and 100 units of K.
View SolutionLet the production function of a firm be Q=5L1/2K1/2 . Find the maximum possible output that the firm can produce with 100 units of L and 100 units of K.
View SolutionA firm knows that demand function for its main product is linear. It also knows that it can sell 3000 units at ₹ 5 per unit and it can sell 1200 units when price is ₹ 11 per unit. Find the demand function.
View SolutionThe total cost function of a firm is C=53x3−10x2+32x+15, where C is the total cost and x is the output. A tax at the rate of Rs2 per unit is imposed by the Govermment and the producer adds it to its cost. Demand function is given by p=4534−10x, where p is price per unit of the output. Find the profit function
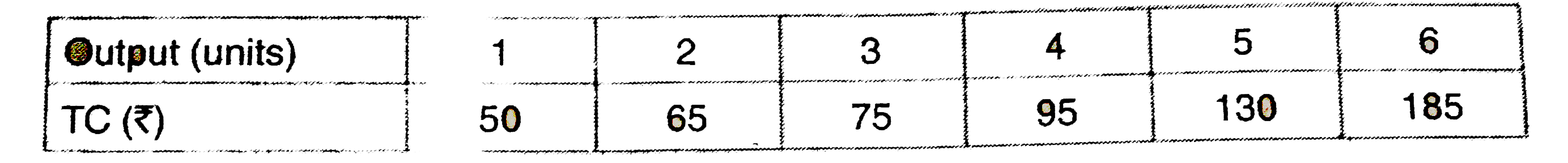
View SolutionThe following table gives the total cost schedule of firm. It is also given that the average fixed cost at 4 units of output is ₹5. Find the TVC, TFC, AVC, AFC, SAC and SMC schedules of the firm for the corresponding values of output.
View Solution
