A conducting wire XY of mass M and negligible resistance slides smothly on two parallel conducting wires as shown in figure. The closed circuit has a resistance R due to AC. AB and CD are perfect conductors. There is a magnetic field B=B(t)ˆk
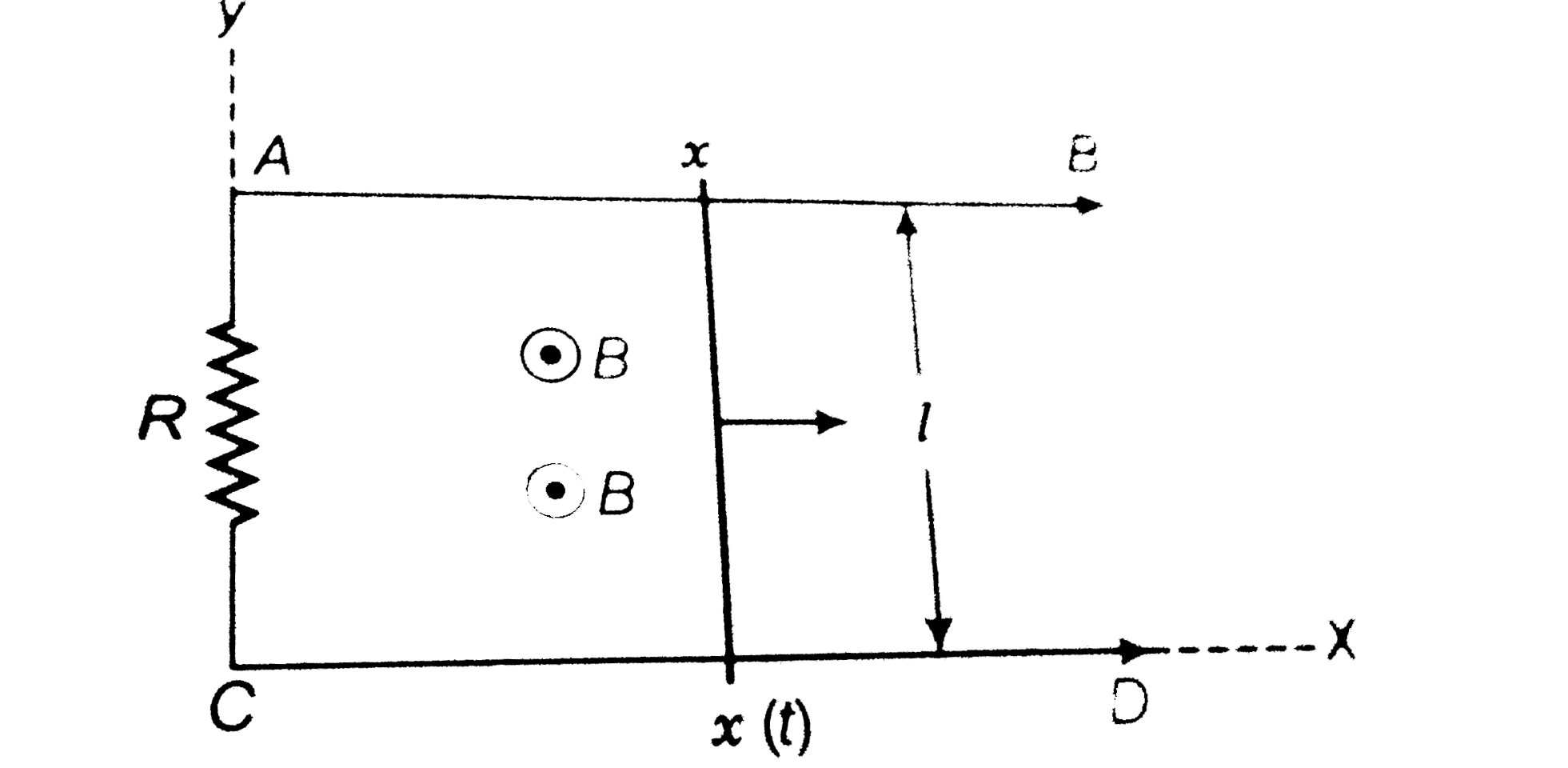
Let us assume that the parallel wiers at are y=0, i.e., along x-axis and y-l, At=0, XY has x=0 i.e., along y-axis.
(i) Let the wire be at x=x(t) at time t.
The magnetic flux linked with the loop is given by
θ=B.A=BAcos0=BA
at any instant t
Magnetic flux = B(t)(l×x(t))
Total emf in the circuit= emf due to change in field (along XYAC) + the motional emf across XY
E=−dϕdt=−(dB(t)dtlx(t)−B(t)lv(t) [second term due to motional emf]
Electric current in clockwise direction is given by
I=1RE
The force acting on the conductor is given by F=ilBsin90∘=ilB
Substituting the values, we have
Force =IB(t)R[−dB(t)dtIx(t)−B(t)Iv(t)]ˆi
Applying Newton's second law of motion,
md2xdt2=−I2B(t)RdBdtx(t)−I2B2(t)Rdxdt
Which is the required equation.
ii) If B is independent of time i.e., B=Constant
Or dBdt=0
Substituting the above value in Eq(i), we have
d2xdt2+I2B2mRdxdt=0
dvdt+I2B2mRV=0
Integrating using variable separable form of differential equation, we have
V=Aexp(−I2B2tmR)
Applying given conditions
at t=0v=u0
v(t)=u0exp(−I2B2t/mR)
This is the required equation,
Since the power consumption is given by P=I2R
Here,
I2R=B2I2V2(t)R2×R
=B2I2Ru20exp(−2I2B2tImR)
Now, energy consumed in time interval dt is given by energy consumed =Pdt=I2Rdt
Therefore, total energy consumed in time t
=∫t0I2Rdt=B2I2Rμ20mR2I2B2[1−e−(l2B2t/mr)
m2u20−m2v2(t)
= decrease in kinetic energy.
This proves that the decrease in kinetic energy of XY equals the heat lost in R.
Topper's Solved these Questions
Similar Questions
A rod of mass m and resistance R slides smoothly over two parallel perfectly conducting wires kept sloping at an angle θ with respect to the horiaontal, Fig. The circuit is closed through a perfert conductor at the top. There is a constant magnetic field B along the vertical direction. If the rod is initially at rest, find the velocity of the rod as a function of time.
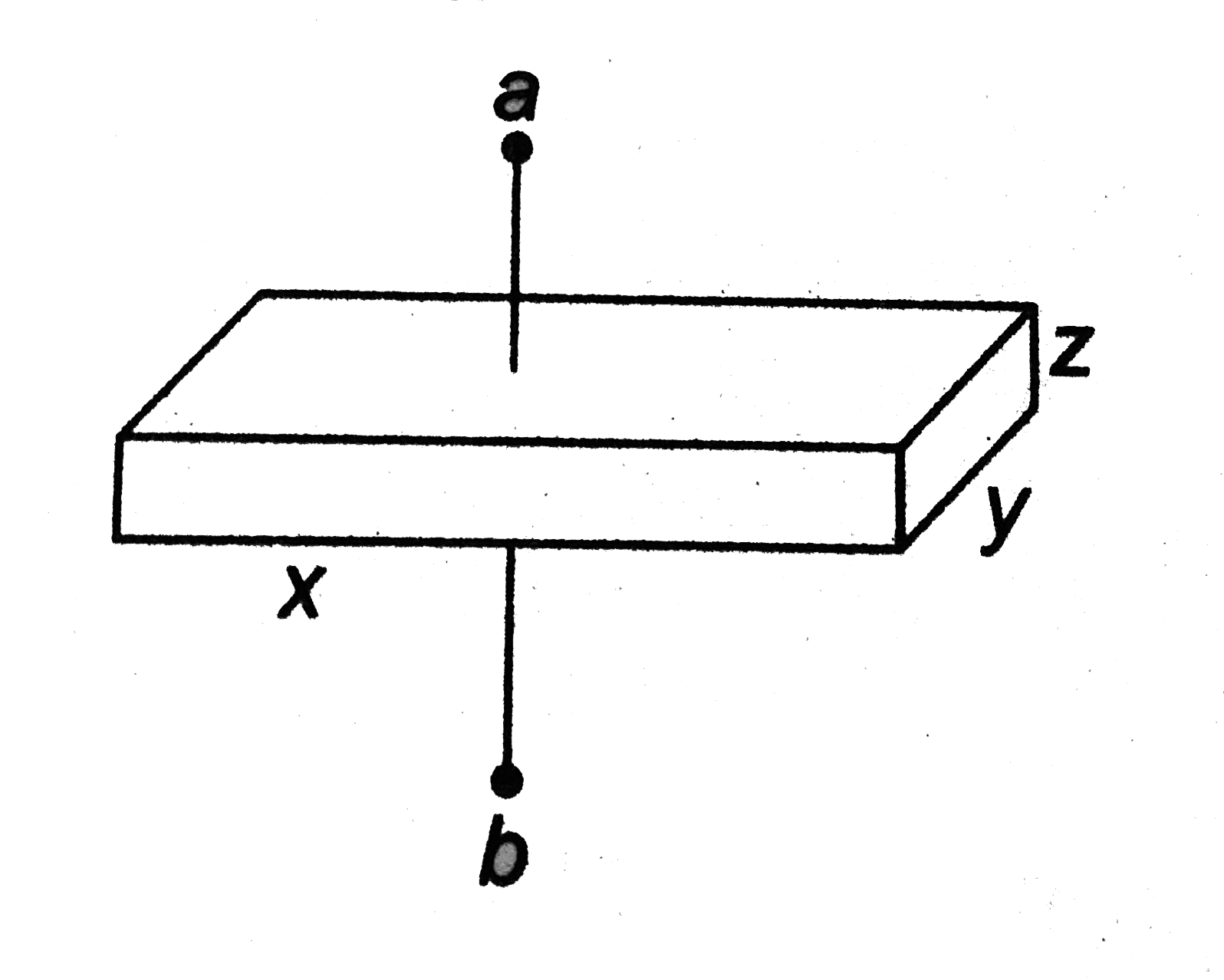
View SolutionThermal conductivity of the conductor shown in figure is K. Find thermal resistance between points a and b.
 .
.
View Solution
Knowledge Check
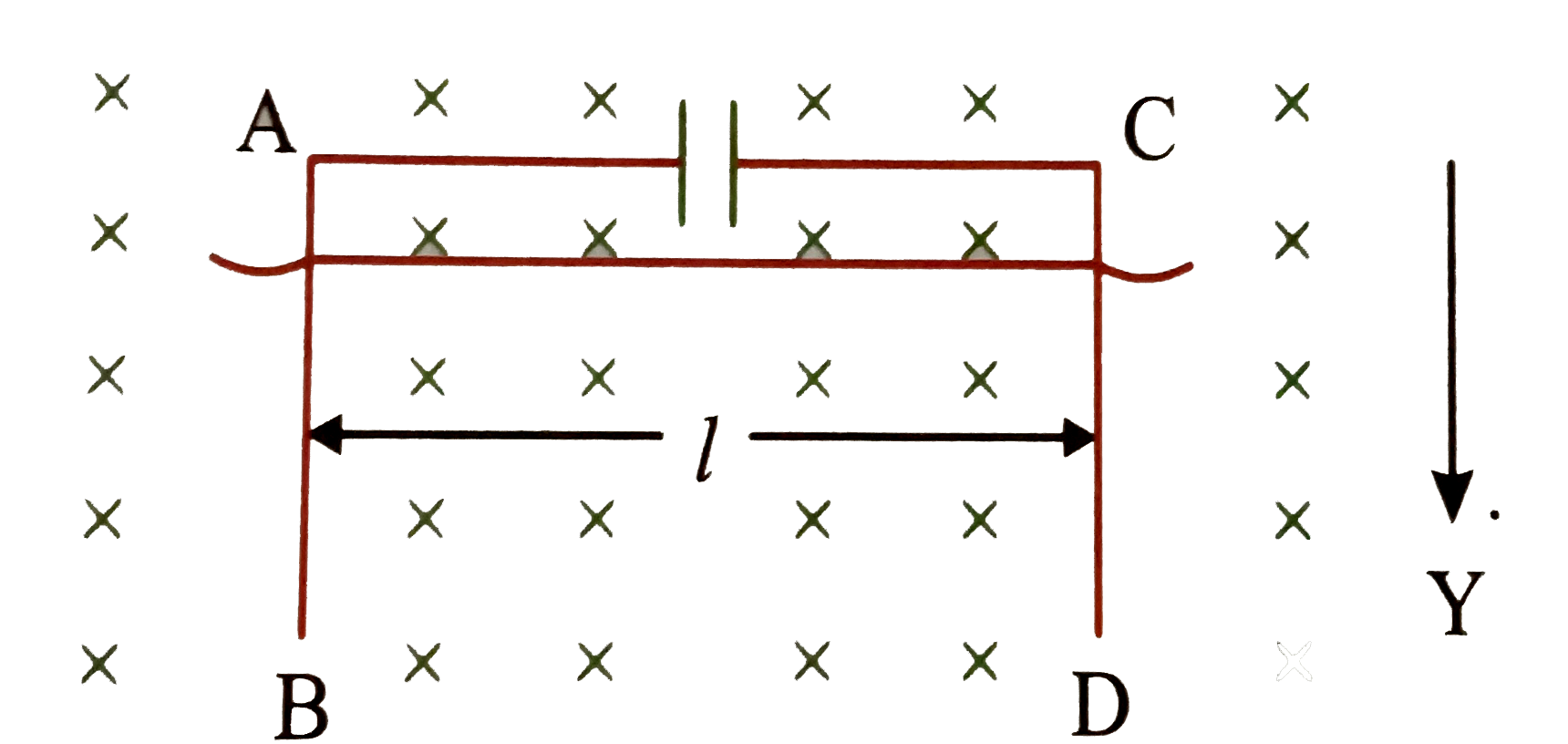
A conductor of mass m and length l is sliding smoothly an two vertical conducting rails AB and CD as shown in figure. The top ends of two conducting rails are joined by a capacitor of capacitance C. The conductor is relased from rest when it is very close to AC i.e., y=0. A uniform magnetic field B0 perpendicular to plane of figure existing. Neglect the resistance of rails and connecting wires. Take acceleration due to gravity to be g.
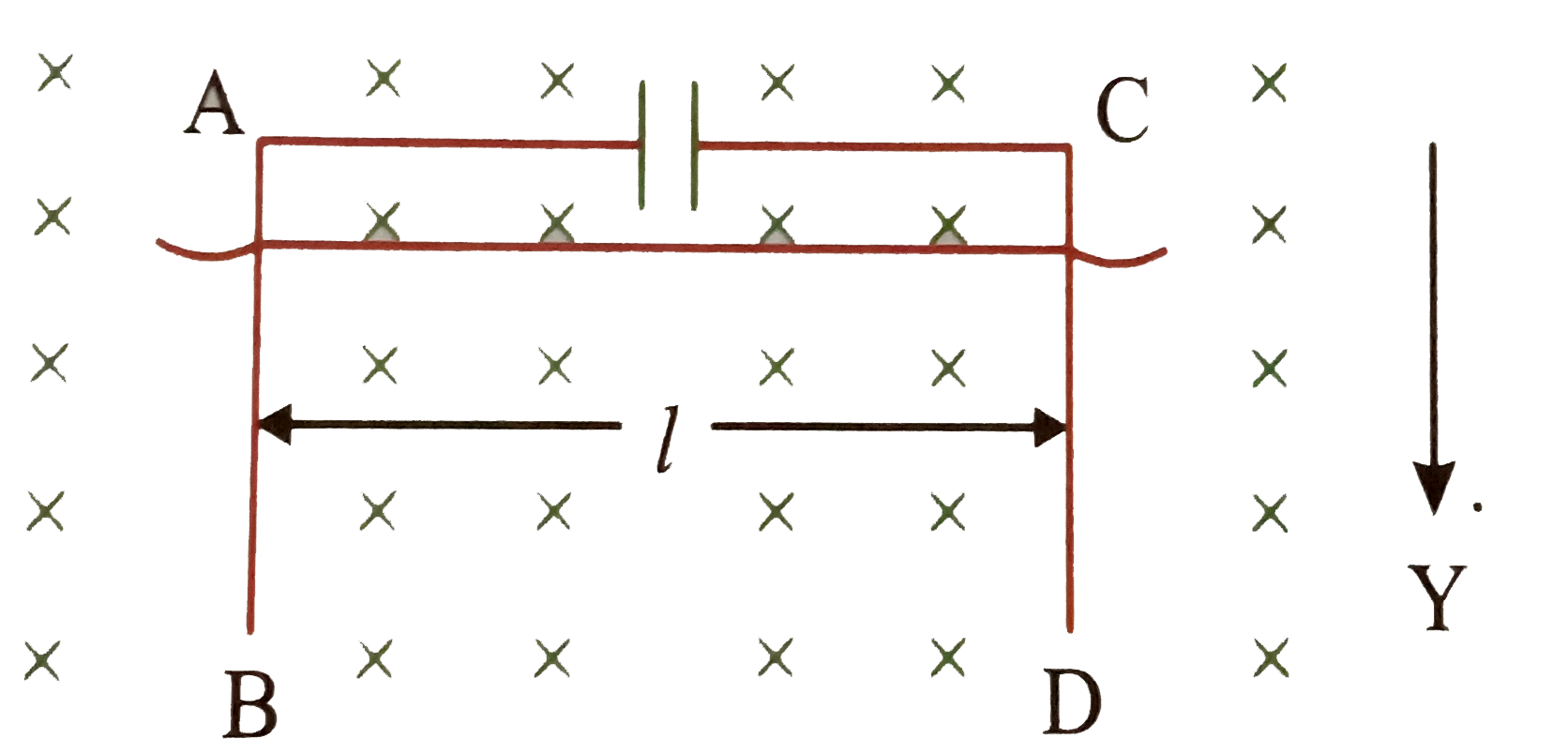
Based on above information answer the following questions:
Current in the circuit isAconstantBincreasing with timeCdecreasing with timeDFirst increase, then reaches a maximum value, and then starts decreasing to attain a constant value finallyA conductor of mass m and length l is sliding smoothly an two vertical conducting rails AB and CD as shown in figure. The top ends of two conducting rails are joined by a capacitor of capacitance C. The conductor is relased from rest when it is very close to AC i.e., y=0. A uniform magnetic field B0 perpendicular to plane of figure existing. Neglect the resistance of rails and connecting wires. Take acceleration due to gravity to be g.
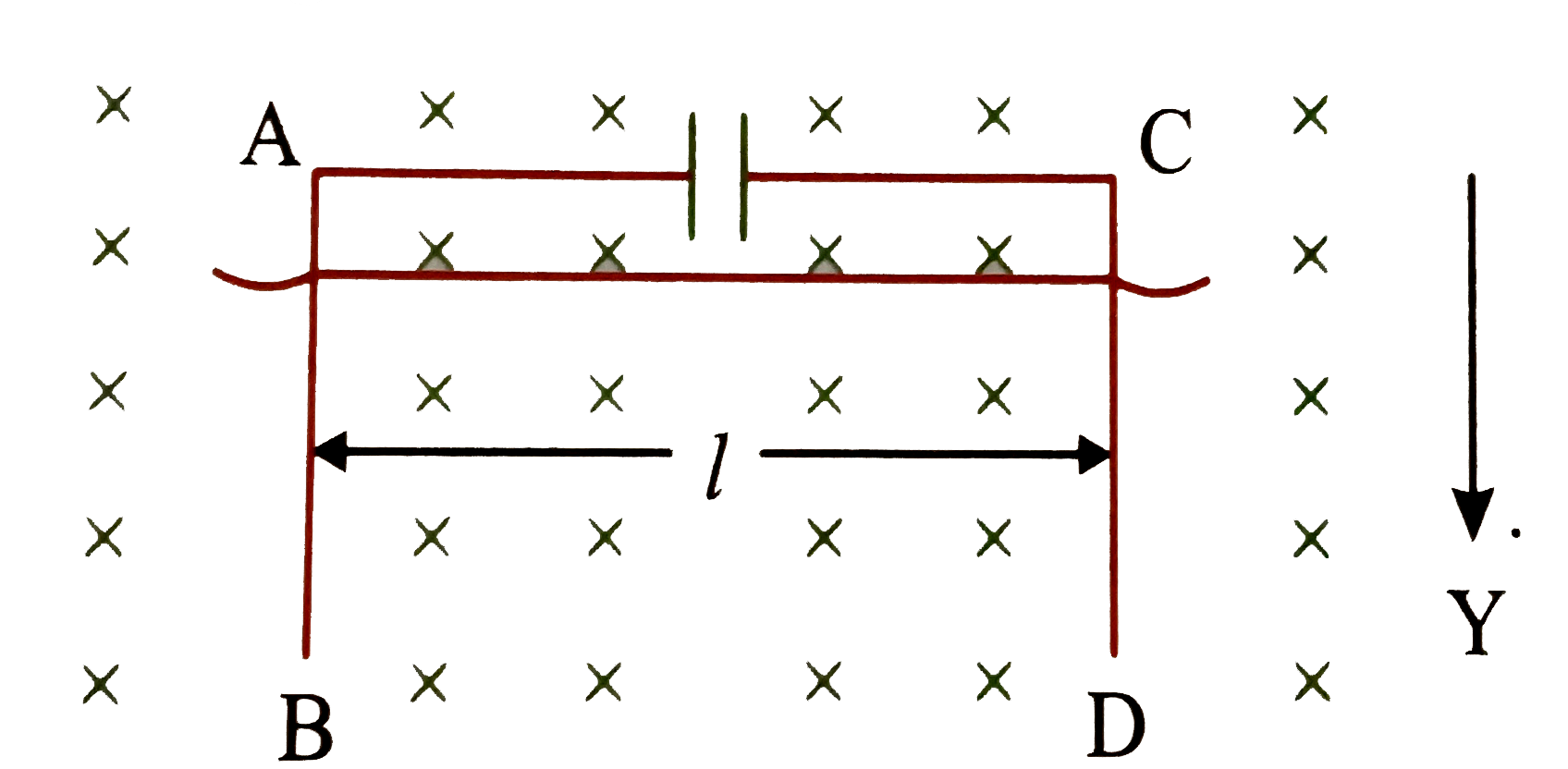
Based on above information answer the following questions:
Mark the correct statement about the motion of conductorAIt is falling down with constant acceleration gBIt is falling down with constant acceleration but not equal to gCIt is falling down with increasing accelerationDIt is falling down with decreasing accelerationA conductor of mass m and length l is sliding smoothly an two vertical conducting rails AB and CD as shown in figure. The top ends of two conducting rails are joined by a capacitor of capacitance C. The conductor is relased from rest when it is very close to AC i.e., y=0. A uniform magnetic field B0 perpendicular to plane of figure existing. Neglect the resistance of rails and connecting wires. Take acceleration due to gravity to be g.
Based on above information answer the following questions:
Charge on the capacitor as a function of distance travelled y is given byACB0l√2mgym+CB20l2BCB0l√m+CB20l22mgyCCB0lemgym+CB20l2DCB0l√2mgym+CB20l2×emgym+CB20l2
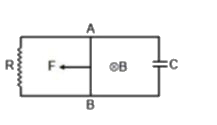
A conducting rod AB of mass m, has negligible resistance and of length 0.5 m slides on a pair of parallel metalic rails, which are connected by a resistance R=10Ω and capacitance C=1μF as shown in the figure. A magnetic field B=1T is applied normal and into the plane of the circuit. At tim t=0 a force F=0.4N is applied on the rod AB. What will be the terminal speed acquired by rod?
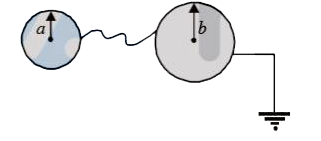
View SolutionTwo conducting shells of radius a and b are connected by conducting wire as shown in figure. The capacity of system is :
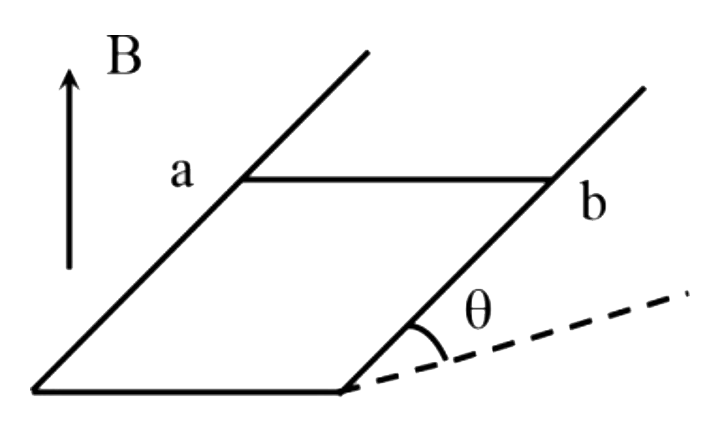
View SolutionA wire ab of length l, mass m and resistance R slides on a smooth thick pair of metallic rails joined at the bottom as shown in fig. The plane of the rails makes an angle θ with the horizontal. A vertical magnetic field B exist in the region. If the wire slides on the rails at a constant speed v, then the value of B is -
View SolutionA horizontal wire is free to slide on the vertical rails of a conducting frame as shown in figure. The wire has a mass m and length l and the resistance of the circuit is R. If a uniform magnetic field B is directed perpendicular to the frame, the terminal speed of the wire as it falls under the force of gravity is

View SolutionA conducting wire is moving towards right in a magnetic field B. The direction of induced current in the wire is shown in the figure. The direction of magnetic field will be

View Solution
