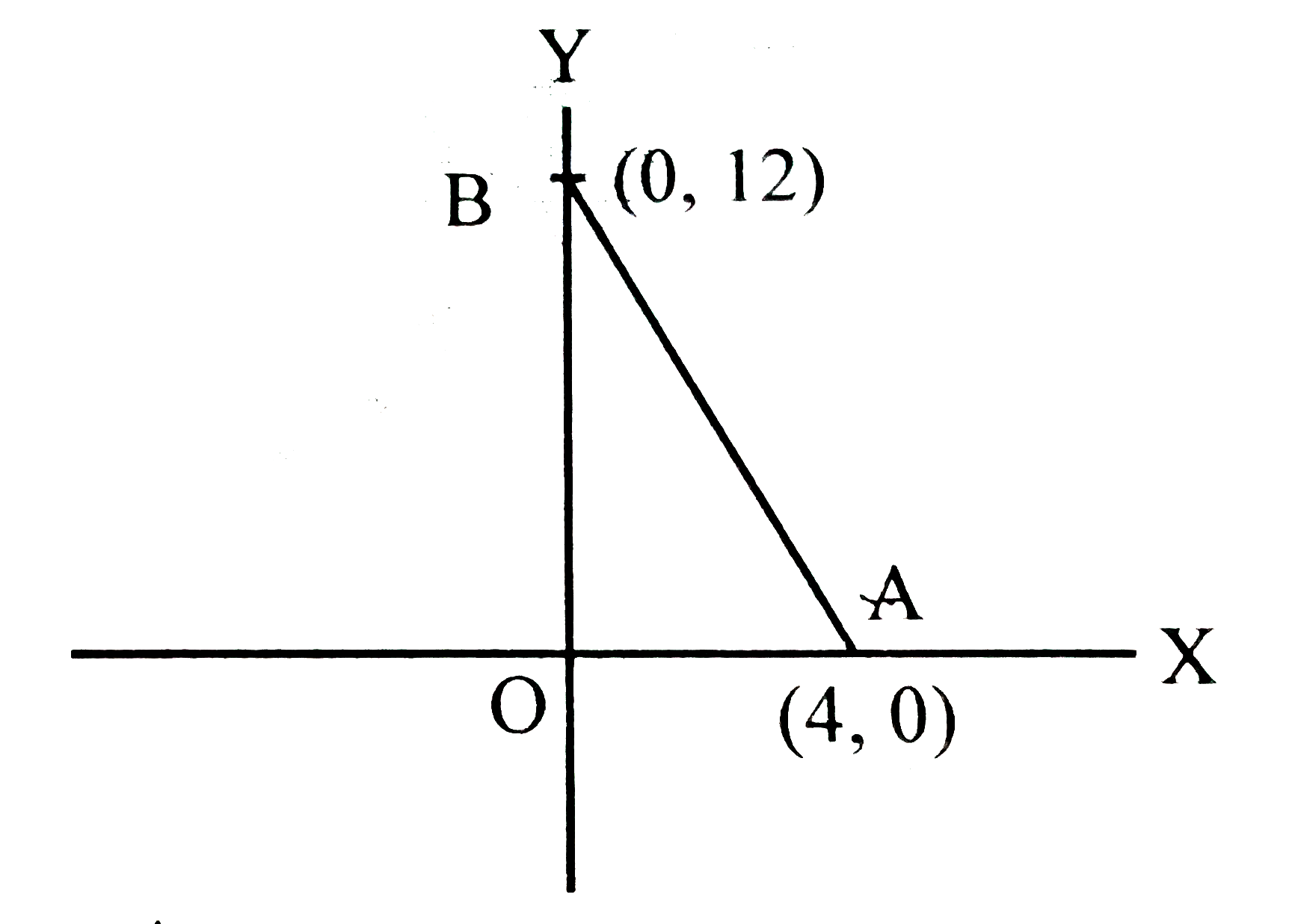
Let $A = (4, 0), B = (0, 12)$ be two points in the plane. The locus of a point C such that the area of triangle ABC is 18 sq. units is -

A

${(y + 3 x + 12)}^{2} = 81$

B

${(y + 3 x + 81)}^{2} = 12$

C

${(y + 3 x - 12)}^{2} = 81$

D

${(y + 3 x - 81)}^{2} = 12$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$\frac{1}{2} ∣ ∣ ∣ ∣ ∣ \begin{matrix} 1 & x & y 1 & 0 & 12 1 & 4 & 0 \end{matrix} ∣ ∣ ∣ ∣ ∣ = \pm 18$
$1 (- 48) - x (- 12) + y (4) = \pm 36$
$12 x + 4 y - 48 = \pm 36$
$3 x + y - 12 = \pm 9$
${(3 x + y - 12)}^{2} = 81$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Question 1 - Select One
Let O(0, 0) and (0, 1) be two fixed points, then the locus of a point P such that the perimeter of $Δ A O P$ is 4, is
A $8 x^{2} - 9 y^{2} + 9 y = 18$
B $9 x^{2} - 8 y^{2} + 8 y = 16$
C $9 x^{2} + 8 y^{2} - 8 y = 16$
D $8 x^{2} + 9 y^{2} - 9 y = 18$
Question 2 - Select One
Let BC be a fixed line segment in the plane . The locus of a point A such that the triangle ABC is isosceles , is (with finitely many possible exceptional points )
Aa line
Ba circle
Cthe union of a circle and a line
Dthe union of two circles and a line
Question 3 - Select One
The locus of P such that area of $Δ P A B$ is 12 sq. units, where A(2,3) and B(-4,5) is
A(x+3y-1) ,(x+3y-23) = 0
B(x+3y+1) ( x+3y-23) =0
C(3x+ y-1), (3x+ y-23)=0
D(3x+ y+1) (3x+ y+23)=0

Recommended Questions

Home
Ask Doubt
Profile