The number of ordered pairs (x,y) of real numbers that satisfy the simultaneous equations
$x + y^{2} = x^{2} + y = 12$ is

A

0

B

1

C

2

D

4

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

$x + y^{2} = x^{2} + y = 12$
$curve (1) x + y^{2} = 12 curve (2) x^{2} + y = 12$
$y^{2} = - (x - 12) x^{2} = - (y - 12)$
Intersection on x-axis (2,0) Intersection on x-axis $= (\pm \sqrt{12}, 0)$
Intersection on y-zxis $(0, \pm \sqrt{12}) I n t e r sec t i o n o n y - a ξ s = (0, 12)$

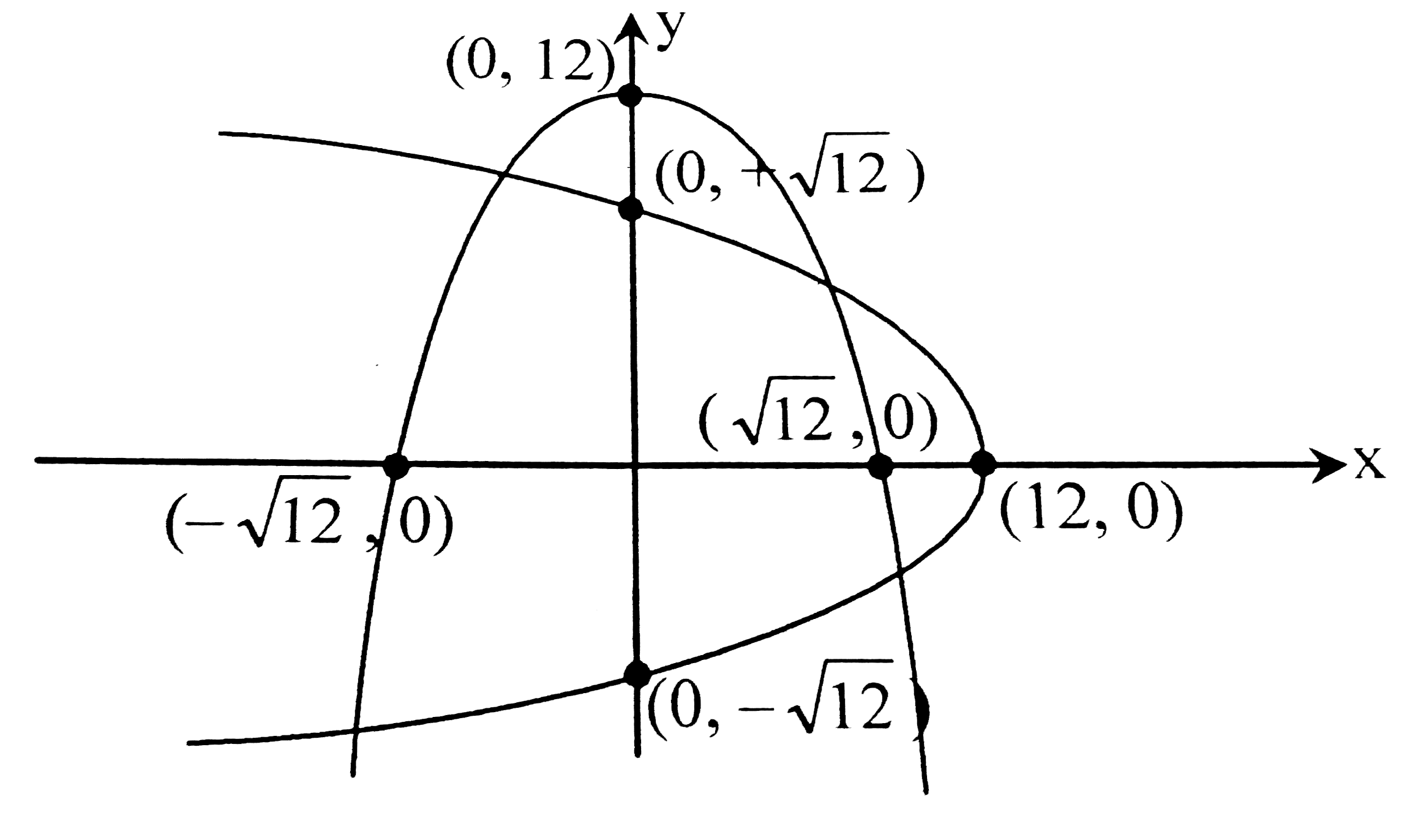
four intersection

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Question 1 - Select One
The number of ordered pair(s) $(x, y)$ of real numbers satisfying the equation $1 + x^{2} + 2 x sin ({cos}^{- 1} y) = 0$ , is :
A0
B1
C2
D3
Question 2 - Select One
The number of ordered pairs (x, y) of integers satisfying $x^{3} + y^{3} = 65$ is
A0
B2
C4
D6
Question 3 - Select One
The number of all possible ordered pairs (x, y) x, y $\in$ R satisfying the system of equations
$x + y = \frac{2 π}{3}, cos x + cos y = \frac{3}{2},$ is
A0
B1
Cinfinite
Dnone of these

Recommended Questions

Home
Ask Doubt
Profile