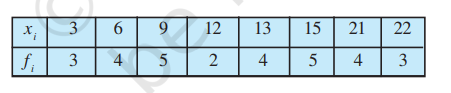
Find the mean deviation from the median for the following data:
{size:1011121415frequency:23834
To find the mean deviation from the median for the given data, we will follow these steps:
Step 1: Create a Frequency Table
We have the sizes (X) and their corresponding frequencies (f).
| Size (X) | Frequency (f) |
|----------|---------------|
| 10 | 2 |
| 11 | 3 |
| 12 | 8 |
| 14 | 3 |
| 15 | 4 |
Step 2: Calculate the Total Frequency (n)
To find the total frequency, we sum up all the frequencies.
n=2+3+8+3+4=20
Step 3: Find the Cumulative Frequency
Next, we calculate the cumulative frequency to help find the median.
| Size (X) | Frequency (f) | Cumulative Frequency (CF) |
|----------|---------------|----------------------------|
| 10 | 2 | 2 |
| 11 | 3 | 5 |
| 12 | 8 | 13 |
| 14 | 3 | 16 |
| 15 | 4 | 20 |
Step 4: Determine the Median
The median is found at the position n2=202=10. Since the cumulative frequency just exceeds 10 at the size of 12, we conclude that the median is 12.
Step 5: Calculate the Absolute Deviations from the Median
Now we calculate the absolute deviations from the median (12) for each size:
|Xi−Median|=|Xi−12|
| Size (X) | Absolute Deviation | Frequency (f) | f×|Xi−12| |
|----------|--------------------|---------------|--------------------------|
| 10 | |10 - 12| = 2 | 2 | 2 × 2 = 4 |
| 11 | |11 - 12| = 1 | 3 | 3 × 1 = 3 |
| 12 | |12 - 12| = 0 | 8 | 8 × 0 = 0 |
| 14 | |14 - 12| = 2 | 3 | 3 × 2 = 6 |
| 15 | |15 - 12| = 3 | 4 | 4 × 3 = 12 |
Step 6: Sum of Absolute Deviations
Now we sum the values of f×|Xi−12|:
Total=4+3+0+6+12=25
Step 7: Calculate the Mean Deviation
Finally, we calculate the mean deviation from the median using the formula:
Mean Deviation=∑fi|Xi−Median|n=2520=1.25
Thus, the mean deviation from the median is 1.25.
---
We have the sizes (X) and their corresponding frequencies (f).
|----------|---------------|
| 10 | 2 |
| 11 | 3 |
| 12 | 8 |
| 14 | 3 |
| 15 | 4 |
To find the total frequency, we sum up all the frequencies.
Next, we calculate the cumulative frequency to help find the median.
|----------|---------------|----------------------------|
| 10 | 2 | 2 |
| 11 | 3 | 5 |
| 12 | 8 | 13 |
| 14 | 3 | 16 |
| 15 | 4 | 20 |
The median is found at the position n2=202=10. Since the cumulative frequency just exceeds 10 at the size of 12, we conclude that the median is 12.
Now we calculate the absolute deviations from the median (12) for each size:
|----------|--------------------|---------------|--------------------------|
| 10 | |10 - 12| = 2 | 2 | 2 × 2 = 4 |
| 11 | |11 - 12| = 1 | 3 | 3 × 1 = 3 |
| 12 | |12 - 12| = 0 | 8 | 8 × 0 = 0 |
| 14 | |14 - 12| = 2 | 3 | 3 × 2 = 6 |
| 15 | |15 - 12| = 3 | 4 | 4 × 3 = 12 |
Now we sum the values of f×|Xi−12|:
Finally, we calculate the mean deviation from the median using the formula:
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the mean deviation from the median of the following data
 A7.08B7C7.1D7.05
A7.08B7C7.1D7.05 - Question 2 - Select One
Find the mean deviation about median for the following data.
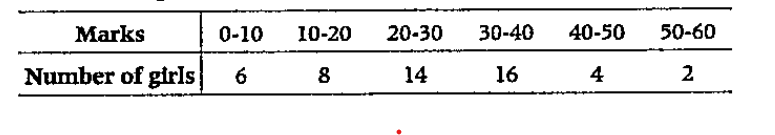 A10.34B10.50C10.40D10.37
A10.34B10.50C10.40D10.37
Find the mean deviation using median for the following datas:
 View Solution
View SolutionCalulate the mean deviation from the median of the following data
 View Solution
View SolutionFind the mean deviation from the mean for the following data :
6,7,10,12,13,4,8,12View SolutionCalculate the mean deviation from the median of the following data:
 View Solution
View SolutionConstruct mean deviation (from median) for the following data:
 View Solution
View SolutionFind the mean deviation from the mean for the following data :
4,7,8,9,10,12,13,17View Solution