The equation of smaller circle of radius √3−1 in lst quadrant having pair of straight lines (y√3−x+2)(y−x√3−2√3)=0 as its tangents , is
A
(x−2)2+(y−2)2=(√3−1)2
B
(x−4)2+(y−2)2=(√3−1)2
C
(x−4)2+(y−2)2=(√3−1)2
D
none of these
Text Solution
The correct Answer is:D
|
Topper's Solved these Questions
- STRAIGHT LINE BOOK - FIITJEECHAPTER - STRAIGHT LINE EXERCISE - Exercise 1
3 Videos
- STRAIGHT LINE BOOK - FIITJEECHAPTER - STRAIGHT LINE EXERCISE - Exercise 2
4 Videos
- STRAIGHT LINE BOOK - FIITJEECHAPTER - STRAIGHT LINE EXERCISE - SOLVED PROBLEMS (SUBJECTIVE)
12 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Comprehension Type
6 Videos
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
328 Videos
Similar Questions
Knowledge Check
The graph of straight line y=√3x+2√3 is :
A B
B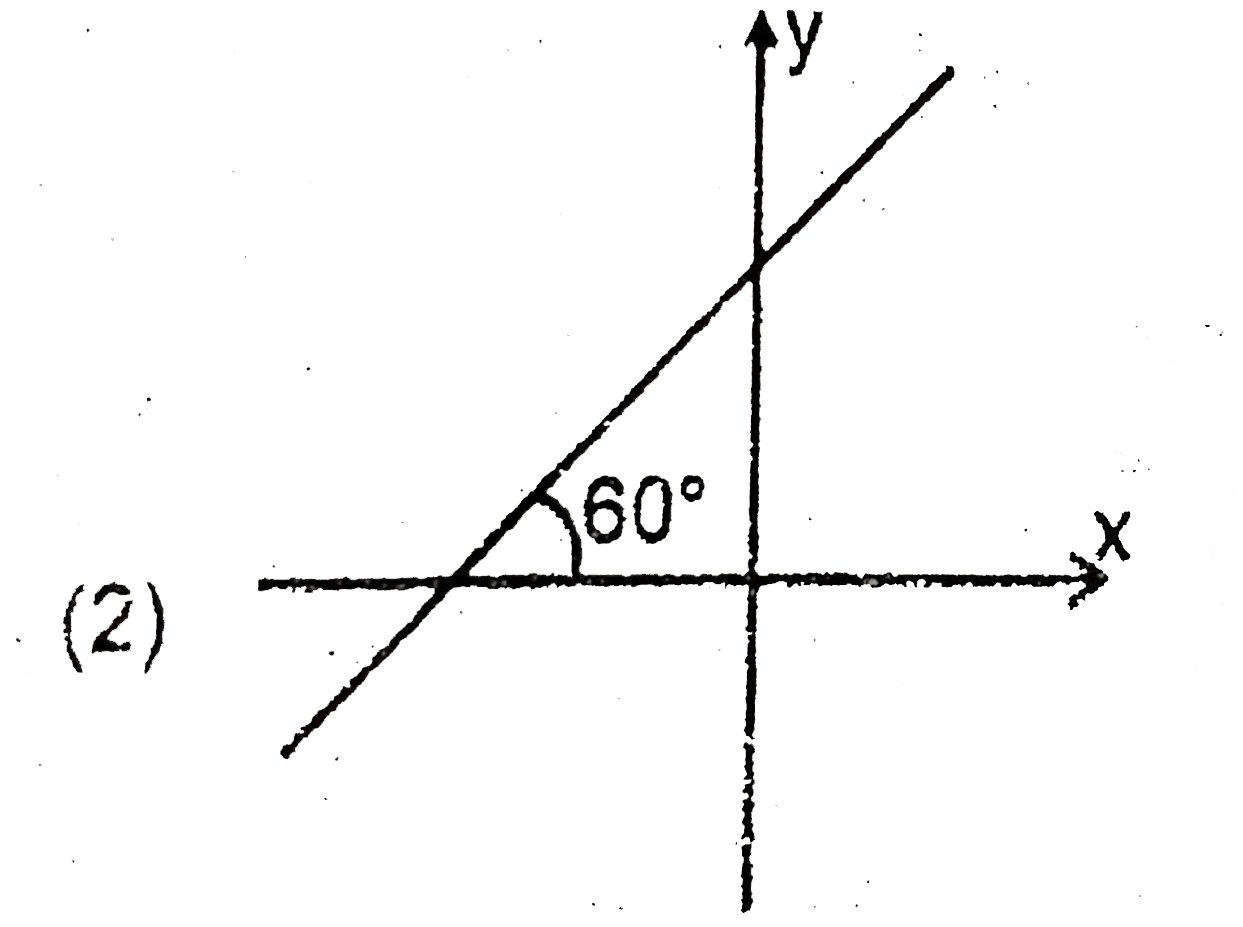 C
C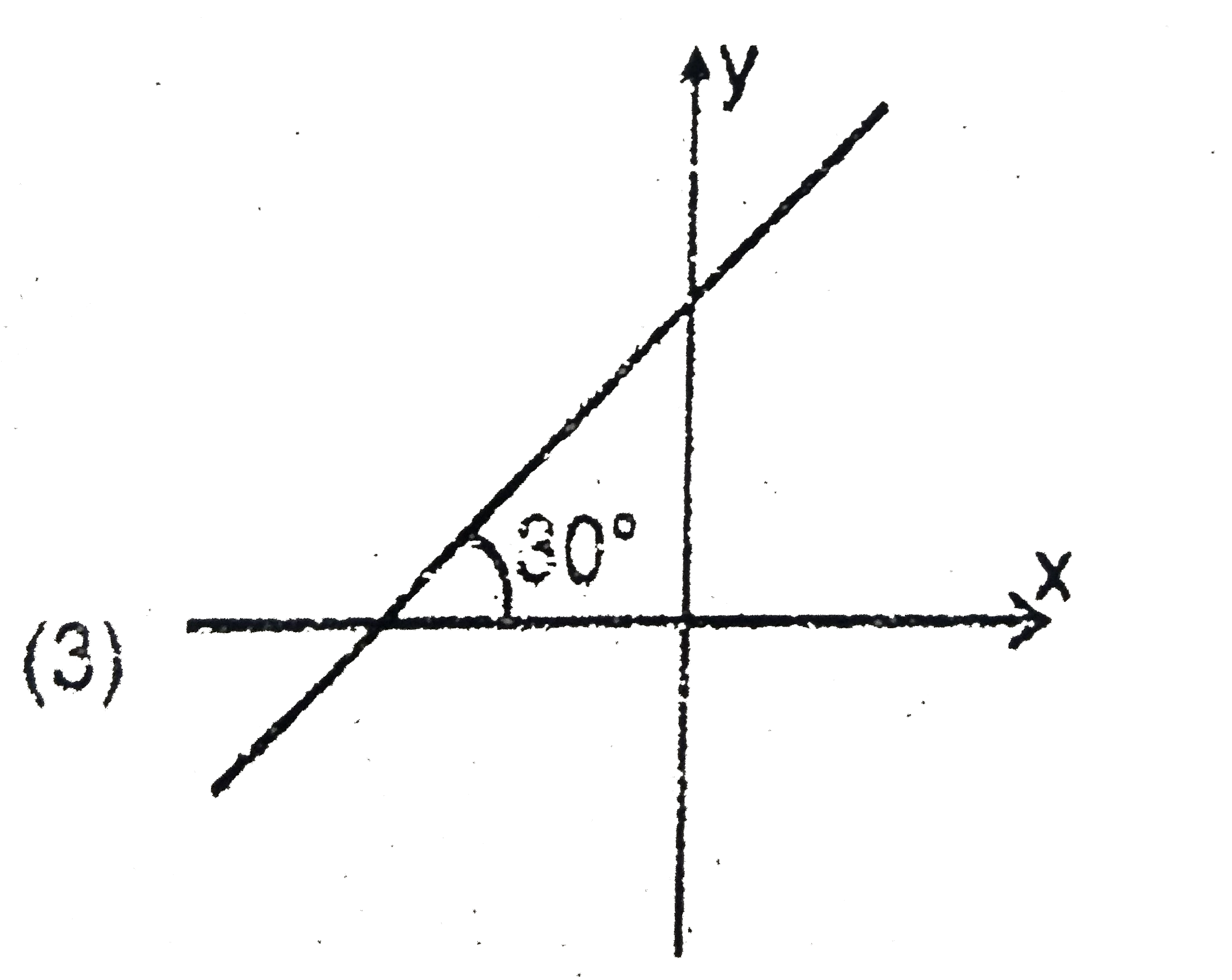 D
D
What is the acute angle between the pair of straight line √2x+√3y=1 and √3x+√2y=2 ?
Atan−1(12√6)Btan−1(1√2)Ctan−1(3)Dtan−1(1√3)The equations of the lines which pass through the point (3,-2) and are inclined at 60∘ to the line √3x+y=1 are (i) y+2=0,√3x−y−2−3√3=0 (ii) x−2=0,√3x−y+2+3√3=0 (iii) x−3=0,√3x−y−2−3√3=0 (iv) none of these
Ay+2=0,√3x−y−2−3√3=0Bx−2=0,√3x−y+2+3√3=0Cx−3=0,√3x−y−2−3√3=0Dnone of these
Find the angles between the pairs of straight lines: y=(2−√3)x+5 and y=(2+√3)x−7.
View SolutionFind the angles between the pairs of straight lines: y=(2−√3)x+5 and y=(2+√3)x−7.
View SolutionWrite the equation of the circle having radius 5 and tangent as the line 3x−4y+5=0 at (1,2)
View SolutionThe equation of two straight lines through (7,9) and making an angle of 60∘ with the line x−√3y−2√3=0 is
View SolutionIf a circle of radius 3 units is touching the lines √3y2−4xy+√3x2=0 in the first quadrant then length of chord of contact to this circle is :
View Solution
