If the mid points of consecutive of a quadrilateral connected by straight lines prove that the resulting quadrilateral is a parallelogram.
To prove that the quadrilateral formed by the midpoints of the sides of a given quadrilateral is a parallelogram, we can follow these steps:
Step 1: Define the Quadrilateral
Let the vertices of the quadrilateral be A,B,C,D.
Step 2: Identify the Midpoints
Identify the midpoints of the sides:
- Let E be the midpoint of side AB.
- Let F be the midpoint of side BC.
- Let G be the midpoint of side CD.
- Let H be the midpoint of side DA.
Step 3: Apply the Midpoint Theorem
According to the midpoint theorem:
- In triangle ABC, segment EF (connecting midpoints E and F) is parallel to side AC and EF=12AC.
- In triangle ADC, segment HG (connecting midpoints H and G) is parallel to side AC and HG=12AC.
Step 4: Establish Parallelism
Since both segments EF and HG are parallel to AC:
- EF∥HG
Step 5: Show Equal Lengths
Since EF and HG are both half the length of AC:
- EF=HG
Step 6: Conclude that Opposite Sides are Equal and Parallel
Now, we have:
- EF∥HG and EF=HG
Step 7: Repeat for the Other Pair of Sides
Now consider triangles ABD and BCD:
- In triangle ABD, segment EH (connecting midpoints E and H) is parallel to side BD and EH=12BD.
- In triangle BCD, segment FG (connecting midpoints F and G) is parallel to side BD and FG=12BD.
Thus, we have:
- EH∥FG
- EH=FG
Step 8: Final Conclusion
Now we have established that:
- EF∥HG and EF=HG
- EH∥FG and EH=FG
Since both pairs of opposite sides are equal and parallel, we conclude that quadrilateral EFGH is a parallelogram.
Final Statement
Therefore, the quadrilateral formed by the midpoints of the sides of the quadrilateral ABCD is a parallelogram.
---
Let the vertices of the quadrilateral be A,B,C,D.
Identify the midpoints of the sides:
- Let E be the midpoint of side AB.
- Let F be the midpoint of side BC.
- Let G be the midpoint of side CD.
- Let H be the midpoint of side DA.
According to the midpoint theorem:
- In triangle ABC, segment EF (connecting midpoints E and F) is parallel to side AC and EF=12AC.
- In triangle ADC, segment HG (connecting midpoints H and G) is parallel to side AC and HG=12AC.
Since both segments EF and HG are parallel to AC:
- EF∥HG
Since EF and HG are both half the length of AC:
- EF=HG
Now, we have:
- EF∥HG and EF=HG
Now consider triangles ABD and BCD:
- In triangle ABD, segment EH (connecting midpoints E and H) is parallel to side BD and EH=12BD.
- In triangle BCD, segment FG (connecting midpoints F and G) is parallel to side BD and FG=12BD.
- EH∥FG
- EH=FG
Now we have established that:
- EF∥HG and EF=HG
- EH∥FG and EH=FG
Therefore, the quadrilateral formed by the midpoints of the sides of the quadrilateral ABCD is a parallelogram.
Topper's Solved these Questions
- VECTORBook:FIITJEEChapter:VECTORExercise:EXERCISE 2
Explore 2 Videos
- VECTORBook:FIITJEEChapter:VECTORExercise:EXERCISE 3
Explore 3 Videos
- VECTORBook:FIITJEEChapter:VECTORExercise:SOLVED PROBLEMS (OBJECTIVE)
Explore 32 Videos
- TRIGONOMETIC EQUATIONS Book:FIITJEEChapter:TRIGONOMETIC EQUATIONS Exercise:NUMERICAL BASED
Explore 3 Videos
Similar Questions
Knowledge Check
Which of the following quadrilateral is not a parallelogram ?
ARectangleBSquareCRhombusDNone of these
If each diagonals of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View SolutionProve that the quadrilateral formed by joining the mid-points of the pairs of consecutive sides of a quadrilateral is a parallelogram.
View SolutionIf the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram, so formed will be half of the area of the given quadrilateral (figure).
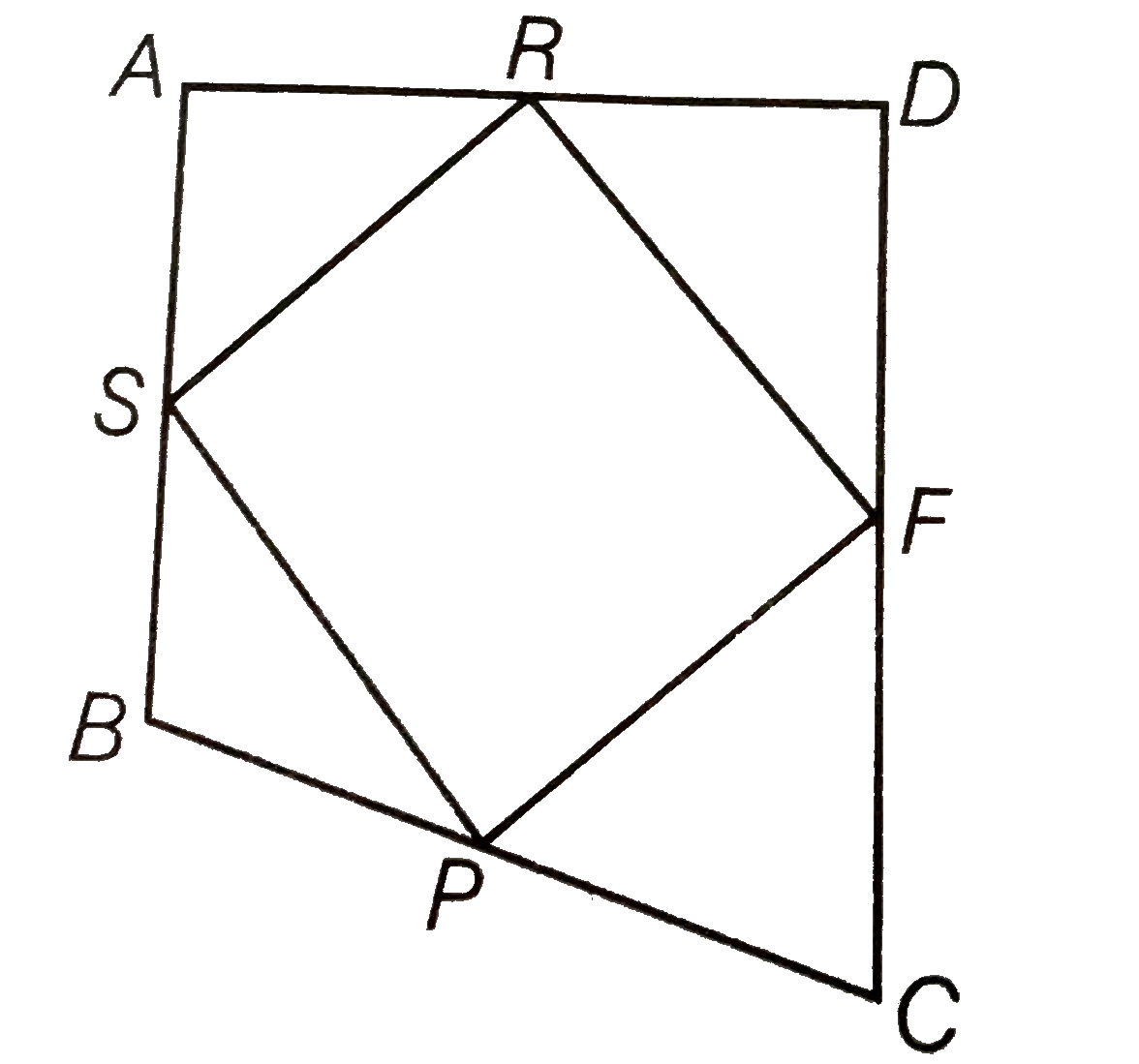
View SolutionIf the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
View SolutionIf the diagonals of a quadrilateral bisect each other at right angle, then the quadrilateral is a parallelogram (b) rectangle (c) rhombus (d) kite
View SolutionThe vertices of a quadrilateral are at (−2,4), (1,5), (4,3) and (1,2). Show that this quadrilateral is a parallelogram
View SolutionGiven the following statements :
A:If the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
B: If the diagonals of a quadrilateral do not bisect each other, then the quadrilateral is not a parallelogram.
Identify these as contrapositive or converse of each other.
View Solution