A charge 'Q' is placed at the centre of a hemispherical surface of radius 'R'. The flux of electric field due to charge 'Q' through the surface of hemisphere is
Q/4ε0
Q/4πε0
Q/2ε0
Q/2πε0
The correct Answer is:C
To solve the problem of finding the electric flux through a hemispherical surface due to a charge Q placed at its center, we can follow these steps:
Step 1: Understand the concept of electric flux
Electric flux (Φ) through a surface is defined as the total number of electric field lines passing through that surface. Mathematically, it is given by:
Φ=∫→E⋅d→A
where →E is the electric field and d→A is the differential area vector.
Step 2: Use Gauss's Law
According to Gauss's Law, the total electric flux (Φ) through a closed surface is proportional to the charge enclosed within that surface:
Φ=Qencϵ0
where Qenc is the charge enclosed and ϵ0 is the permittivity of free space.
Step 3: Consider the entire spherical surface
If we consider a complete sphere of radius R with charge Q at its center, the total electric flux through the entire sphere would be:
Φsphere=Qϵ0
Step 4: Relate the flux through the hemisphere to the sphere
Since the hemispherical surface is half of the entire sphere, the flux through the hemispherical surface would be half of the total flux through the sphere:
Φhemisphere=12Φsphere=12(Qϵ0)
Thus, we have:
Φhemisphere=Q2ϵ0
Conclusion
The electric flux through the hemispherical surface due to the charge Q placed at its center is:
Φ=Q2ϵ0
Electric flux (Φ) through a surface is defined as the total number of electric field lines passing through that surface. Mathematically, it is given by:
Φ=∫→E⋅d→A
where →E is the electric field and d→A is the differential area vector.
According to Gauss's Law, the total electric flux (Φ) through a closed surface is proportional to the charge enclosed within that surface:
Φ=Qencϵ0
where Qenc is the charge enclosed and ϵ0 is the permittivity of free space.
If we consider a complete sphere of radius R with charge Q at its center, the total electric flux through the entire sphere would be:
Φsphere=Qϵ0
Since the hemispherical surface is half of the entire sphere, the flux through the hemispherical surface would be half of the total flux through the sphere:
Φhemisphere=12Φsphere=12(Qϵ0)
Thus, we have:
Φhemisphere=Q2ϵ0
The electric flux through the hemispherical surface due to the charge Q placed at its center is:
Φ=Q2ϵ0
Topper's Solved these Questions
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL - II
13 Videos
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - COMPREHENSION BASED (COMPREHENSION - II)
3 Videos
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE) LEVEL - II & III
15 Videos
- ELECTROMAGNETIC WAVE & WAVE OPTICSBOOK - FIITJEECHAPTER - ELECTROMAGNETIC WAVE & WAVE OPTICSEXERCISE - ASSIGNMENT PROBLEMS Objective : Level-II (MULTI CHOICE SINGLE CORRECT)
21 Videos
- ELECTROSTATICSBOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example
14 Videos
Similar Questions
A charge q is placed at the centre of an imaginary spherical surface. What will be the electric flux due to this charge through any half of the sphere.
View SolutionA charge of 8.85C is placed at the centre of a spherical Gaussian surface of radius 5cm .The electric flux through the surface ..
View Solution
Knowledge Check
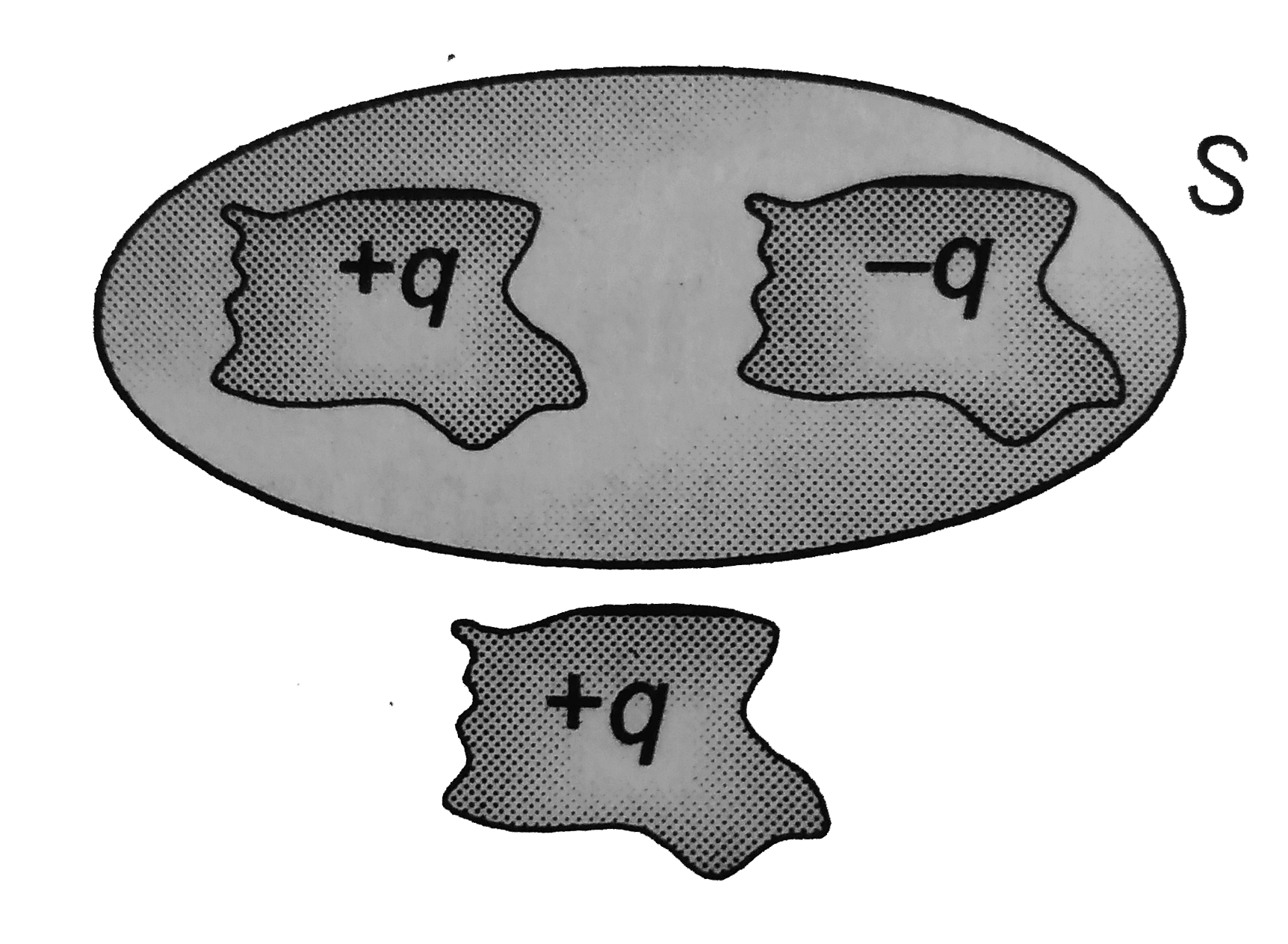
The flux of electric field due to these charges through the surface S is
 A2qε0B3qε0C−5qε0Dqε0
A2qε0B3qε0C−5qε0Dqε0A charge q is placed at the centre of the open end of a cylindrical vessel . The flux of the electric field through the surface of the vessel is
 AzeroBqε0Cq2ε0D2q2ε0.
AzeroBqε0Cq2ε0D2q2ε0.Shown below is a distribution of charges. The flux of electric field due to these charges through the surface S is
 A3qε0B2qε0Cqε0DZero
A3qε0B2qε0Cqε0DZero
A charge q is placed st the centre of a spher. Taking outward normal as positive, find the flux of the electric field through the surface of the sphere due to the enclosed charge.
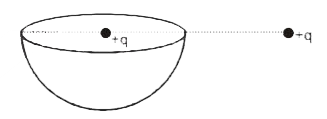
View SolutionFind flux through the hemispherical surface
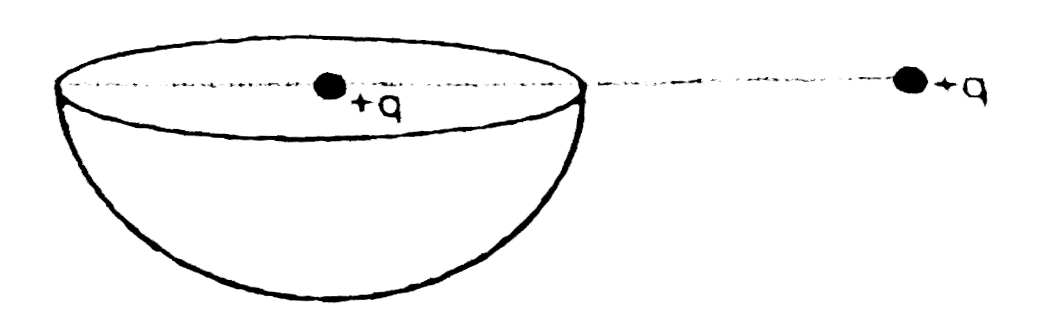
View SolutionFind flux through the hemispherical surface
View SolutionA point charge Q is placed at the centre of a hemisphere. Find the ratio of electric flux passing through curved andplane surface of the hemisphere.
View SolutionFigure shown below is a distribution of charges. The flux of electric field due to these charges through the surface S is
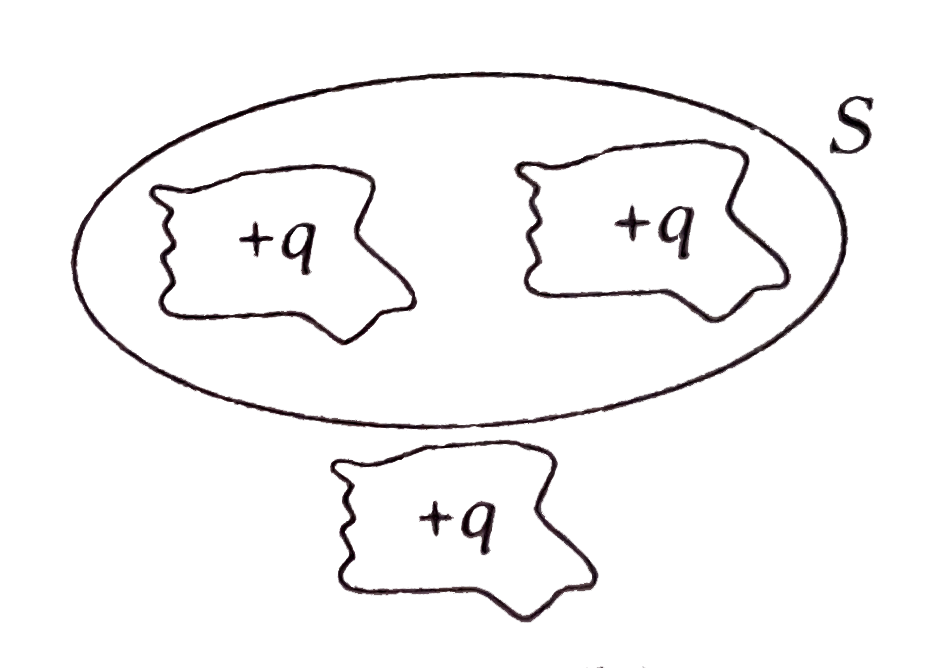
View Solution
