A particle of mass 100 gm and charge 2μC is released from a distance of 50 cm from a fixed charge of 5μC. Find the speed of the particle when its distance from the fixed charge becomes 3 m.
To solve the problem step-by-step, we will apply the principle of conservation of energy. Here’s how we can approach the question:
Given Data:
- Mass of the particle, m=100g=0.1kg
- Charge of the particle, q2=2μC=2×10−6C
- Fixed charge, q1=5μC=5×10−6C
- Initial distance from the fixed charge, r1=50cm=0.5m
- Final distance from the fixed charge, r2=3m
Step 1: Calculate the initial potential energy (PE1)
The potential energy between two point charges is given by the formula:
PE=kq1q2r
where k=14πϵ0≈9×109N m2/C2.
Calculating PE1:
PE1=kq1q2r1=9×109×(5×10−6)(2×10−6)0.5
PE1=9×109×10×10−120.5=9×109×20×10−12=180×10−3=0.18J
Step 2: Calculate the final potential energy (PE2)
Calculating PE2:
PE2=kq1q2r2=9×109×(5×10−6)(2×10−6)3
PE2=9×109×10×10−123=9×109×103×10−12=30×10−3=0.03J
Step 3: Apply conservation of energy
According to the conservation of energy:
PE1+KE1=PE2+KE2
Initially, the particle is at rest, so KE1=0:
PE1=PE2+KE2
0.18=0.03+12mv2
0.18−0.03=12mv2
0.15=12(0.1)v2
0.15=0.05v2
v2=0.150.05=3
v=√3≈1.732m/s
Final Answer:
The speed of the particle when its distance from the fixed charge becomes 3 m is approximately 1.732m/s.
---
- Mass of the particle, m=100g=0.1kg
- Charge of the particle, q2=2μC=2×10−6C
- Fixed charge, q1=5μC=5×10−6C
- Initial distance from the fixed charge, r1=50cm=0.5m
- Final distance from the fixed charge, r2=3m
The potential energy between two point charges is given by the formula:
PE=kq1q2r
where k=14πϵ0≈9×109N m2/C2.
PE1=kq1q2r1=9×109×(5×10−6)(2×10−6)0.5
PE1=9×109×10×10−120.5=9×109×20×10−12=180×10−3=0.18J
Calculating PE2:
PE2=kq1q2r2=9×109×(5×10−6)(2×10−6)3
PE2=9×109×10×10−123=9×109×103×10−12=30×10−3=0.03J
According to the conservation of energy:
PE1+KE1=PE2+KE2
Initially, the particle is at rest, so KE1=0:
PE1=PE2+KE2
0.18=0.03+12mv2
0.18−0.03=12mv2
0.15=12(0.1)v2
0.15=0.05v2
v2=0.150.05=3
v=√3≈1.732m/s
The speed of the particle when its distance from the fixed charge becomes 3 m is approximately 1.732m/s.
Topper's Solved these Questions
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - MATRIX MATCH TYPE
1 Videos
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - ASSERTION REASONING TYPE
1 Videos
- ELECTROMAGNETIC WAVE & WAVE OPTICSBOOK - FIITJEECHAPTER - ELECTROMAGNETIC WAVE & WAVE OPTICSEXERCISE - ASSIGNMENT PROBLEMS Objective : Level-II (MULTI CHOICE SINGLE CORRECT)
21 Videos
- ELECTROSTATICSBOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example
14 Videos
Similar Questions
Two identical charges, 5μC each are fixed at a distance 8 cm and a charged particle of mass 9×10−6kg and charge −10μC is placed at a distance 5 cm from each of them and is released. Find the speed of the particle when it is nearest to the two charges.
View SolutionA point particle of mass m is released from a distance √3R along the axis of a fixed ring of mass M and radius R as shown in figure. Find the speed of particle as it passes through the centre of the ring.

View Solution
Knowledge Check
A particle of mass 2 g and charge 1μC is held at rest on a frictionless surface at a distance of 1m from a fixed charge of 1 mC. If the particle is released it will be repelled. The speed of the particle when it is at distance of 10 m from fixed charge is :
A100 m/sB90 m/sC60 m/sD45 m/sA charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
A2E2t2mqBE2q2t22mCEq2m2t2DEqm2tA charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
A2E2t2mqBE2q2r22mCEq2m2t2DEqm2t
Two point charges, each of mass m and charge q are released when they are at a distance from each other. What is the speed of each charged particle when they are at a distance 2r ?
View SolutionA long straight wire carries a charge with linear density λ. A particle of mass m and a charge q is released at a distance r from the the wire. The speed of the particle as it crosses a point distance 2r is
View SolutionTwo circular rings A and B each of radius a = 30 cm are placed coaxially with their axis horizontal in a uniform electric field E=105NC−1 directed vertically upward as shown in figure. Distance between centers of the rings A and B (CAandCB)is40cm. Ring A has positive charge qA=10μC and B has a negative charge qB=−20μC. A particle of mass m and charge q=10μC is released from rest at the center of ring A. If particle moves along CACB, then
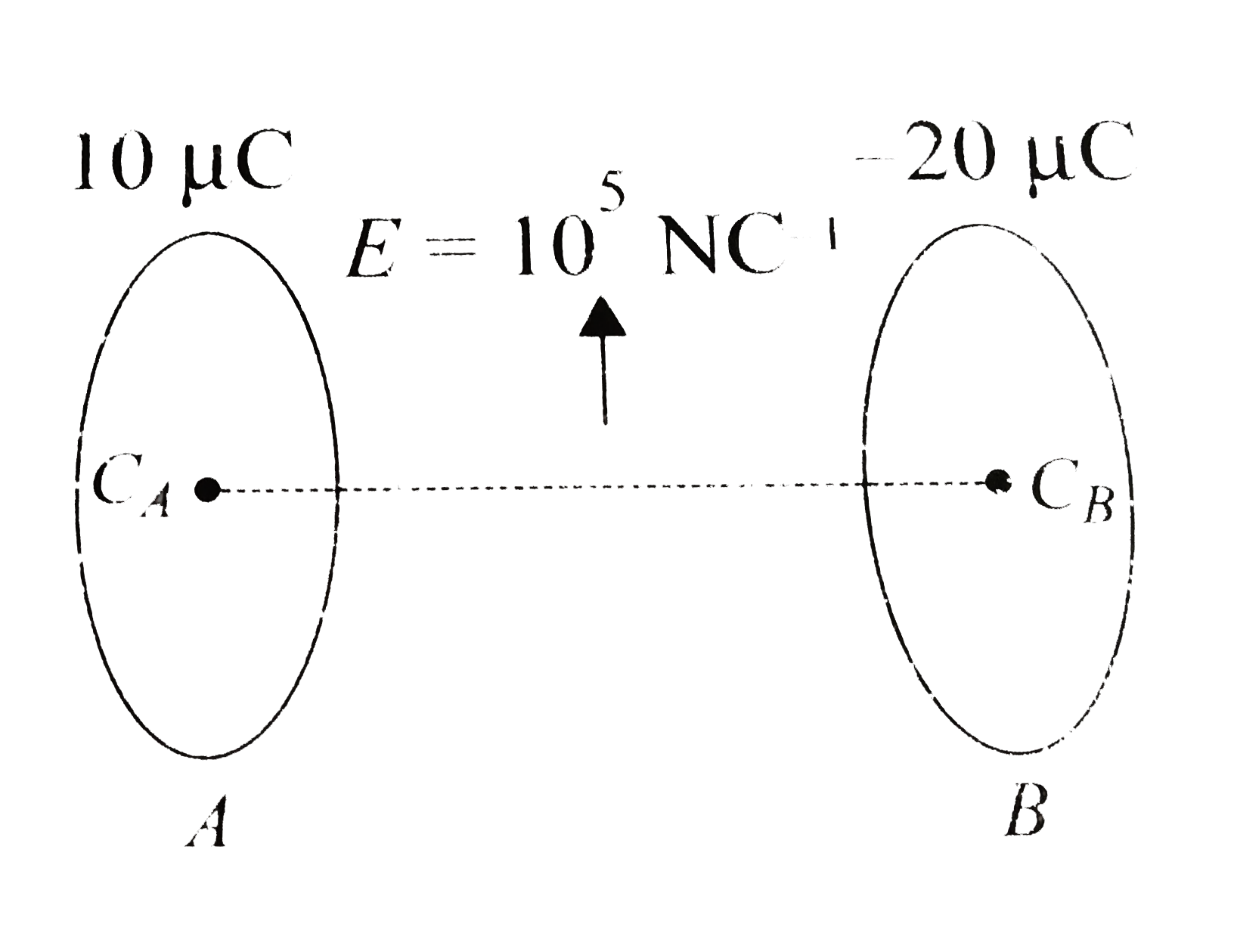
Speed of particle when it reaches at center of B is
View SolutionA charged particle of charge 'Q' is held fixed and another charged particle of mass 'm' and charge 'q' (of the same sign) is released from a distance 'r'. The impulse of the force exerted by the external agent on the fixed charge by the time distance between 'Q' and 'q' becomes 2r is
View SolutionTwo point charges q1=q2=2μC are fixed at x1=+3m and x2=−3m as shown in figure. A third particle of mass 1 g and charge q3=−4μC is released from rest at y=4.0m.Find the speed of the particle as it reaches the origin.
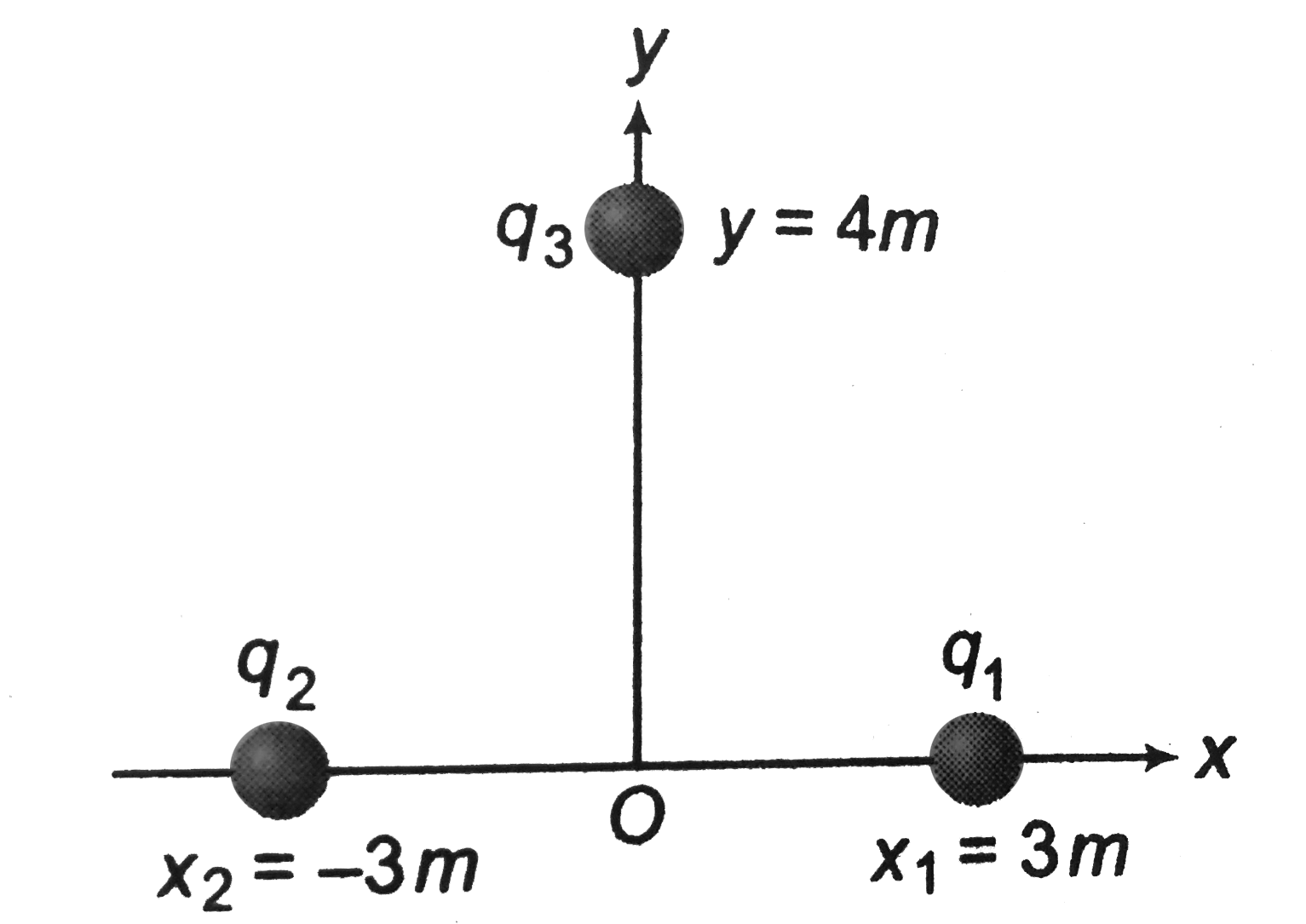
View Solution
