The function f:R→R,f(x)=x2 is
injective but not surjective
surjective but not injective
injective as well as surjective
neither injective nor surjective.
The correct Answer is:D
To determine the properties of the function f:R→R defined by f(x)=x2, we need to check if it is injective (one-to-one) and/or surjective (onto).
Step 1: Check if the function is injective
A function is injective if different inputs map to different outputs. In other words, if f(a)=f(b) implies that a=b.
Let’s assume f(a)=f(b):
a2=b2
This implies:
a=bora=−b
Since a can be equal to b or the negative of b, we can find different inputs that yield the same output (for example, f(1)=1 and f(−1)=1). Therefore, the function is not injective.
Step 2: Check if the function is surjective
A function is surjective if for every element y in the codomain R, there exists at least one x in the domain R such that f(x)=y.
Let’s consider y=−4:
f(x)=−4⟹x2=−4
This equation has no real solutions since the square of a real number cannot be negative. Therefore, there are values in R (like -4) that are not achieved by f(x). Thus, the function is not surjective.
Conclusion
Since the function f(x)=x2 is neither injective nor surjective, the final answer is that the function is neither injective nor surjective.
Final Answer
The function f(x)=x2 is neither injective nor surjective.
A function is injective if different inputs map to different outputs. In other words, if f(a)=f(b) implies that a=b.
a2=b2
This implies:
a=bora=−b
Since a can be equal to b or the negative of b, we can find different inputs that yield the same output (for example, f(1)=1 and f(−1)=1). Therefore, the function is not injective.
A function is surjective if for every element y in the codomain R, there exists at least one x in the domain R such that f(x)=y.
f(x)=−4⟹x2=−4
This equation has no real solutions since the square of a real number cannot be negative. Therefore, there are values in R (like -4) that are not achieved by f(x). Thus, the function is not surjective.
Since the function f(x)=x2 is neither injective nor surjective, the final answer is that the function is neither injective nor surjective.
The function f(x)=x2 is neither injective nor surjective.
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - TIPSEXERCISE - ASSERTION REASONING8videos
TIPS
- BOOK - FIITJEECHAPTER - TIPSEXERCISE - MCQ (MULTIPLE CORRECT)37videos
TIPS
- BOOK - FIITJEECHAPTER - TIPSEXERCISE - ASSIGNMENT (SECTION (I): MCQ (SINGLE CORRECT)125videos
TIPS
- BOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - MATHEMATICS328videos
TEST PAPERS
- BOOK - FIITJEECHAPTER - TRIGNOMETRIC RATIOS AND IDENTITIESEXERCISE - All Questions1videos
TRIGNOMETRIC RATIOS AND IDENTITIES
Similar Questions
The function f:R→R,f(x)=ax2−b|x|,a,b∈R has a local minimum at x=0 then which of the following options must be CORRECT?
O a>0
O b<0
O |ab|+a|b|+b|a|=−ab
O |ab|+a|b|=b|a|+abView SolutionDefine the function f : R →R by y = f(x) = x2, x∈R. Complete the Table given below by using this definition. What is the domain and range of this function?
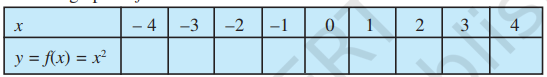 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Function is said to be onto if range is same as co - domain otherwise it is into. Function is said to be one - one if for all x1≠x2⇒f(x1)≠f(x2) otherwise it is many one.
Function f:R→R,f(x)=x2+x, isAone - one ontoBone - one intoCmany one ontoDmany one into - Question 2 - Select One
consider the function f:R→R,f(x)=x2−6x+4x2+2x+4
f(x) isAunbounded functionBone one functionConto functionDnone of these - Question 3 - Select One
consider the function f:R→R,f(x)=x2−6x+4x2+2x+4
Range of fX() isA(−∞−23]∪[2,0)B[−13,5]C(−∞,2)∪[73,∞)D(20,∞)
Function f:R→R,f(x)=x|x| is
View SolutionThe function f:R→R satisfies f(x2).fx=f′(x).f′(x2) for all real x. Given that f(1)=1 and f1=8 , then the value of f′(1)+f1 is 2 b. 4 c. 6 d. 8
View SolutionIf f(x)=1x2+x+1, then the function f:R→R is
View Solutionconsider the function f:R→R,f(x)=x2−6x+4x2+2x+4
which of the following is not true about f(x)?View SolutionThe function f:R→R defined as f(x)=x2−x+1x2+x+1 is
View Solution

