If the determinant ∣∣ ∣ ∣∣x+ap+ul+fy+bq+vm+gz+cr+wn+h∣∣ ∣ ∣∣ splits into exactly k determinants of order 3, each element of which contains onlyone term, then the value of k is 8.
The correct Answer is:N/a
True
Since ∣∣
∣
∣∣x+ap+ul+fy+bq+vm+gz+cr+wn+h∣∣
∣
∣∣
=∣∣
∣
∣∣xply+bq+vm+gz+cr+wn+h∣∣
∣
∣∣+∣∣
∣
∣∣aufy+bq+vm+gz+cr+wn+h∣∣
∣
∣∣ [splitting first row]
=∣∣
∣
∣∣xplyqmz+cr+mn+h∣∣
∣
∣∣+∣∣
∣
∣∣xplbvgz+cr+wn+h∣∣
∣
∣∣
+∣∣
∣
∣∣aufyqmz+cr+wn+h∣∣
∣
∣∣+∣∣
∣
∣∣aufbvgz+cr+wn+h∣∣
∣
∣∣ [splitting second row]
Similarly we can split these 4 determinants in 8 determinantes by splitting each one in two determinants further. So, given statement is true.
Topper's Solved these Questions
Similar Questions
If the determinant |a+pl+xu+fb+qx+yv+gc+rn+zw+h| splits into exactly K determinants of order 3, each element of which contains only one term, then the values of K is
View SolutionGiven question are based on English alphabet.
A B C D E F G H I J K L M N O P Q R S T U V W X Y Z
If the letters from T to Z are interchanged by the letters A to G in such a way that A takes the position of T and so on, then which will be the third letter to the left of 18th letter from right?
1) Y
2) U
3) B
4) C
5) None of theseView Solution
Knowledge Check
- Question 1 - Select One
Each question is based on the following alphabet-series :
ABCDEFGHIJKLMNOPQRSTUVWXYZ
ABCDEFGH
Which letter is second to the left of the letter immediately to the left of the letter which is fourth to the right of the letter immediately to the left of the letter which is second to the left of the letter D?AABBCCDD - Question 2 - Select One
If ∣∣ ∣ ∣∣abclmnpqr∣∣ ∣ ∣∣=2, then what is the value of the determinant ∣∣ ∣ ∣∣6a3b15c2lm5n2pq5r∣∣ ∣ ∣∣ = ?
A10B20C40D60 - Question 3 - Select One
If∣∣ ∣ ∣∣abclmnpqr∣∣ ∣ ∣∣=2,then what is the value of the determinant∣∣ ∣ ∣∣6a3b15c2lm5n2pq5r∣∣ ∣ ∣∣?
A10B20C40D60
This question is based on the following alphabet series.
A B C D E F G H I J K L M N O P Q R S T U V W X Y Z
If the letters from T to Z are interchanged by the letters A to G in such a way that A takes the position of T and so on, then which will be the third letter to the left of 18th letter from the right?
1) Y
2) U
3) B
4) C
5) None of theseView SolutionBelow are given lettes A to Z . Under each capital letter a small letter is written which is to be used as a code for the capital letter :
ABCDEFGHIJKLMjnirixavesoydNOPQRSTUVWXYZqmtguczwhpkbf
in each of the following questions a group of six capital letters is given and its code equivalent is given is one of the columns (1), (2), (3) or (4) . Study the group of letters given in each question and with the help of code given above, choose the code equivalent from amongst (1),(2),(3) or (4) as your answer
HITREH
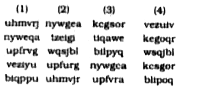 View Solution
View SolutionEach question is based on the following alphabet-series :
ABCDEFGHIJKLMNOPQRSTUVWXYZ
Which letter will be sixth to the right of the third letter from the left of the letter which is exactly in the middle of the letters in the series given below ?
ABCDEZYXWVQRSTUFGHIJKLMNOPAView SolutionStudy the following information carefully to answee these questions.
M K K I D N E T T Q O B F H A A G T U U X W L S R I
Each of the letters gets a numerical value based on its position in the above arrangement, such as, 1 for M, 2 for K, 4 for I and so on.
Value of A is exactly equal to the total value of which of the following pairs ?
(A) DO (B) QE (C) MHView SolutionEach of the following question is based on the following alphabet series :
A B C D E F G H I J K L M
N O P Q R S T U V W X Y Z
K L M N O P Q R S
Which letter is fourth to the right of the letter immediately to the left of the letter immediately to the left of the letter which is third to the right of the letter immediately to the left of the letter L ?View Solution

