The curve which represents a quadratic polynomial meets the x axis at (2,0) and (-2,0). Form the quadratic polynomial.

Video Solution

Answer

Step by step video & image solution for The curve which represents a quadratic polynomial meets the x axis at (2,0) and (-2,0). Form the quadratic polynomial. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS REAL NUMBERS

Knowledge Check

Which of the following is a quadratic polynomial ?
A $x + 4$
B $x^{3} + x$
C $x^{3} + 2 x + 6$
D $x^{2} + 5 x + 4$
The sum of zeroes of quadratic polynomial is $- \sqrt{2}$ and product is 7, that quadratic polynomial is :
A $x^{2} - \sqrt{2} x + 7$
B $x^{2} + \sqrt{2} x + 7$
C $x^{2} - \sqrt{2} x - 7$
D $x^{2} + \sqrt{2} x - 7$
A quadratic polynomial with zeroes -2 and 3, is :
A $3 x^{2} - 2 x + 6$
B $2 x^{2} + 3 x - 6$
C $x^{2} - 2 x + 6$
D $x^{2} - x - 6$

Form a quadratic polynomial whose zeros are $5$ and $4$
View Solution
Problems on Quadratic Polynomial-part 2
View Solution
.

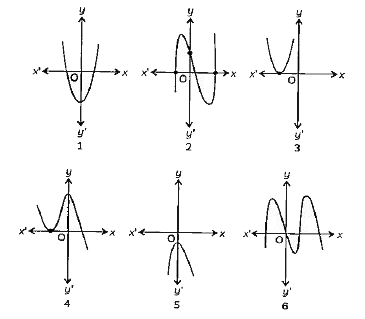
Which of the above graph represent quadratic polynomials?
View Solution
Graphs of quadratic polynomial and their application, Problems related to sign of quadratic polynomial
View Solution
Form a quadratic polynomial whose zeroes are

$\frac{3}{5} and - \frac{1}{2}$
View Solution

The curve which represents a quadratic polynomial meets the x axis at (2,0) and (-2,0). Form the quadratic polynomial.

Similar Questions

Knowledge Check

Which of the following is a quadratic polynomial ?

The sum of zeroes of quadratic polynomial is $- \sqrt{2}$ and product is 7, that quadratic polynomial is :

A quadratic polynomial with zeroes -2 and 3, is :

Recommended Questions

The curve which represents a quadratic polynomial meets the x axis at (2,0) and (-2,0). Form the quadratic polynomial.

Similar Questions

Knowledge Check

Which of the following is a quadratic polynomial ?

The sum of zeroes of quadratic polynomial is −√2 and product is 7, that quadratic polynomial is :

A quadratic polynomial with zeroes -2 and 3, is :

Recommended Questions

The sum of zeroes of quadratic polynomial is $- \sqrt{2}$ and product is 7, that quadratic polynomial is :