If angle of repose is ϕ, the coefficient of static friction between body and the plane is
cotϕ
tanϕ
sinϕ
cosϕ
To find the coefficient of static friction (μ) between a body and an inclined plane at the angle of repose (φ), we can follow these steps:
1. Understanding the Forces:
- When a body is placed on an inclined plane, the gravitational force (mg) acts downwards. This force can be resolved into two components:
- Perpendicular to the plane: mgcosθ
- Parallel to the plane: mgsinθ
2. Normal Force Calculation:
- The normal force (N) acting on the body is equal to the perpendicular component of the gravitational force:
N=mgcosθ
3. Frictional Force:
- The maximum static frictional force (f_s) that can act on the body is given by:
fs=μN=μ(mgcosθ)
4. Condition for Motion:
- The angle of repose is defined as the angle at which the body is about to slide down the plane. At this point, the force due to gravity parallel to the plane equals the maximum static frictional force:
mgsinθ=μ(mgcosθ)
5. Simplifying the Equation:
- We can cancel mg from both sides (assuming mg≠0):
sinθ=μcosθ
6. Finding the Coefficient of Static Friction:
- Rearranging the equation gives:
μ=sinθcosθ=tanθ
7. Relating to the Angle of Repose:
- The angle of repose (φ) is defined such that:
θ=ϕ
- Therefore, we can substitute φ into our equation:
μ=tanϕ
Final Answer:
The coefficient of static friction (μ) between the body and the inclined plane is:
μ=tanϕ
- When a body is placed on an inclined plane, the gravitational force (mg) acts downwards. This force can be resolved into two components:
- Perpendicular to the plane: mgcosθ
- Parallel to the plane: mgsinθ
- The normal force (N) acting on the body is equal to the perpendicular component of the gravitational force:
N=mgcosθ
- The maximum static frictional force (f_s) that can act on the body is given by:
fs=μN=μ(mgcosθ)
- The angle of repose is defined as the angle at which the body is about to slide down the plane. At this point, the force due to gravity parallel to the plane equals the maximum static frictional force:
mgsinθ=μ(mgcosθ)
- We can cancel mg from both sides (assuming mg≠0):
sinθ=μcosθ
- Rearranging the equation gives:
μ=sinθcosθ=tanθ
- The angle of repose (φ) is defined such that:
θ=ϕ
- Therefore, we can substitute φ into our equation:
μ=tanϕ
The coefficient of static friction (μ) between the body and the inclined plane is:
μ=tanϕ
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-A)65videos
LAWS OF MOTION
- BOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-B)44videos
LAWS OF MOTION
- BOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Example24videos
LAWS OF MOTION
- BOOK - AAKASH INSTITUTECHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions10videos
KINETIC THEORY
- BOOK - AAKASH INSTITUTECHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)26videos
MAGNETISM AND MATTER
Similar Questions
The coefficient of friction between the block and the inclined plane is μ.
View SolutionA body is moving down along inclined plane of angle of inclination theta The coefficient of friction between the body and the plane varies as mu=0.5x where x is the distance moved down the plane. The body will have the maximum velocity when
View Solution
Knowledge Check
- Question 1 - Select One
The coefficient of static friction between two surfaces depend on
Anature of surfaceBthe shape of the surface in contactCthe area of contactDall of the above - Question 2 - Select One
When a maximum force of 3 N is applied on a body kept on rough inclined plane of shown in the figure, then body remains stationary. The maximum external force up the inclined plane that does not move the block is 12 N. The coefficient of static friction between the block and the plane is [Take, g = 10 m/s2]
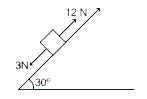 A√35B1√3C3√35D53√3
A√35B1√3C3√35D53√3 - Question 3 - Select One
A block of mass 4kg rests on a rough horizontal plane. The plane is gradually inclined until at an angle θ=60∘ , with the vertical, the mass just begins to side. What is the coefficient of static friction between the block and the plane?
A√3B12C1√2D1√3
A box is lying on an inclined plane if the box slides when the angle of inclination is 60∘ then the coefficient of static friction of the box and the plane is?
View SolutionCoefficient of static friction does not depend upon
View SolutionStatic friction between two surfaces
View SolutionA body is on a rough horizontal surface which is moving horizontally in SHM of frequency 2Hz. The coefficient of static friction between the body and the surface is 0.5. The maximum value of the amplitude for which the body will not slip along the surface is approximately
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View Solution

