What is the velocity of the highest point of a rotating wheel of a car moving with a velocity of 20 m/s ?
To find the velocity of the highest point of a rotating wheel of a car moving with a velocity of 20 m/s, we can follow these steps:
Step 1: Understand the motion of the wheel
When a car is moving forward, the wheels are also rotating. The point of contact of the wheel with the ground is momentarily at rest, while the topmost point of the wheel is moving forward with the car's velocity plus the additional velocity due to the wheel's rotation.
Step 2: Determine the velocity of the wheel's center
The velocity of the center of the wheel (which is also the velocity of the car) is given as 20 m/s.
Step 3: Calculate the additional velocity at the highest point
The highest point of the wheel moves in the same direction as the car. The rotational motion of the wheel adds to the translational motion of the car. The additional velocity at the highest point due to rotation is equal to the linear velocity of the wheel, which is also 20 m/s.
Step 4: Combine the velocities
To find the total velocity of the highest point of the wheel, we add the translational velocity of the car (20 m/s) to the rotational velocity at the highest point (20 m/s):
Total Velocity=Velocity of the center+Velocity due to rotation
Total Velocity=20m/s+20m/s=40m/s
Step 5: Conclusion
The velocity of the highest point of the rotating wheel is 40 m/s.
---
When a car is moving forward, the wheels are also rotating. The point of contact of the wheel with the ground is momentarily at rest, while the topmost point of the wheel is moving forward with the car's velocity plus the additional velocity due to the wheel's rotation.
The velocity of the center of the wheel (which is also the velocity of the car) is given as 20 m/s.
The highest point of the wheel moves in the same direction as the car. The rotational motion of the wheel adds to the translational motion of the car. The additional velocity at the highest point due to rotation is equal to the linear velocity of the wheel, which is also 20 m/s.
To find the total velocity of the highest point of the wheel, we add the translational velocity of the car (20 m/s) to the rotational velocity at the highest point (20 m/s):
Total Velocity=20m/s+20m/s=40m/s
The velocity of the highest point of the rotating wheel is 40 m/s.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - J) Aakash Challengers Questions
13 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTECHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTECHAPTER - TEST 1EXERCISE - EXERCISE
9 Videos
Similar Questions
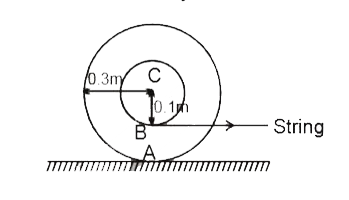
A wheel is made to roll without slipping, towards right, by pulling a string wrapped around a coaxial spool as shown in figure. With what velocity the string should be pulled so that the centre of the wheel moves with a velocity of 3m/s ?
View SolutionTwo particles move simultaneously from two points A and B, 300m apart. The particle at A, starts towards B with a velocity of 25 m/s and that at B, moves normal to the former with a velocity of 20 m/s
View Solution
Knowledge Check
A motor car A is travelling with a velocity of 20m/s in the north-west direction and another motor car B is travelling with a velocity of 15m/s in the north-east directions.The magnitude of relative velocity of B with respect to A is.
A25m/sB15m/sC20m/sD35m/sA car is going east wards with a velocity of 8m/s. To the passengers in the car, a train appears to be moving north wards with a velocity of 15 m/s. What is the actual velocity of the train:
A7 m/sB17 m/sC23 m/sDNone of theseTwo cars move in the same direction along parallel roads. One of them is a 200m, long travelling with a velocity of 20m/s. The second one is 800m long travelling with a velocity of 7.5m/s. How long will it take for the first car to overtake the second car?
A20sB40sC60sD80s
A car of mass one metric ton travelling at 32m/sc dashes into the rear of a truck of mass 8000kg moving in the same direction with a velocity of 4m/s After the collision the car bounces back wards with a velocity of 8m/s What is the velocity of the truck after the impact ?
View SolutionWhat will be the minimum stopping distance required by a car to stop if it is moving with a velocity of 40m/s with a deceleration of 20m/s3? How much time will it take to stop the car?
View SolutionA man going east in a car with a velocity of 20 km/hr, a train appears to him to move towards north with a velocity of 20√3 km/hr. What is the actual velocity and direction of motion of train?
View SolutionA car is travelling along a straight line and covers one-fourth of the total distance with a velocity of 10m/s. the remaining distance is covered with a velocity of 5m/s. calculate the average velocity of the car.
View SolutionA car travels half the distance with a constant velocity of 40m/s and the remaining half with a constant velocity of 60m/s. The average velocity of the car in m/s is
View Solution