Explain the step-deviation method of calculating arithmetic mean, taking an imaginary set of data.
Video Solution
|
Answer
Step by step video & image solution for Explain the step-deviation method of calculating arithmetic mean, taking an imaginary set of data. by Economics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCY - ARITHMETIC MEANBOOK - TARUN JAIN & VK OHRICHAPTER - MEASURES OF CENTRAL TENDENCY - ARITHMETIC MEANEXERCISE - 4. Essential Practicals
46 Videos
- MEASURES OF CENTRAL TENDENCY - ARITHMETIC MEANBOOK - TARUN JAIN & VK OHRICHAPTER - MEASURES OF CENTRAL TENDENCY - ARITHMETIC MEANEXERCISE - 5. NCERT Questions (With Hints to Answers)
12 Videos
- MEASURES OF CENTRAL TENDENCY - ARITHMETIC MEANBOOK - TARUN JAIN & VK OHRICHAPTER - MEASURES OF CENTRAL TENDENCY - ARITHMETIC MEANEXERCISE - 2. Short Answer Type Questions
18 Videos
- INDEX NUMBERSBOOK - TARUN JAIN & VK OHRICHAPTER - INDEX NUMBERSEXERCISE - NCERT Questions (With Hints to Answers)
12 Videos
- MEASURES OF CENTRAL TENDENCY MEDIAN AND MODEBOOK - TARUN JAIN & VK OHRICHAPTER - MEASURES OF CENTRAL TENDENCY MEDIAN AND MODEEXERCISE - NCERT Questions (With Hints to Answers)
5 Videos
Similar Questions
Knowledge Check
The formula for the step deviation method of finding the mean is-
A¯¯¯X=∑xNB¯¯¯X=A+∑dxNC¯¯¯X=A+∑dxNXID¯¯¯X=∑fx∑fWhich is not a method to find arithmetic mean?
ADirect methodBShort-cut methodCStep-deviation methodDKarl Pearson's methodWhich is not a method to find arithmetic mean?
ADirect methodBShort-cut methodCStep-deviation methodDKarl Pearson's method
Find the mean deviation using arithmetic mean from the following table:
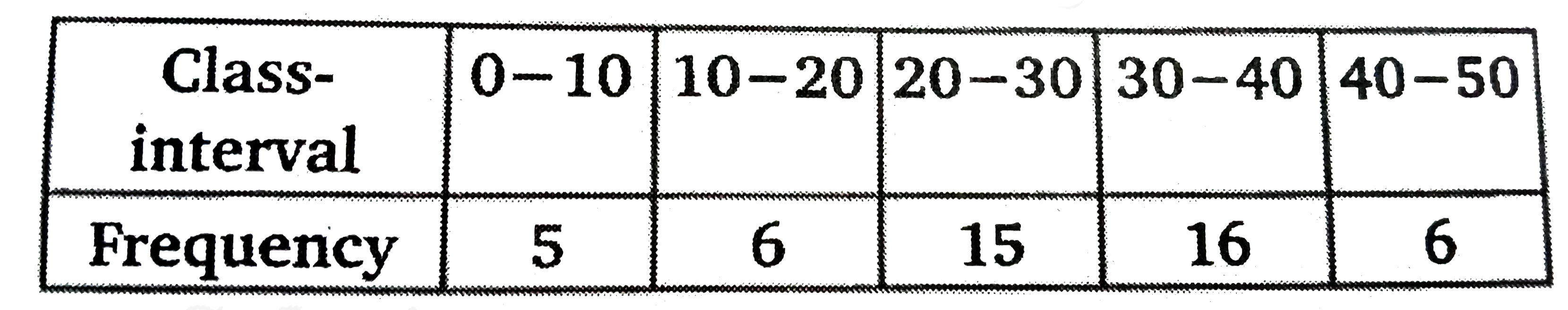
View SolutionCalculate Arithmetic mean by the "Assumed mean" method
View SolutionGive formula of calculating arithmetic mean of a continuous series using direct method.
View SolutionGive formula of calculating arithmetic mean of a continuous series using direct method.
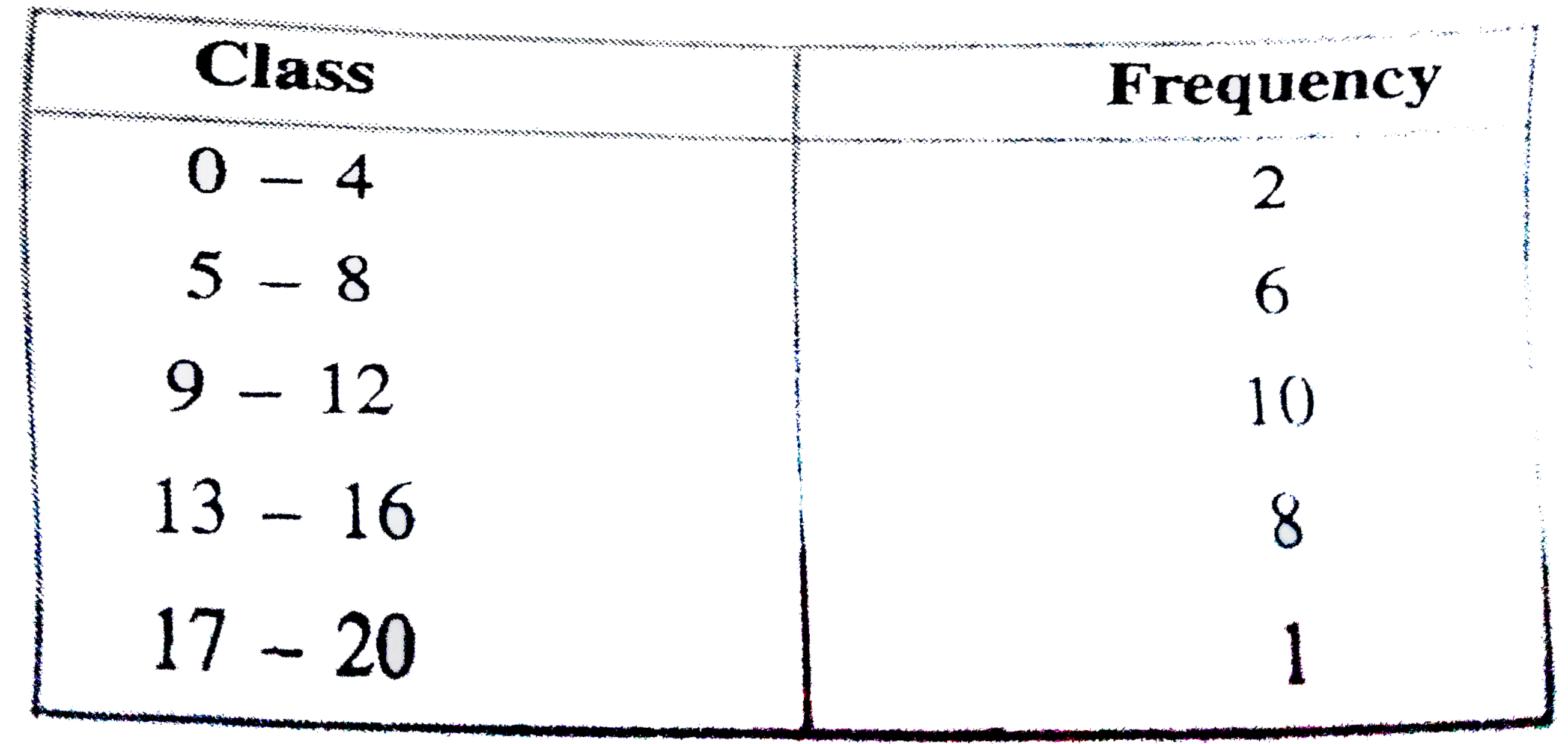
View SolutionFind the mean deviation using arithmetic mean from the folloiwng observations:
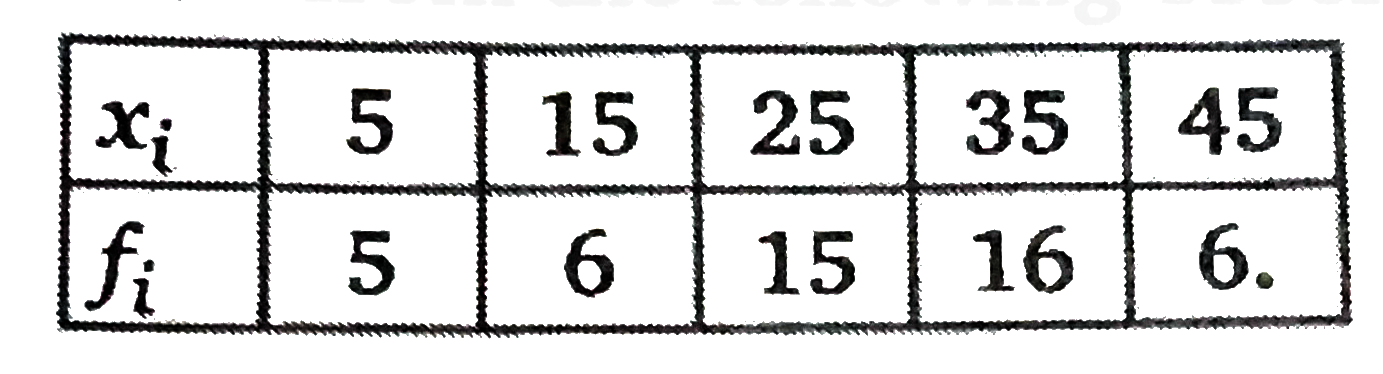
View Solution

