is the probability of finding the Is electron of hydrogen atom in a spherical shell of infitesimal thickness, dr, at a distance from the nucleus. The volume of this shell is . The qualitative sketch of the dependence of on r is
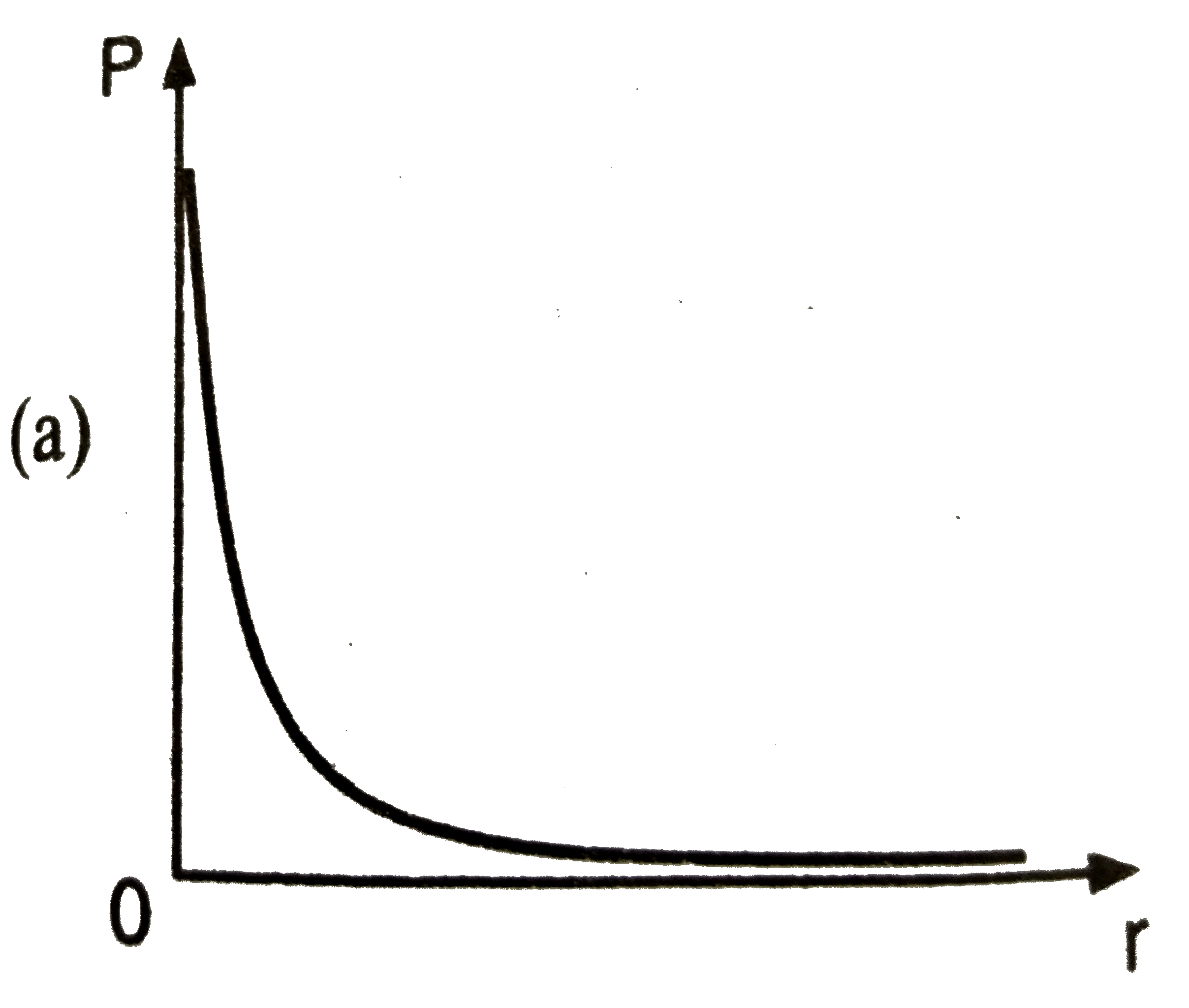
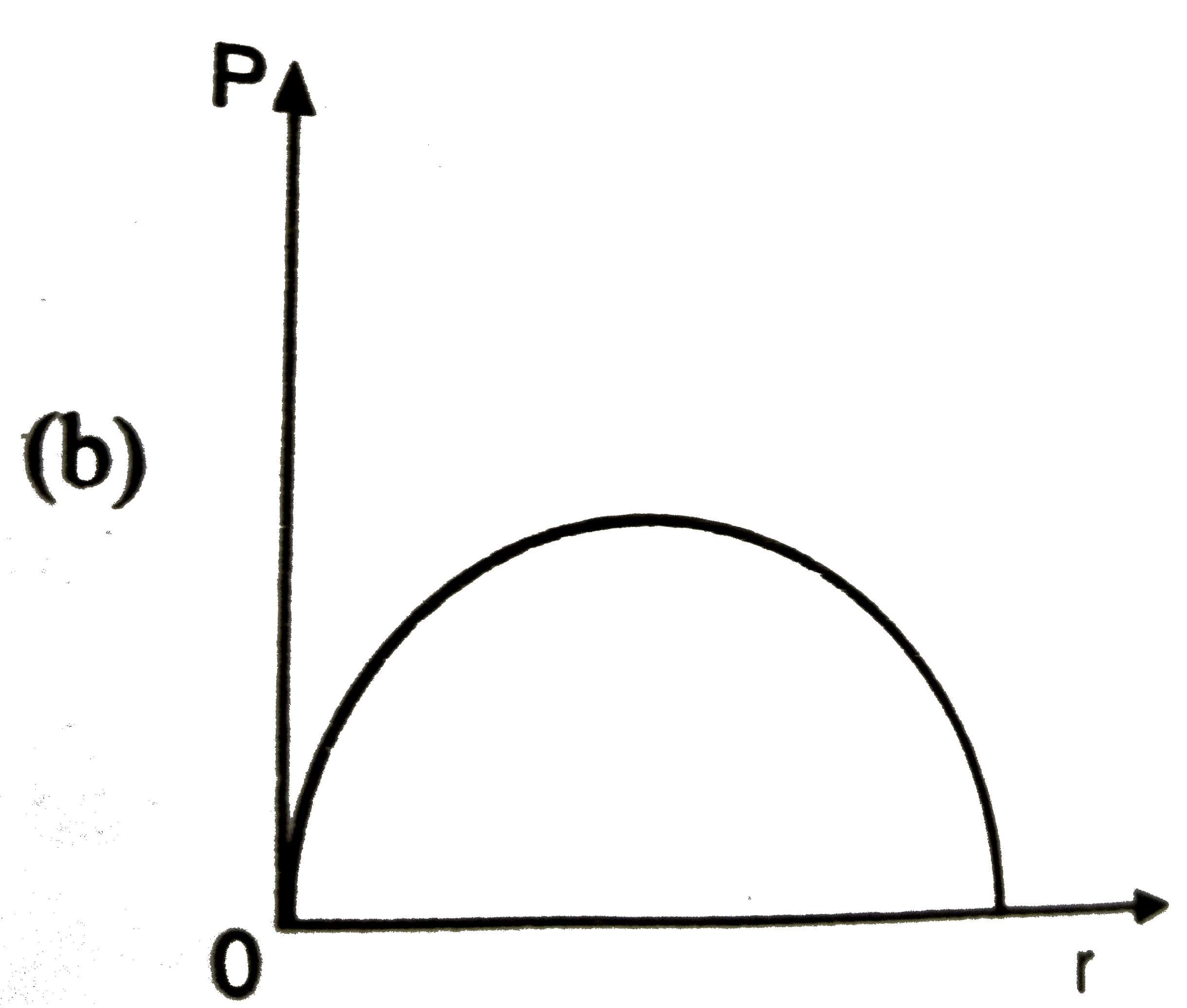
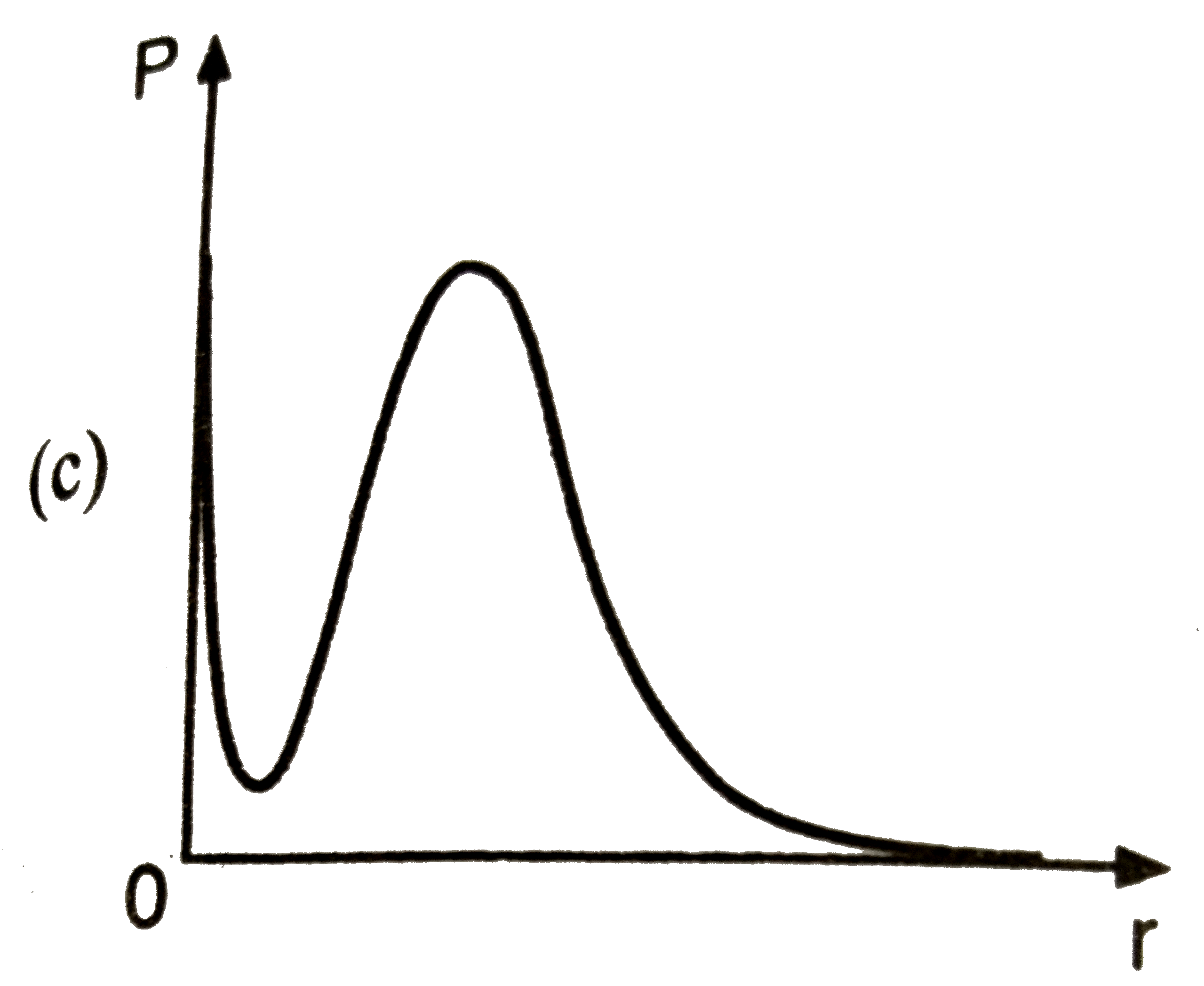
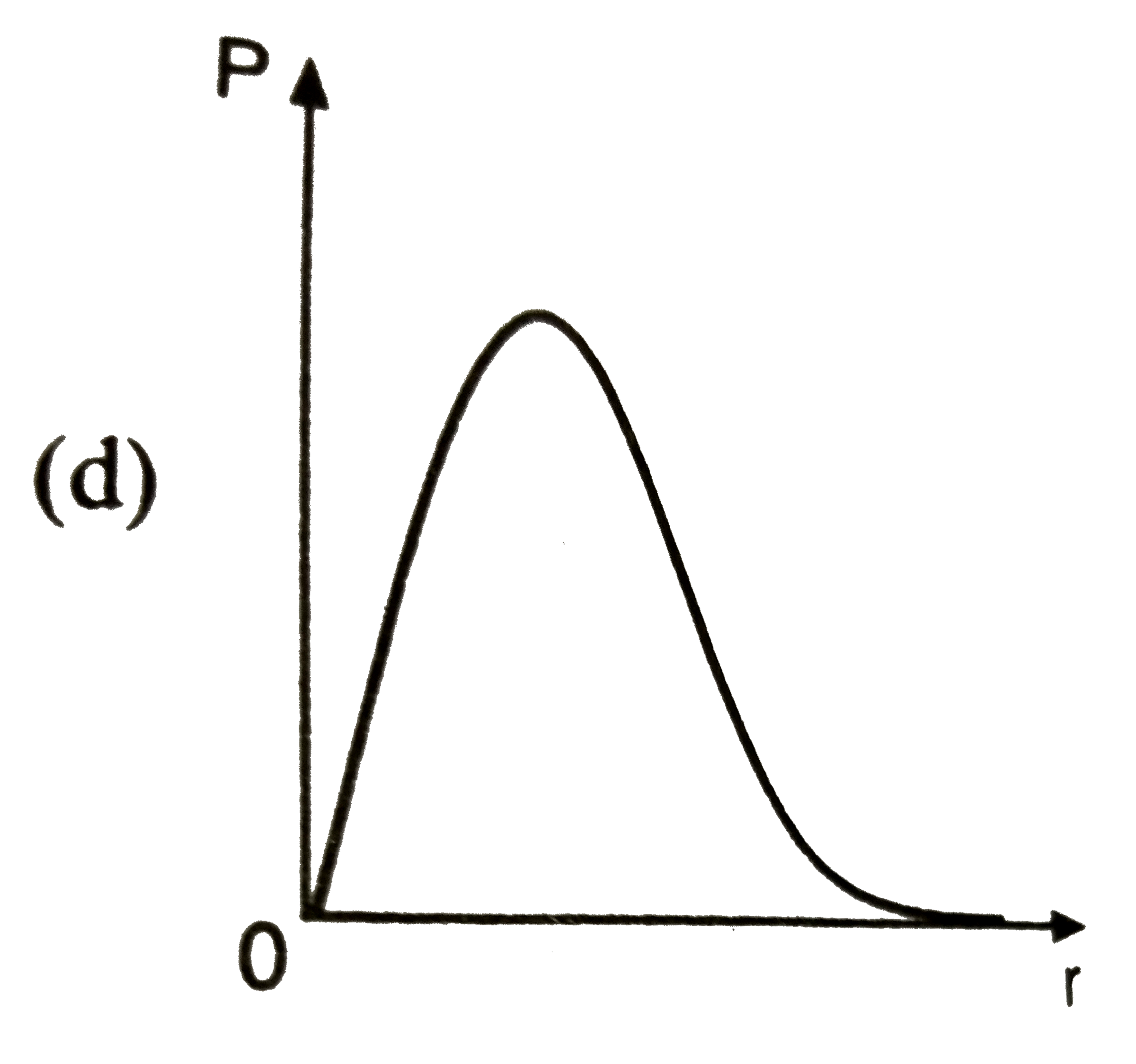
The correct Answer is:D
Radial probability function describes the total probability of finding the electron in a spherical shell of thickness 'dr' located at distance r fro0m nucleus.
R.P.F.=(volume of spherical shell)probability density
.
R.P.F. will be zero when r=0 and r=, it is represented by Graph (d).
Topper's Solved these Questions
- ATOMIC STRUCTURE BOOK - OP TANDONCHAPTER - ATOMIC STRUCTURE EXERCISE - Assertion Reason
22 Videos
- ATOMIC STRUCTURE BOOK - OP TANDONCHAPTER - ATOMIC STRUCTURE EXERCISE - Matrix matching Type Question
5 Videos
- ATOMIC STRUCTURE BOOK - OP TANDONCHAPTER - ATOMIC STRUCTURE EXERCISE - Set-2
46 Videos
- AROMATIC HYDROCARBONS (ARENES)BOOK - OP TANDONCHAPTER - AROMATIC HYDROCARBONS (ARENES)EXERCISE - EXAMPLES
24 Videos
- CHARACTERISATION OF ORGANIC COMPOUNDS BOOK - OP TANDONCHAPTER - CHARACTERISATION OF ORGANIC COMPOUNDS EXERCISE - Passage-2
5 Videos
Similar Questions
Find the gravitational potential due to a spherical shell of mass M and radius R at r<R and r>R, where r is the distance from the centre of the shell.
View SolutionP is the probability of finding the Is electron of hydrogen atom in a spherical shell of infitesimal thickness, dr, at a distance r from the nucleus. The volume of this shell is 4πr2dr. The qualitative sketch of the dependence of P on r is
View Solution
Knowledge Check
P is the probability of finding the Is electron of hydrogen atom in a spherical shell of infitesimal thickness, dr, at a distance r from the nucleus. The volume of this shell is 4πr2dr. The qualitative sketch of the dependence of P on r is
A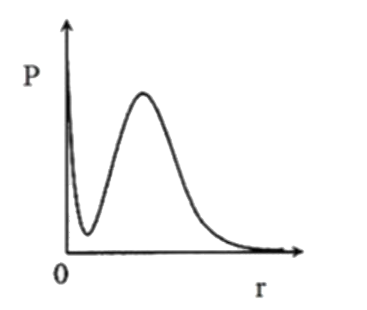 B
B C
C D
D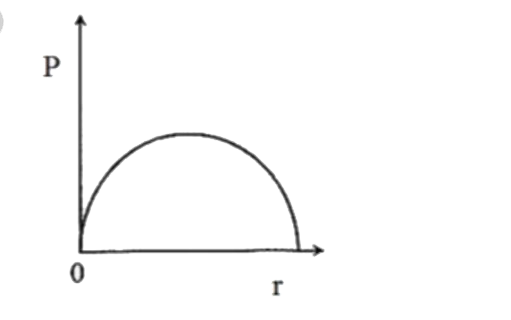
P is the probability of finding the 1s electron of hydrogen atom in a spherical shell of infinitesimal thickness, dr at a distanc, r from the nucleus. The volume of this shell is 4πr2dr. The qualitative sketch of the dependence of P on r is
A B
B C
C D
D
P is the probability of finding the Is electron of hydrogen atom in a spherical shell of infitesimal thickness, dr, at a distance r from the nucleus. The volume of this shell is 4πr2dr. The qualitative sketch of the dependence of P on r is
A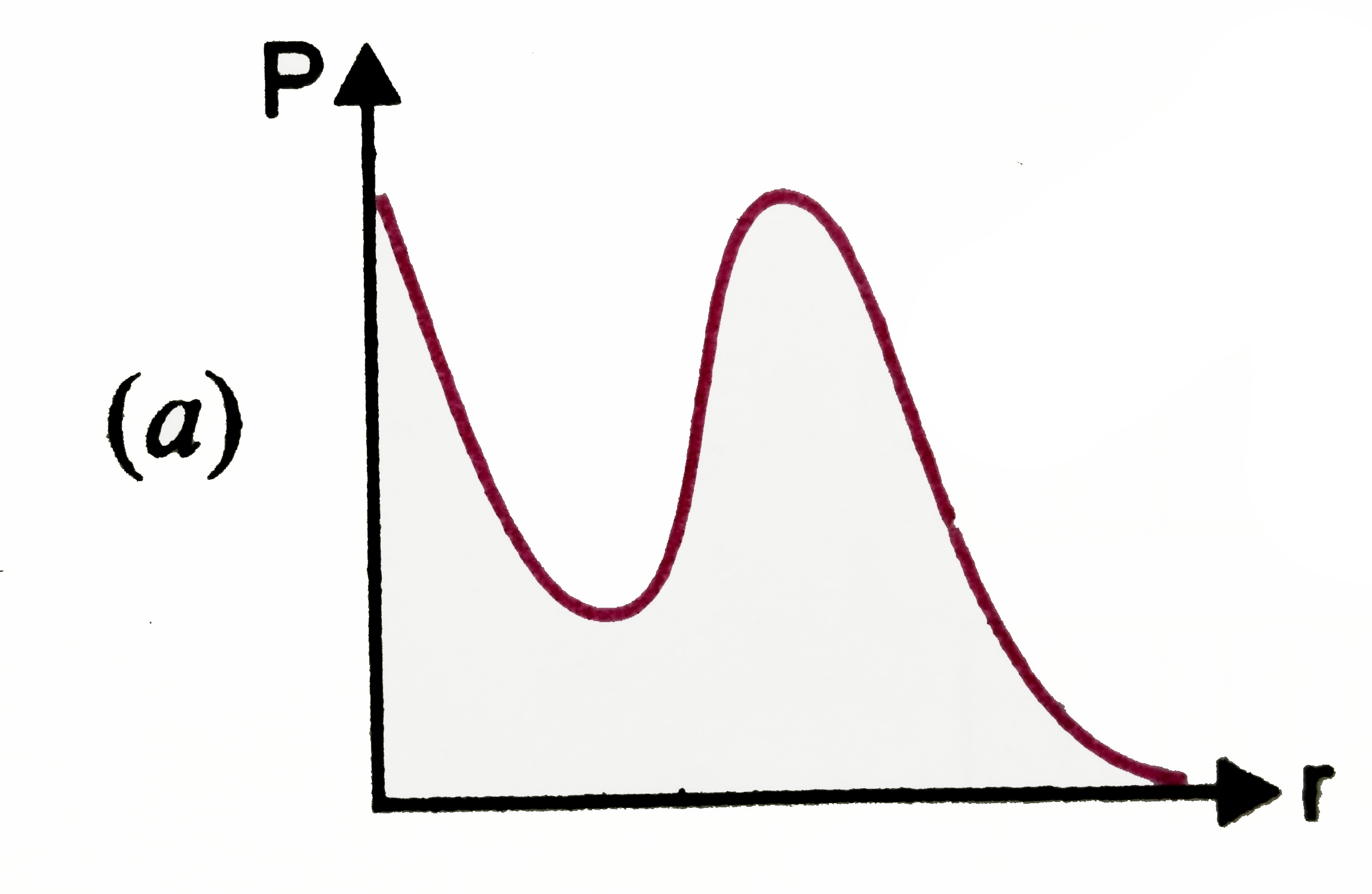 B
B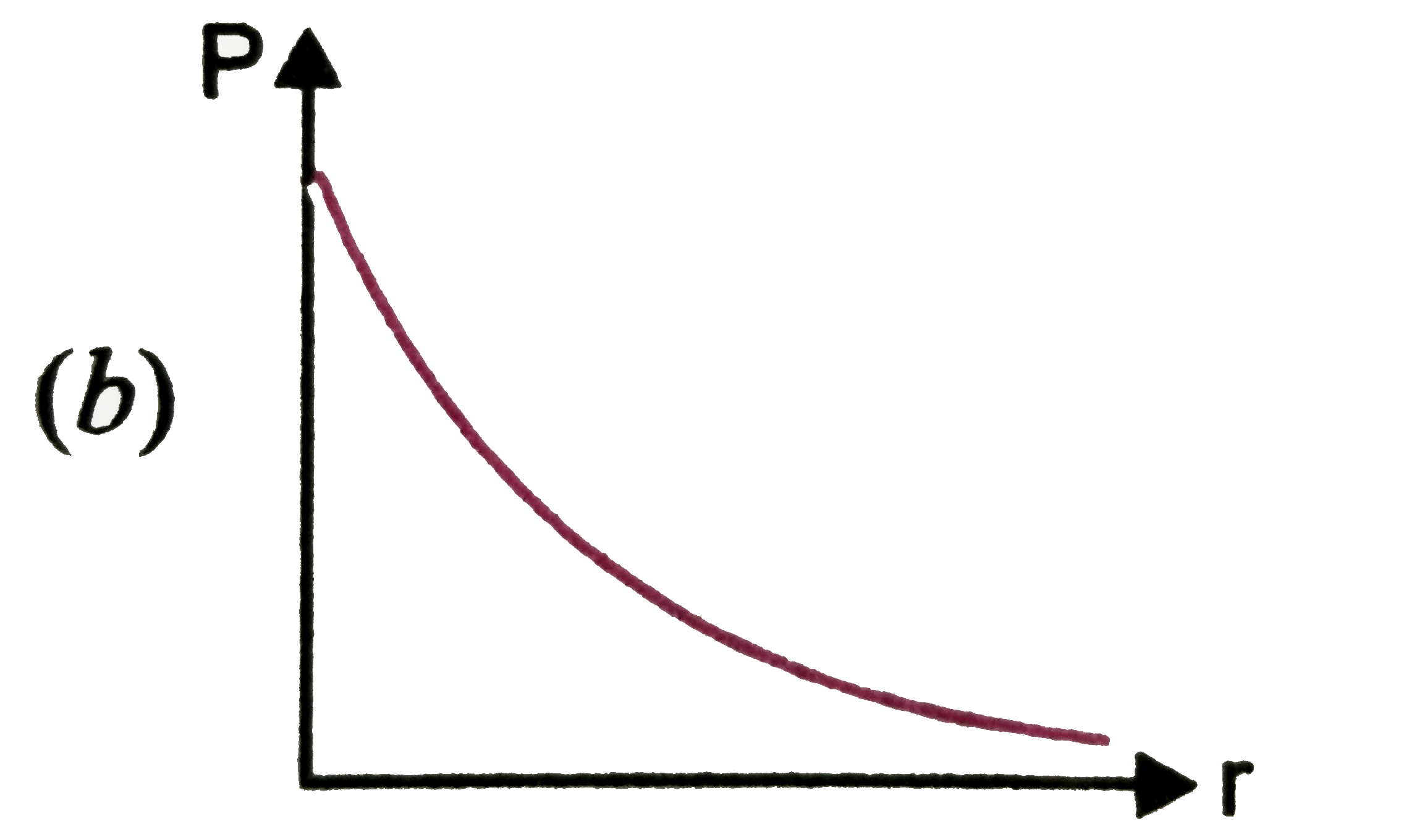 C
C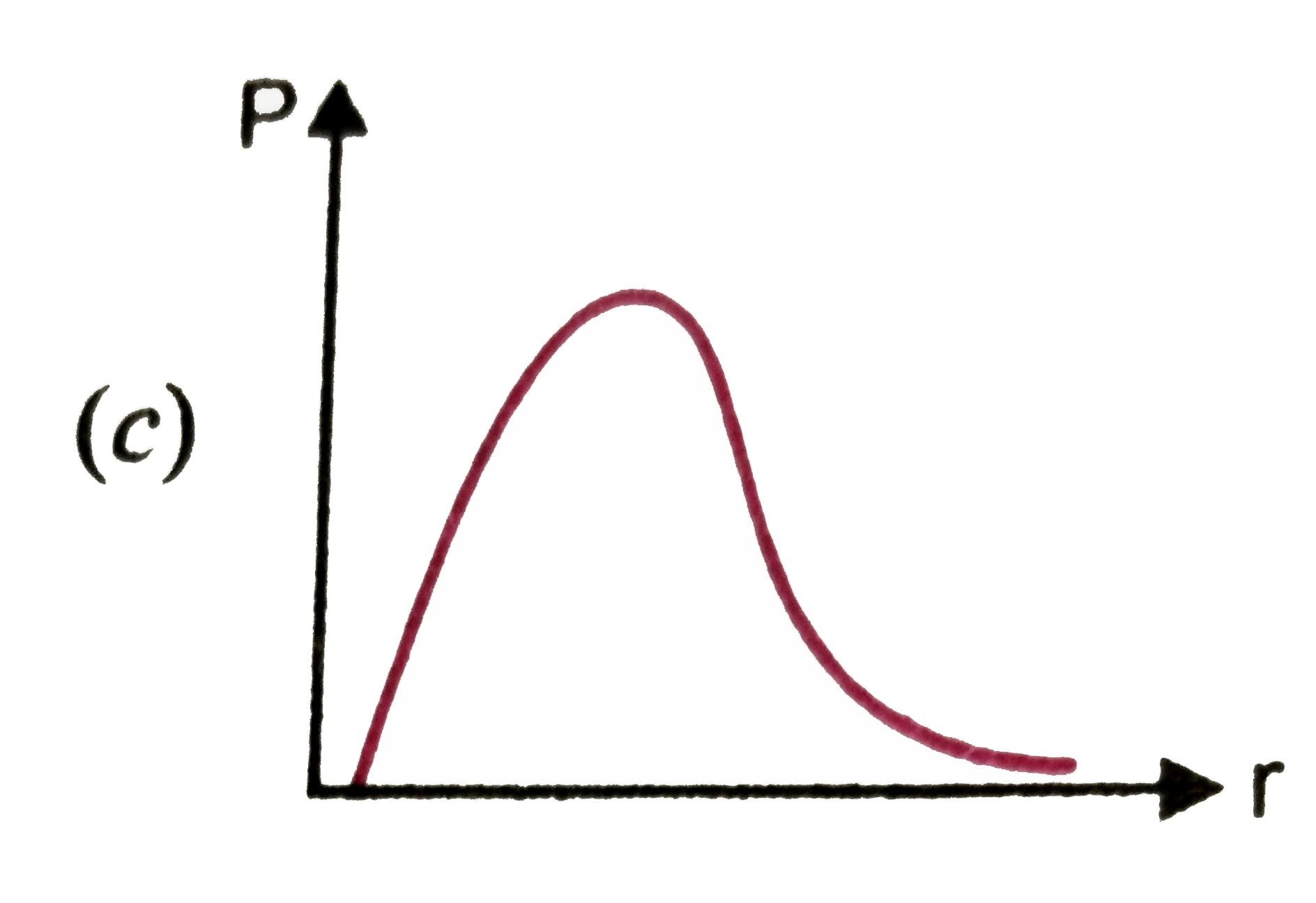 D
D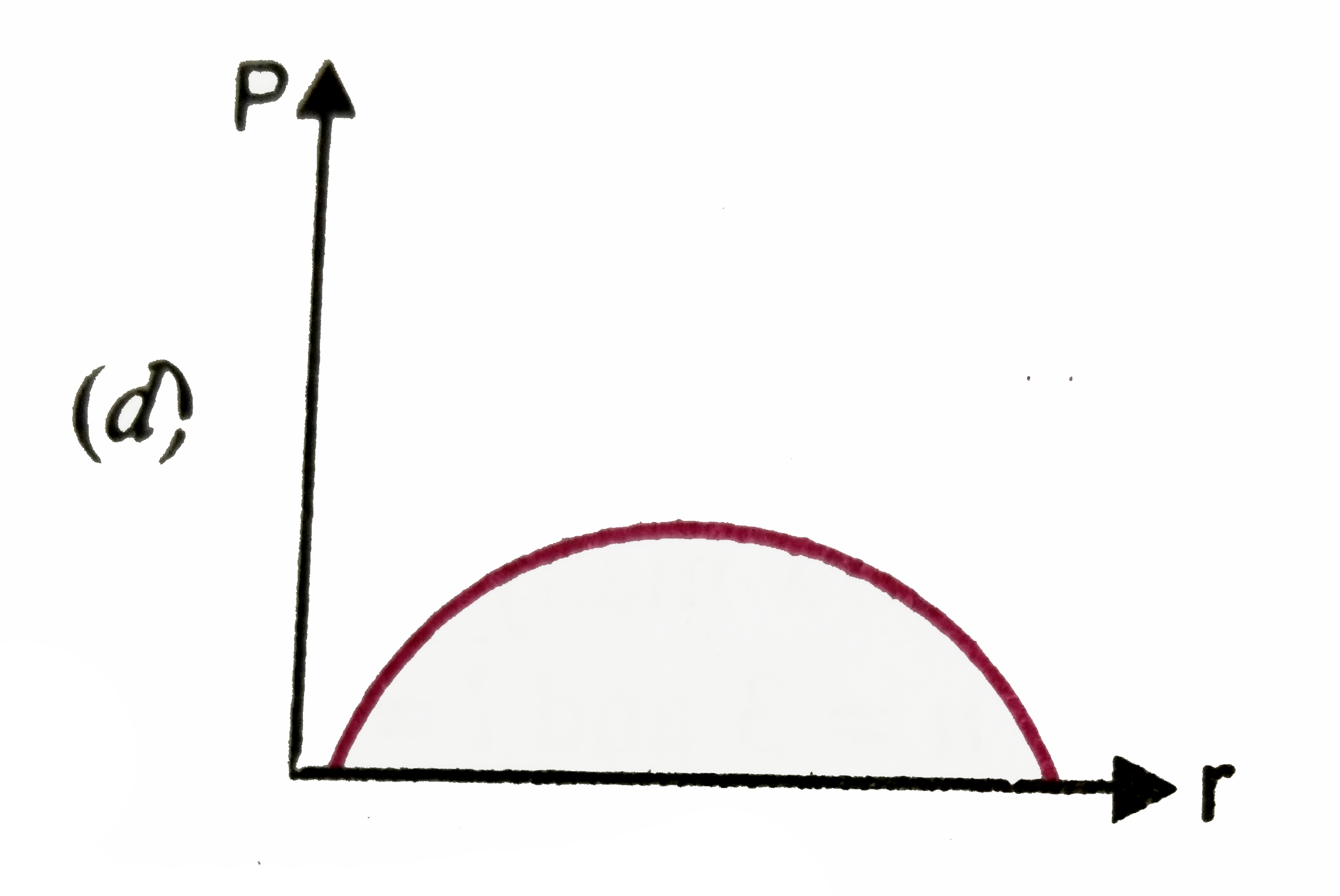
The electrostatic potential of a uniformly charged thin spherical shell of charge Q and radius R at a distance r from the centre
View SolutionThe radial probability is the probability of finding electrons in a small spherical shell around the nucleus at a particular distance ( r ). Hence, radial probability is
View SolutionA point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
View SolutionThe electric field at a distance 3R2 from the centre of a charged conducting spherical shell of radius R is E. The electric field at a distance R2 from the centre of the sphere is :
View SolutionThe electric field at a distance 3R/2 from the centre of a charge conducting spherical shell of radius R is E. The electric field at a distance R/2 from the centre of the sphere is
View Solution
