Doubtnut Promotions Sticky

A planet revolves around the sun in elliptical orbit of eccentricity 'e'. If 'T' is the time period of the planet then the time spent by the planet between the end of the minor axis and close to the sun is

A

Tπ2e

B

T(2eπ−1)

C

Te2π

D

T(14−e2π)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:D

(d) 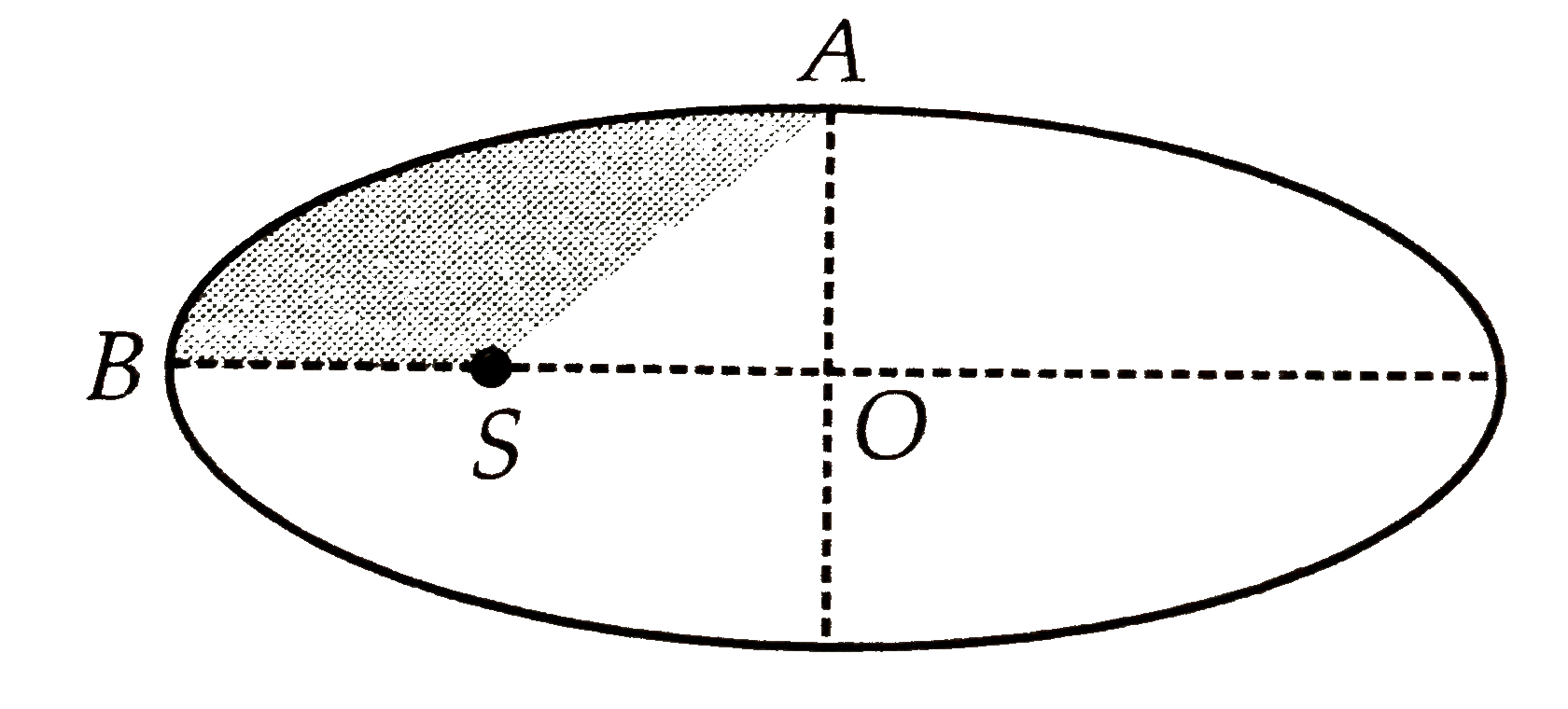ocr_image
As a real velocity of a planet around the sun is constant. Therefore, the desired time is
tAB=(area ABS area of ellipse)× time period
If a= semi-major axis and b=semi- minor axis of ellipse, then , area of ellipse =πab
Area ABS=14(area of ellipse)- Area of triangle ASO
14×πab−12(ea)×(b)
∴tAB=[π(ab)4−12eab]πab×T−T(14−e2π)

|
Updated on:21/07/2023
Class 11PHYSICSGRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    A planet is revolving round the sun in an elliptical orbit, If v is the velocity of the planet when its position vector from the sun is r, then areal velocity of the planet is

    A|v×r|
    B2|r×v|
    C∣∣∣12(r×v)∣∣∣
    DNone of these
  • Question 2 - Select One

    A planet is revolving around the sun as shown in elliptical path. The correct option is
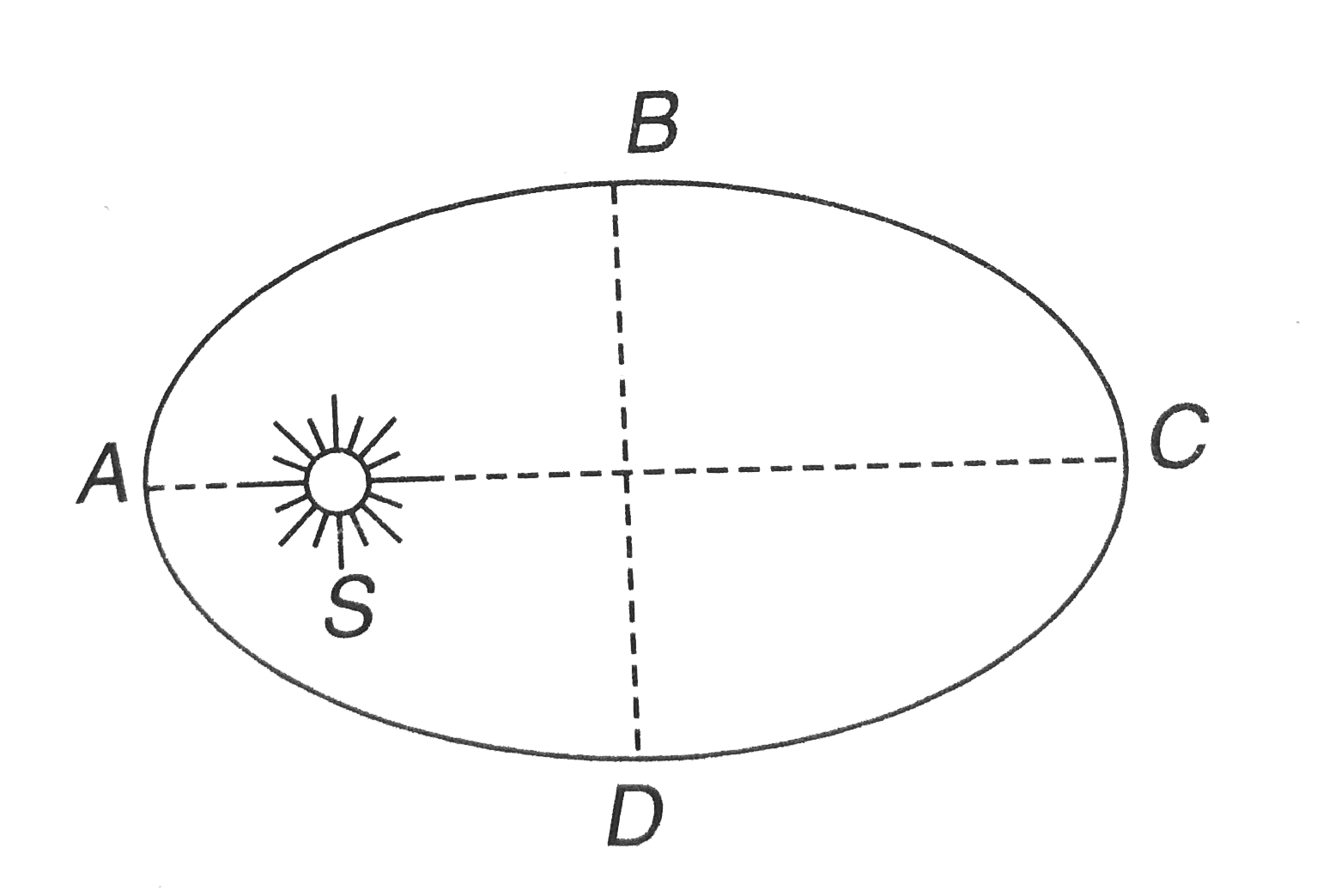    ocr_image

    AThe time taken in travelling DAB is less than that for BCD
    BThe time taken in travelling DAB is greater than that for BCD
    CThe time taken in travelling CDA is less than that for ABC
    DThe time taken in travelling CDA is greater than that for ABC
  • Question 3 - Select One

    A planet is revolving round the sun in an elliptical orbit. Of the following the property which is a constant during the motion of the planet is :

    AThe force of attraction between the planet and sun.
    BThe total energy of the planet plus sun system.
    CThe linear momentum of the planet.
    DThe kinetic energy of the planet about the sun.