Consider a rectangular block of wood moving with a velocity v0 in a gas at temperature T and mass density p. Assume the velocity is along x-axis and the are of cross-section of the block perpendicular to v0 is A. show that the drag force on the block is 4rAv0√kTm where, m is the mass of the gas molecule.
4pAv0√kTm
2pAv0√kT3m
pA2v0√kTm
v0pA√kT2m
The correct Answer is:A
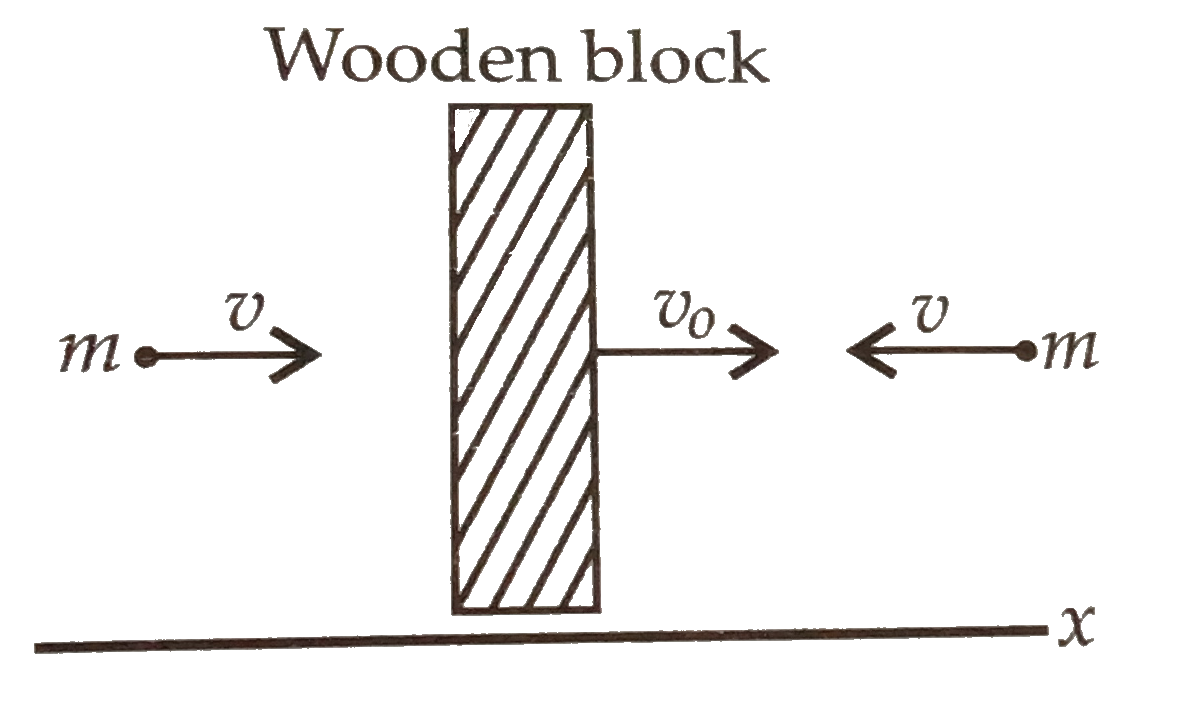
Speed of wooden block =v0
Cross-sectional area of block = A
Temperature of the gas= T
Let n be the number of molecules per unit volume. Rms speed of gas molecules =vrms.
Relative speed of the molecule with respect to front face of the block =v+v0
In head on collision, momentum transferred to block per collision =2m(v+v0) when m is the mass of molecule.
Number of collision in time Δt,=12(v+v0)nΔtA. (factor of 12 appears due to particles moving towards block)
hence, momentum transferred in time Δt,=m(v+v0)2nAΔt
Net force ΔpΔt=m[v+v0)2−(v−v0)2]nA
=mnA(4vv0)=(4ρAv)0=(4ρAv)v0(∵mn=ρ) ...(i)
Also, 12mv2=12kT (v along x-axis)
∴v=√KTm
∴Net force=4ρA√KTmv0. [Using (i)]
This is required drag force.
Topper's Solved these Questions
- BOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - NCERT Exemplar8videos
KINETIC THEORY
- BOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason10videos
KINETIC THEORY
- BOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - Specific Heat Capacity13videos
KINETIC THEORY
- BOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Assertion And Reason15videos
GRAVITATION
- BOOK - NCERT FINGERTIPSCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason15videos
LAWS OF MOTION
Similar Questions
Consider a rectangular block of wood moving with a velocity v0 in a gas at temperature T and mass density p. Assume the velocity is along x-axis and the are of cross-section of the block perpendicular to v0 is A. show that the drag force on the block is 4rAv0√kTm where,m is the mass of the gas molecule.
View SolutionA block of mass 2 kg is moving with a velocity of 2ˆi−ˆj+3ˆk m/s. Find the magnitude and direction of momentum of the block with the x-axis.
View Solution
Knowledge Check
- Question 1 - Select One
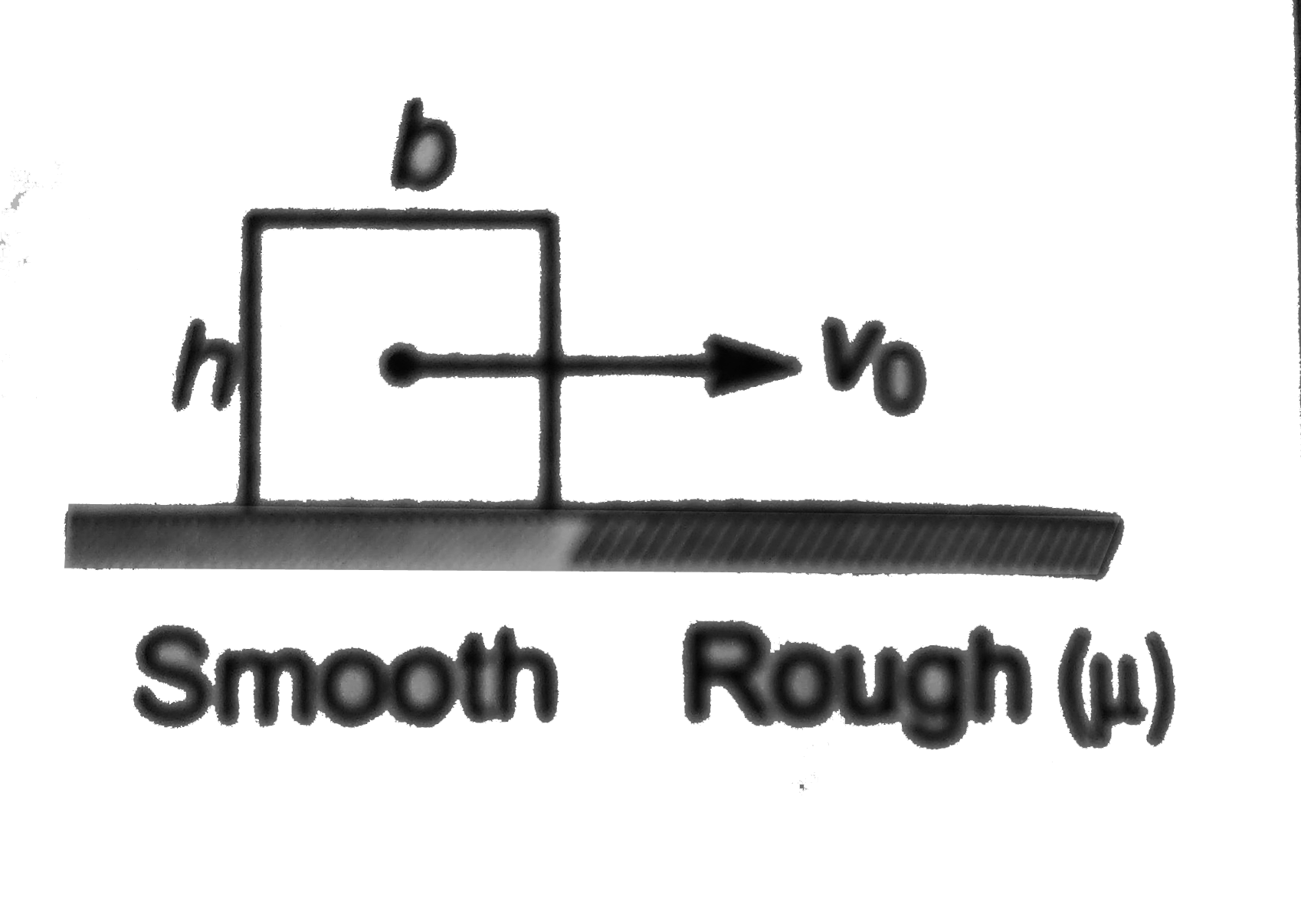
A rectangular block of size (×h) moving with velocity v0 enters on a rough surface where the coefficient of friction is μ as shown in figure. Identify the correct statement.AThenet torque acting on the block about its COM is μmg(h)2 (clockwise)Bthe net torque acting on the block about its COM is zeroCThe net torque acting on the block about its COM is in the anticlockwise senseDNone of the above. - Question 2 - Select One
A bullet of mass m moving with velocity υ1 strikes a suspended wooden block of mass M as shown in the figure and sticks to it. If the block rises to a height y. the initial of the bullet is
 A√2ghBM+mm√ghCmM+m2ghDM+mM√2gh
A√2ghBM+mm√ghCmM+m2ghDM+mM√2gh - Question 3 - Select One
A bullet of mass m moving with velocity υ1 strikes a suspended wooden block of mass M as shown in the figure and sticks to it. If the block rises to a height y. the initial of the bullet is
 A√2ghBM+mm√2ghCmM+m2ghDM+mM√2gh
A√2ghBM+mm√2ghCmM+m2ghDM+mM√2gh
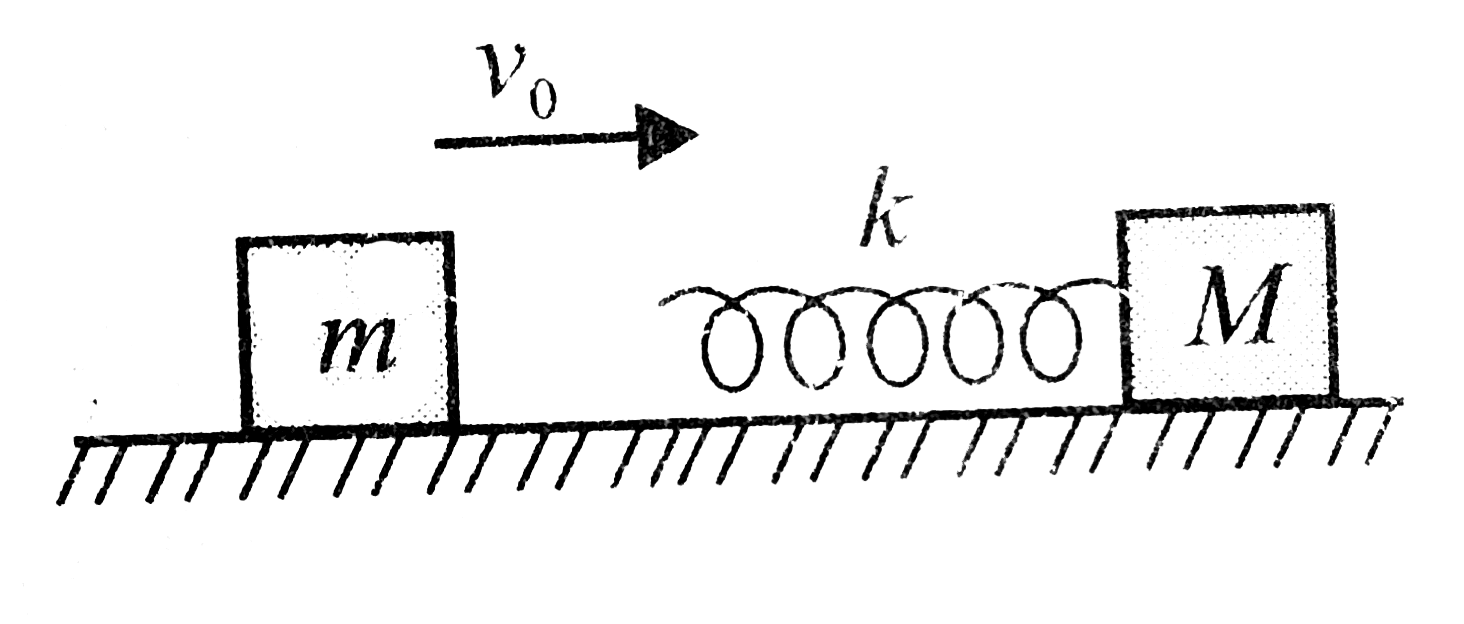
In the fig 4.24 a block of mass m moves with velocity v0 toward a stationaly block of a mass M on a smooth horizontal surface. Find the maximum compression in the spring of stiffnesss constant K.
 View Solution
View SolutionA block of mass m moving with a velocity v0 collides with a stationary block of mass M to which a spring of stiffness k is attached, as shown in Fig. Choose the correct alternative(s)
 View Solution
View SolutionA bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionA bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionA block of mass m moving with velocity v0 on a smooth horizontal surface hits the spring of constant k as shown. Two maximum compression in spring is
 View Solution
View Solution

