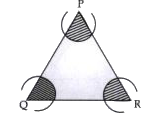
In figure arcs have been drawn with radii 14 cm each and with centres P, Q and R. Find the area of the shaded region .

Video Solution
Text Solution
Given that radii of each arc ( r) = 14cm
Now, area of the sector with central ∠P=∠P360∘×πr2
= ∠P360∘×π×(14)2cm2
[∴ area of any sector with central angle θ and radius r = πr2360∘×θ]
Area of the sector with central central angle = ∠Q360∘×πr2=∠Q360∘×π×(14)2cm2
and area of the sector with central angle R = ∠R360∘×πr2=∠R360∘×π×(14)2cm2
Therefore, sum of the areas (in cm2 ) of three sectors
= ∠P360∘×π×(14)2+∠θ360∘×π×(14)2+∠R360∘×π×(14)2
= ∠P+∠Q+∠R360×196×π=180∘360∘×196πcm2 [since, sum of all interior angles in any triangle is 180∘]
= 98πcm2=98×227
= 14×22=308cm2
Hence, the required area of the shaded region is 308cm2 .
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Three circles with centres A, B and C and with unit radii touch each other at O, P and Q. Find the area of the shaded region .
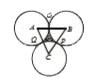 A0.16 sq. unitsB1.21 sq. unitsC0.03 sq. unitsD0.32 units
A0.16 sq. unitsB1.21 sq. unitsC0.03 sq. unitsD0.32 units
Find the area of the shaded region in Figure.
 View Solution
View SolutionFind the area of the shaded region in figure.
 View Solution
View SolutionIn figure , find the area of the shaded region
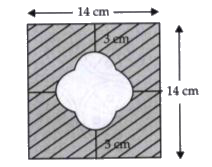 View Solution
View SolutionIn the given figure ABCD is a trapezium in which AB∣∣DC,AB=18cm,DC=32cm and the distance between AB and DC is 14 cm. If arcs of equal radii 7 cm hav been drawn with centres A,B,C and D then find the area of the shaded region.
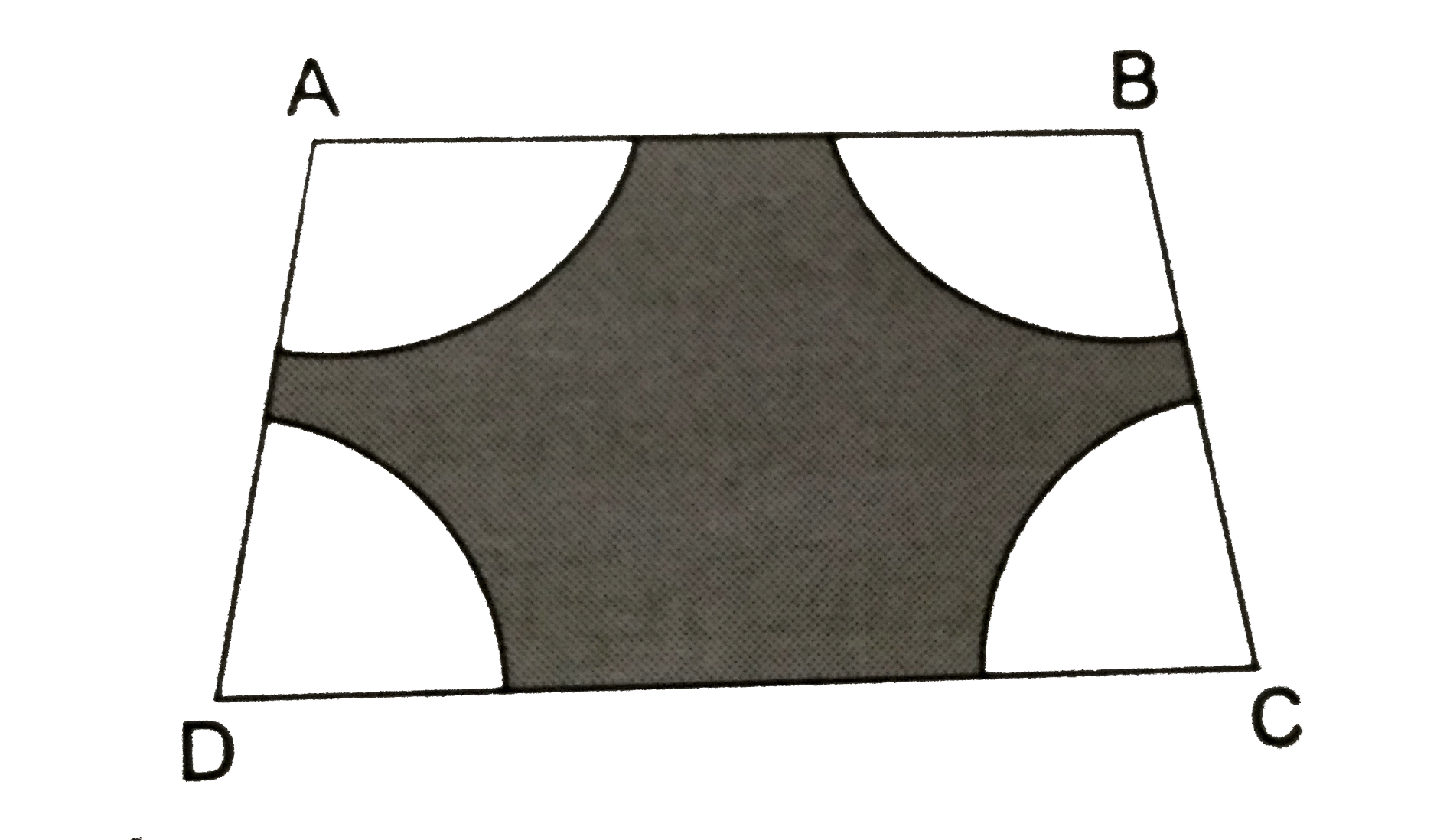 View Solution
View SolutionIn figure, find the area of the shaded region [Useπ=3.14]
View SolutionΔLMN is an equilateral triangle. LM=14cm As shown in figure, three sectors are drawn with vertices as centres and radius 7 cm. Find, Area of the shaded region. (√3=1.732)
View SolutionIn the adjoining figure, find the area of the shaded region (Use π=3.14)
View Solution