There are 175 students in a class, out of which 100 students opt Maths, 70 opt Physics and, 23 opt Physics and Chemisty, 28 opt Chemistry and Maths and 18 opt of all three subjects. Now find each of the following :
No. of students who opt why only Maths
(ii) No. of students who opt only Physics
(iii) No. of students who opt only Chemistry
(iv) No. of students who opt Physics and Chemisty but not Maths
(v) No. of students who opt Maths and Physics but not Chemistry
(vi) No. of students who opt only one subject
(viii) No. of students who opt atleast one subject
(viii) No. of student who do not opt any subject.
Let A = set of students opted Maths
B = set of student opted Physics
C = set of students opted Chemistry
and U = set of students of the class
Given that n(U)=175,n(A)=100,n(B)=70,n(C)46
n(A∩B)=30,n(B∩C)=23,n(C∩A)=28,n(A∩B∩C)=18
(i) No. of students opted Maths only
=n(A∩B'∩C')
=n[A∩(B∪C)']
=n(A)−n[A∩(B∪C)]
[∵n(A∩B')=n(A)−n(A∩B)]
=n(A)−n[(A∩B)∪(A∩C)]
=n(A)−[n(A∩B)+n(A∩C)−n(A∩B∩C)]
=100−30−28+18=60.
(ii) No. of students opted Physics only
=n(B∩A'∩C')
=n(B)−[n(B∩C)+n(B∩A)−n(A∩B∩C)]
=70−(23+30−18)=35.
(iii) No. of students opted Chemistry only
n(C∩A'∩B')
=n(C)−[n(C∩A)+n(C∩B)−n(A∩B∩C)]
=46−(28+23−18)=13.
(iv) No. of students opted Physics and Chemistry but not Maths
=n(B∩C∩A')
=n(B∩C)−n(B∩C∩A)
=23−18=5.
(v) No. fo student opted Maths and Physics but not Chemistry
n(A∩B∩C')=n(A∩B)−n(A∩B∩C)
=30−18=12
(vi) No. of students taken only one subject
=n(A)+n(B)+n(C)−2n(A∩B)−2n(B∩C)−2n(C∩A)+3n(A∩B∩C)
=100+70+46−2(30+23+28)+3×18
=216−162+54=108.
(vii) No. of students taken atleast one subject
n(A∪B∪C)=n(A)+n(B)+n(C)−n(A∩B)−n(B∩C)−n(C∩A)+n(A∩B∩C)
=100+70+46−(30+23+28)+18
=234−81=153
(viii) No. of students taken no subject =n(U)−n(A∪B∪C)=175−153=22
Second method : Here M = set of students taken Maths
P = set of students taken Physics
C = set of students taken Chemistry
Consider the Venn diagram
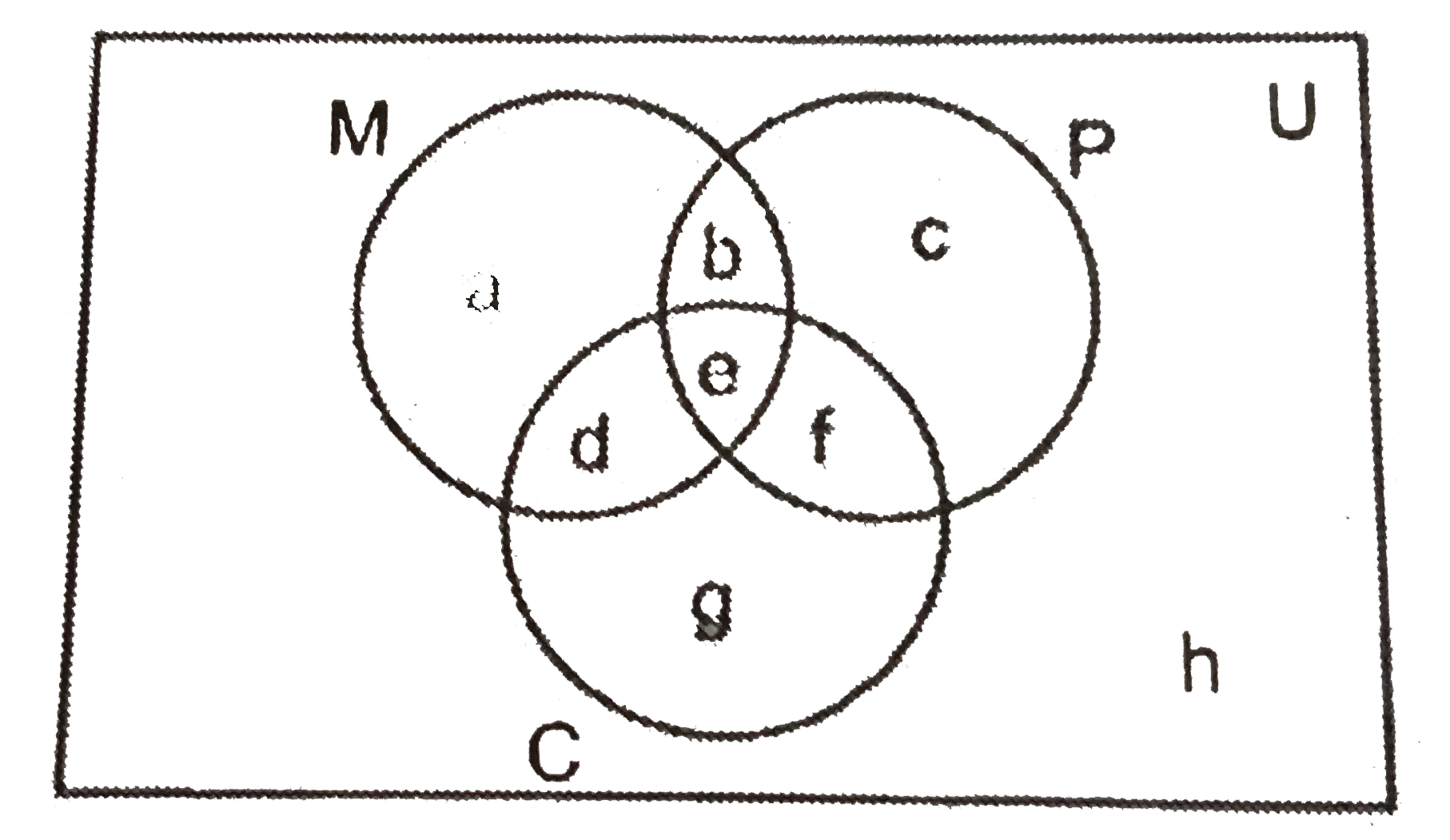
n(M)=a+b+d+e=100
n(P)=b+c+e+f=70
n(C)=d+e+f+g=46
n(M∩P)=b+e=30
n(P∩C)=e+f=23
n(M∩C)=d+e=28
n(M∩P∩C)=r=18
n(U)=a+b+c+d+e+f+g+h
=175
On solving
e=18
d+18=28⇒d=10
18+f=23⇒f=5
b+18=30⇒b=12
n(C)=d+e+f+g=46
⇒10+18+5+g=46
⇒g=13
n(P)=b+c+e+f=70
⇒12+c+18+5=70
⇒c=35
n(M)=a+b+d+e=100
⇒a+12+10+18=100
⇒a=60
and n(U)=a+b+c+d+e+f+g+h
=175
⇒60+12+35+10+18+5+13+h=175
⇒h=22
Now, (i) No. of students taken Maths only =a=60.
(ii) No. of students taken Physics only = c = 35
(iii) No. of student taken Chemisty only = g = 13.
(iv) No. of students taken Physics and Chemistry but not Maths = f = 5
(v) No. of students taken Maths and Physics but not Chemistry = b = 12
(vi) No. of students taken only one subject
=a+c+g
=60+35+13=108
(vii) No, of students taken atleast one subject
=a+b+c+d+e+f+g
=60+12+35+10+18+5+13
=153
(viii) No. of student taken no subject
=h=22.
Topper's Solved these Questions
- SETSBOOK - NAGEEN PRAKASHANCHAPTER - SETSEXERCISE - Exercise 1A
7 Videos
- SETSBOOK - NAGEEN PRAKASHANCHAPTER - SETSEXERCISE - Exercise 1B
10 Videos
- SEQUENCE AND SERIESBOOK - NAGEEN PRAKASHANCHAPTER - SEQUENCE AND SERIESEXERCISE - Miscellaneous Exercise
32 Videos
- STATISTICSBOOK - NAGEEN PRAKASHANCHAPTER - STATISTICSEXERCISE - MISCELLANEOUS EXERCISE
7 Videos
Similar Questions
In a school, 21 student read Maths, 26 reads Physics and 29 read Chemistry. If 14 students read Maths and Physics, 15 read Physics and Chemistry, 12 read Chemisry and Maths and 8 read all three subjects, then find the total students in the school.
View SolutionIn a class of 35 students, 18 students opt Maths, 12 students opt Maths but not Science. Find the number of students who opt both subjects. Also find the number of students two opt Science but not Maths. It is given that each student opt atleast one subject.
View Solution
Knowledge Check
In a class 20 students opted for Physics, 17 for Maths,12 for both Physics and Maths and 10 students for other subjects. The class contains how many students ?
A52B35C50D42In a class , 40 student like Maths, 50 students like Physics and 60 students like Chemistry. 30 students like both Maths and Physics, 20 students like both Physics and Chemistry and none of them like both Maths of Chemistry . Find the total number of students who like only Maths, only Physics and only Chemistry.
A10B40C50D30
Out of 1800 students in a school, 750 opted basketball, 800 opted cricket and remaining opted table tennis. If a student can opt only one game, find the ratio of(a) Number of students who opted basketball to the number of students who opted table tennis.(b) Number of students who opted cricket to the number of students optingbasketball.(c) Number of students who opted basketball to the total number of students.
View Solution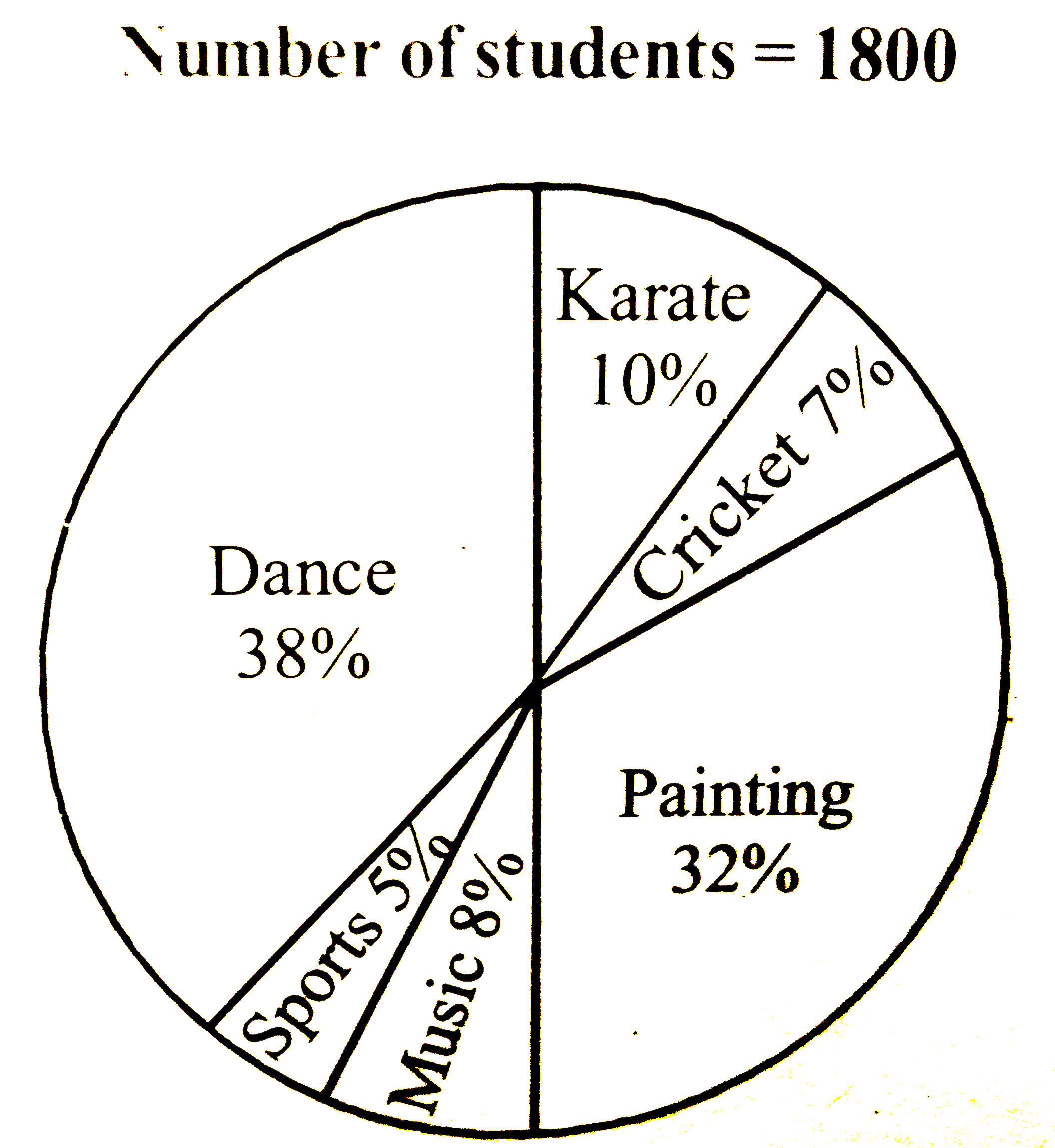
What is the number of students who opted for dancing ?
View SolutionOut of 1800 students in a school, 750 opted basketball, 800 opted cricket and remaining opted table tennis. If a student can opt only one game, find the ratio of
Number of students who opted basketball to the number of students who opted table tennis.
View SolutionOut of 1800 students in a schools, 750 opted basketball, 800 opted cricket and remaining opted table tennis. If a student can only one game, find the ratio of :
Number of students who opted cricket to the number of students who opted basketball.
View SolutionIn a class of 100 students, 60 like Mathematics, 72 like Physics, 68 like Chemistry and no student likes all three subjects. Then number of students who don't like Mathematics and Chemistry is
View SolutionOut of 1800 students in a schools, 750 opted basketball, 800 opted cricket and remaining opted table tennis. If a student can only one game, find the ratio of :
Number of students who opted basketball to number of students who opted table tennis.
View Solution
