It is required to get minimum marks in each subject out of 5 subject to pass in an examination. In how many ways can a student fail?
To solve the problem of how many ways a student can fail in an examination with 5 subjects, we can follow these steps:
1. Identify the Choices for Each Subject:
Each subject has two possible outcomes: the student can either pass or fail.
2. Calculate Total Outcomes:
Since there are 5 subjects and each subject has 2 choices (pass or fail), the total number of outcomes can be calculated using the formula for the total number of combinations:
Total Outcomes=2n
where n is the number of subjects. Here, n=5:
Total Outcomes=25=32
3. Identify the Passing Outcome:
There is only one specific way for the student to pass all subjects, which is:
- Pass in Subject 1
- Pass in Subject 2
- Pass in Subject 3
- Pass in Subject 4
- Pass in Subject 5
This can be represented as (Pass, Pass, Pass, Pass, Pass).
4. Calculate the Failing Outcomes:
To find the number of ways a student can fail, we subtract the one passing outcome from the total outcomes:
Failing Outcomes=Total Outcomes−Passing Outcomes
Failing Outcomes=32−1=31
5. Conclusion:
Therefore, the number of ways a student can fail in the examination is 31.
Final Answer:
The number of ways a student can fail is 31.
---
Each subject has two possible outcomes: the student can either pass or fail.
Since there are 5 subjects and each subject has 2 choices (pass or fail), the total number of outcomes can be calculated using the formula for the total number of combinations:
Total Outcomes=2n
where n is the number of subjects. Here, n=5:
Total Outcomes=25=32
There is only one specific way for the student to pass all subjects, which is:
- Pass in Subject 1
- Pass in Subject 2
- Pass in Subject 3
- Pass in Subject 4
- Pass in Subject 5
To find the number of ways a student can fail, we subtract the one passing outcome from the total outcomes:
Failing Outcomes=Total Outcomes−Passing Outcomes
Failing Outcomes=32−1=31
Therefore, the number of ways a student can fail in the examination is 31.
The number of ways a student can fail is 31.
Topper's Solved these Questions
- PERMUTATION AND COMBINATION BOOK - NAGEEN PRAKASHANCHAPTER - PERMUTATION AND COMBINATION EXERCISE - Exercise A
9 Videos
- PERMUTATION AND COMBINATION BOOK - NAGEEN PRAKASHANCHAPTER - PERMUTATION AND COMBINATION EXERCISE - Exercise B
10 Videos
- MATHEMATICAL REASONING BOOK - NAGEEN PRAKASHANCHAPTER - MATHEMATICAL REASONING EXERCISE - Misellaneous exercise
7 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - NAGEEN PRAKASHANCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Exercise 4
29 Videos
Similar Questions
Knowledge Check
Study the following table carefully and answer the questions given below-

If the minimum percentage is 70 in each subject to be declared pass in the examination, how many students failed in the examination ?A1B2CAllD3In an examination 80% of the students passed in maths, 65% of the students passed in reasoning 15% of the students failed in both the subjects . 150 students who passed only maths, then find how many students failed only reasoning.
A155B160C150D165In an examination, it is required to get 296 marks out of aggregate marks to pass. A student gets 222 marks and is declared failed by 10% marks. What are the maximum aggregate marks a student can get?
A830B810C780D740
In order to pass B.E. final, minimum marks has to be secured in each of the 6 subjects. In how many cases can a student fail?
View SolutionTwo subjects are compulsory for a student in an examination. In how many ways can a student select 5 subjects out of given 10 subjects?
View SolutionIn an Examination, a candidate has to pass in each of the four subjects. In how many ways can be fail ?
View SolutionIn a boards examination, 40 students failed in physics, 40 in chemistry and 35 in mathematics, 20 failed in maths and physics, 17 in physics and chemistry, 15 in maths and chemistry and 5 in all the three subjects. If 350 students appeared in the examination, how many of them did not fail in any subject?
View SolutionThe following pie diagram gives the marks scored by a student in different subjects in an examination. Assuming that the total marks scored by the student in the examination are 540, answer the question below.
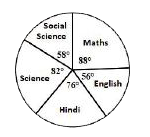
The subject in which the student scored 123 marks is:
View Solution
