Which statement is incorrect about osmotic pressure $(π)$ , volume $(V)$ , and temperature $(T)$ ?

A

$π \propto \frac{1}{V}$ if T is constant

B

$π \propto T$ if V is constant

C

$π \propto V$ if T is constant

D

$π V$ is constant if T is constant

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, D

For a solution containing n moles of a non electrolyte solute
$π \propto \frac{1}{V}$ (at constant T)
or $π V$ = Constant (at constant T)
$π \propto T$ (at constant V)

|

Updated on:21/07/2023

Class 12 CHEMISTRY SOLUTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One

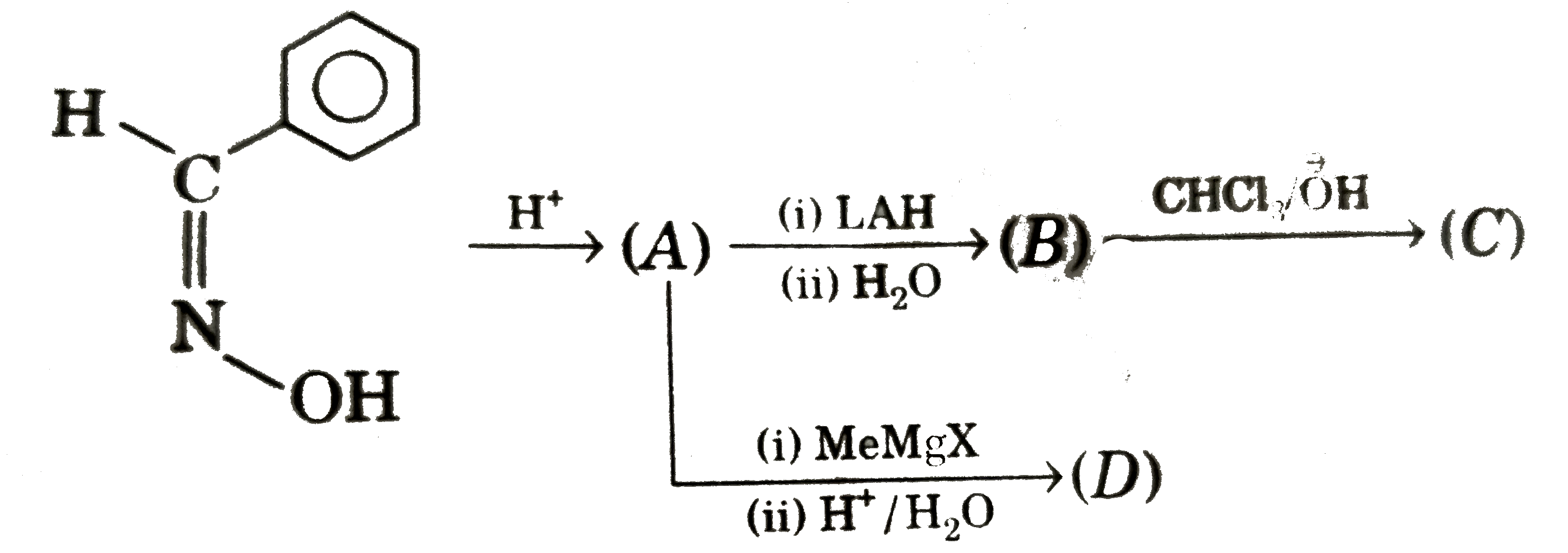
Which statement is incorrect about D?
AD can produce yellow ppt. on reaction with $Θ OH / I_{2}$
BD can be prepared by $P h - C \equiv C H$ on reaction with $H g^{2 +} / H^{+} / H_{2} O$
CD can form geometrically isomeric oximes on reaction with $N H_{2} - O H$
DD can be converted into alcohol on reaction with $Z n (H g) / H C l$ .
Question 2 - Select One
Which statement is incorrect about osmotic pressure $(π)$ , volume $(V)$ , and temperature $(T)$ ?
A $p \propto 1 / V$ if T is constant
B $p \propto T$ if V is constant
C $p \propto V$ if V is constant
DpV is constant if T is constant
Question 3 - Select One
Which statement is incorrect about osmotic pressure (P), volume (V) and temperature (T)
A $P \propto \frac{1}{V},$ if T is constant
B $P \propto T,$ if Vis constant
C $P \propto V,$ if T is constant
DPV is constant, if T is constant

DINESH PUBLICATION-SOLUTIONS -OBJECTIVE TYPE MCQs

Home
Ask Doubt
Profile