The dissociation constant for an acid HA is 1.6×10−5. Calculate its H3O+ in concentration in 0.01 M solution .
The correct Answer is:4.0×10−4 mol L−1
To calculate the concentration of H3O+ in a 0.01 M solution of the weak acid HA with a dissociation constant Ka=1.6×10−5, we can follow these steps:
Step 1: Write the dissociation equation
The dissociation of the weak acid HA in water can be represented as:
HA⇌H++A−
In aqueous solution, H+ is often represented as H3O+.
Step 2: Set up the expression for the dissociation constant
The expression for the dissociation constant Ka is given by:
Ka=(H3O+)(A−)(HA)
Assuming that the initial concentration of HA is C=0.01M, and at equilibrium, let α be the degree of dissociation. Thus, we can express the concentrations at equilibrium as follows:
- (H3O+)=Cα
- (A−)=Cα
- (HA)=C(1−α)
Step 3: Substitute into the Ka expression
Substituting these values into the Ka expression gives:
Ka=(Cα)(Cα)C(1−α)=C2α2C(1−α)=Cα21−α
Step 4: Simplify the equation
Assuming α is small (which is typical for weak acids), we can approximate 1−α≈1. Thus, the equation simplifies to:
Ka≈Cα2
Step 5: Solve for α
Rearranging the equation gives:
α2=KaC
Substituting the values:
α2=1.6×10−50.01=1.6×10−3
Taking the square root:
α=√1.6×10−3≈0.04
Step 6: Calculate the concentration of H3O+
Now, we can find the concentration of H3O+:
(H3O+)=Cα=0.01×0.04=0.0004M=4×10−4M
Final Answer
The concentration of H3O+ in a 0.01 M solution of the acid HA is:
(H3O+)=4×10−4M
---
The dissociation of the weak acid HA in water can be represented as:
HA⇌H++A−
In aqueous solution, H+ is often represented as H3O+.
The expression for the dissociation constant Ka is given by:
Ka=(H3O+)(A−)(HA)
Assuming that the initial concentration of HA is C=0.01M, and at equilibrium, let α be the degree of dissociation. Thus, we can express the concentrations at equilibrium as follows:
- (H3O+)=Cα
- (A−)=Cα
- (HA)=C(1−α)
Substituting these values into the Ka expression gives:
Ka=(Cα)(Cα)C(1−α)=C2α2C(1−α)=Cα21−α
Assuming α is small (which is typical for weak acids), we can approximate 1−α≈1. Thus, the equation simplifies to:
Ka≈Cα2
Rearranging the equation gives:
α2=KaC
Substituting the values:
α2=1.6×10−50.01=1.6×10−3
Taking the square root:
α=√1.6×10−3≈0.04
Now, we can find the concentration of H3O+:
(H3O+)=Cα=0.01×0.04=0.0004M=4×10−4M
The concentration of H3O+ in a 0.01 M solution of the acid HA is:
(H3O+)=4×10−4M
Topper's Solved these Questions
- EQUILIBRIUMBOOK - DINESH PUBLICATIONCHAPTER - EQUILIBRIUMEXERCISE - NCERT
92 Videos
- EQUILIBRIUMBOOK - DINESH PUBLICATIONCHAPTER - EQUILIBRIUMEXERCISE - Multiple choice Qestions
16 Videos
- EQUILIBRIUMBOOK - DINESH PUBLICATIONCHAPTER - EQUILIBRIUMEXERCISE - value Based questions
3 Videos
- ENVIRONMENTAL CHEMISTRYBOOK - DINESH PUBLICATIONCHAPTER - ENVIRONMENTAL CHEMISTRYEXERCISE - Integer Type Questions
4 Videos
- GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSBOOK - DINESH PUBLICATIONCHAPTER - GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - UNIT TEST - 34
1 Videos
Similar Questions
The dissociation constatn of 0.01 M CH3COOH is 1.8×10−5 then calculate CH3COO− concentration of 0.1 M HCl solution.
View SolutionThe ionization constant of propanoic acid is 1.32×10−15. Calculate the degree of ionization if its solution is 0.05 M. What will be its degree of ionization if the solution is0.01 M in HCl solution.
View Solution
Knowledge Check
The first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HS− ion in its 0.1 M solution. How will this concentration be affected, if the solution is 0.1 M in HCl also? If the second dissociation constant of H2S is 1.2×10−13 , then calculate the concentration of S2− under both conditions. Select these four answers from the choices given below.
A B
B C
C DNone of these
DNone of theseThe first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HS− ion in its 0.1 M solution. How will this concentration be affected, if the solution is 0.1 M in HCl also? If the second dissociation constant of H2S is 1.2×10−13 , then calculate the concentration of S2− under both conditions. Select these four answers from the choices given below.
A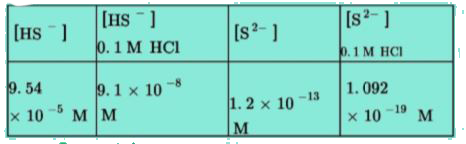 B
B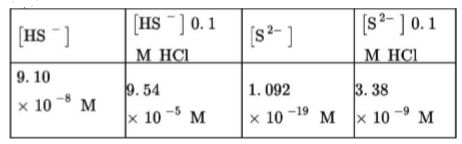 C
C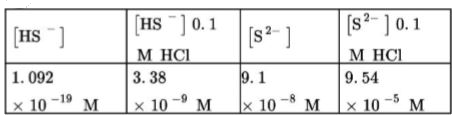 DNone of these
DNone of theseThe dissociation constant of weak acid HA is 1×10−5. Its concentration is 0.1M. pH of that solution is
A1.0B2.0C3.0D5.0
The first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HSΘ ion in its 0.1M solution. How will this concentration be affected if the solution is 0.1M in HCl also? If the second dissociation constant if H2S is 1.2×10−13, calculate the concentration of S2− under both conditions.
View SolutionThe first ionization constant of H2S is 9.1×10−8. Calculate the concentration of HSΘ ion in its 0.1M solution. How will this concentration be affected if the solution is 0.1M in HCl also? If the second dissociation constant if H2S is 1.2×10−13, calculate the concentration of S2− under both conditions.
View SolutionKa for an acid HA is 4.9×10−8. Calculate percentage dissocitation H+ ion concentration for its 0.1 M aqueous solution.
View SolutionThe ionization constant of acetic acid 1.74×10−5. Calculate the degree of dissociation of acetic acid in its 0.05M solution. Calculate the concentration of acetate ion in the solution and its pH.
View SolutionThe ionization constant of acetic acid 1.74×10−5. Calculate the degree of dissociation of acetic acid in its 0.05M solution. Calculate the concentration of acetate ion in the solution and its pH.
View Solution
