Molar conductivity of an electrolyte is the conductance of all the ions produced by one gram mole of the electrolyte in solution and is denoted as .
Here k is the specific conductance while c is the molar concentration of the electrolyte. The molar conductance of the strong electrolytes at infinite dilution can be obtained graphically by extrapolation while the same for weak electrolytes cannot be obtained graphically. It can be calculated theoretically with the help of Kohrausch's. Law.
Equivalent conductance of 1 M methanoic acid solution is and at infinite dilution it is . The pH of methanoic acid solution is :
7
3.3
1.3
6.8.
The correct Answer is:C
(c )
=2-0.6990=1.3
Topper's Solved these Questions
- BOOK - DINESH PUBLICATIONCHAPTER - ALCOHOLS AND PHENOLSEXERCISE - COMPLETION QUESTION2videos
ALCOHOLS AND PHENOLS
- BOOK - DINESH PUBLICATIONCHAPTER - ALCOHOLS AND PHENOLSEXERCISE - NCERT In-Text Questions9videos
ALCOHOLS AND PHENOLS
- BOOK - DINESH PUBLICATIONCHAPTER - ALDEHYDES AND KETONES EXERCISE - Interger5videos
ALDEHYDES AND KETONES
Similar Questions
Match the following Column - I and Column - II
 04:42View Solution
04:42View SolutionClassify the following into primary, secondary and tertiary alcohols
(i) CH−CH3∣C∣CH3−CH2OH, (ii) H2C=CH−CH2OH, (iii) CH3−CH2−CH2OH
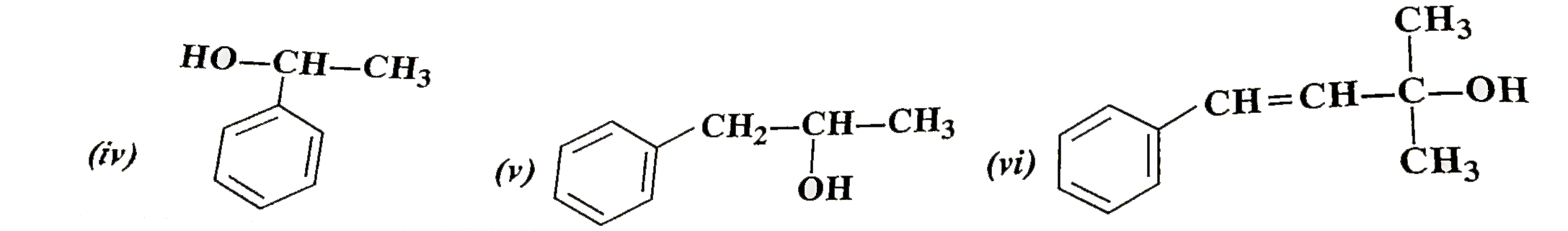 View Solution
View SolutionTotal number of acyclic isomers having formula C3H6O :
View SolutionStatement: For a weak electrolyte, the plot of molar conductivity (Lambda_(m)) against sqrt(C )(C is concentration in mol litre^(-1)) is nearly linear. Explanation: The molar conductivity at infinite dilution (Lambda_(m)) for an electrolyte can be considered equal to the sum of the limiting molar conductivities of the individual ions.
02:38View SolutionMolar conductivity of an electrolyte is the conductance of all the ions produced by one gram mole of the electrolyte in solution and is denoted as Lambda_(m) . Lambda_(m)=(kxx1000)/(c ) Here k is the specific conductance while c is the molar concentration of the electrolyte. The molar conductance of the strong electrolytes at infinite dilution (Lambda_(m)^(oo)) can be obtained graphically by extrapolation while the same for weak electrolytes cannot be obtained graphically. It can be calculated theoretically with the help of Kohrausch's. Law. Lambda_(m(AxBy))^(oo)=xlambda_(m (A^(y+)))^(oo)+ylambda_(m(B^(x)))^(oo) 48250 C of electricity was required to deposit all the copper present in 0.5 L of CuSO_(4) solution using inert electrodes. The molarity of solution was (Assume volume constant).
02:17View SolutionMolar conductivity of an electrolyte is the conductance of all the ions produced by one gram mole of the electrolyte in solution and is denoted as Lambda_(m) . Lambda_(m)=(kxx1000)/(c ) Here k is the specific conductance while c is the molar concentration of the electrolyte. The molar conductance of the strong electrolytes at infinite dilution (Lambda_(m)^(oo)) can be obtained graphically by extrapolation while the same for weak electrolytes cannot be obtained graphically. It can be calculated theoretically with the help of Kohrausch's. Law. Lambda_(m(AxBy))^(oo)=xlambda_(m (A^(y+)))^(oo)+ylambda_(m(B^(x)))^(oo) Which of the following solution of KCl will have the maximum value of specific conductance ?
02:06View SolutionConductors allow the passage of electric current through them. Metallic and electrolytic are the two type of conductors. Current carriers in metallic and electrolytic conductors are free electrons and free ions respectively. Specific conductance or consuctivity of the electrolyte solution is given by the following relation :
κ=c×lA
where, c=1/R is the conductance and l/A is the cell constant. Molar conductance (Λm) and equivalence conductance (Λe) of an electrolyte solution are calculated using the following similar relations :
Λm=κ×1000M
Λe=κ×1000N
Where, M and N are the molarity and normality of the solution respectively. Molar conductance of strong electrolyte depends on concentration :
Λm=Λ∘m−b√c
where, Λ∘m= molar conductance at infinite dilution
c= concentration of the solution
b= constant
The degrees of dissociation of weak electrolytes are calculated as :
α=ΛmΛ∘m=ΛeΛ∘e
Which of the following decreases on dilution of electrolyte solution ?02:04View SolutionConductors allow the passage of electric current through them. Metallic and electrolytic are the two type of conductors. Current carriers in metallic and electrolytic conductors are free electrons and free ions respectively. Specific conductance or consuctivity of the electrolyte solution is given by the following relation :
κ=c×lA
where, c=1/R is the conductance and l/A is the cell constant. Molar conductance (Λm) and equivalence conductance (Λe) of an electrolyte solution are calculated using the following similar relations :
Λm=κ×1000M
Λe=κ×1000N
Where, M and N are the molarity and normality of the solution respectively. Molar conductance of strong electrolyte depends on concentration :
Λm=Λ∘m−b√c
where, Λ∘m= molar conductance at infinite dilution
c= concentration of the solution
b= constant
The degrees of dissociation of weak electrolytes are calculated as :
α=ΛmΛ∘m=ΛeΛ∘e
The correct order of equivalent conductances at infinite dilution of LiCl, NaCl and KCl is :01:39View SolutionConductors allow the passage of electric current through them. Metallic and electrolytic are the two type of conductors. Current carriers in metallic and electrolytic conductors are free electrons and free ions respectively. Specific conductance or consuctivity of the electrolyte solution is given by the following relation :
κ=c×lA
where, c=1/R is the conductance and l/A is the cell constant. Molar conductance (Λm) and equivalence conductance (Λe) of an electrolyte solution are calculated using the following similar relations :
Λm=κ×1000M
Λe=κ×1000N
Where, M and N are the molarity and normality of the solution respectively. Molar conductance of strong electrolyte depends on concentration :
Λm=Λ∘m−b√c
where, Λ∘m= molar conductance at infinite dilution
c= concentration of the solution
b= constant
The degrees of dissociation of weak electrolytes are calculated as :
α=ΛmΛ∘m=ΛeΛ∘e
For which of the following electrolytic solution Λm and Λe are equal ?View SolutionConductors allow the passage of electric current through them. Metallic and electrolytic are the two type of conductors. Current carriers in metallic and electrolytic conductors are free electrons and free ions respectively. Specific conductance or consuctivity of the electrolyte solution is given by the following relation :
κ=c×lA
where, c=1/R is the conductance and l/A is the cell constant. Molar conductance (Λm) and equivalence conductance (Λe) of an electrolyte solution are calculated using the following similar relations :
Λm=κ×1000M
Λe=κ×1000N
Where, M and N are the molarity and normality of the solution respectively. Molar conductance of strong electrolyte depends on concentration :
Λm=Λ∘m−b√c
where, Λ∘m= molar conductance at infinite dilution
c= concentration of the solution
b= constant
The degrees of dissociation of weak electrolytes are calculated as :
α=ΛmΛ∘m=ΛeΛ∘e
The conductance of a solution of an electrolyte is equal to that of its specific conductance. The cell constant of the conductivity cell is equal to :04:07View SolutionConductors allow the passage of electric current through them. Metallic and electrolytic are the two type of conductors. Current carriers in metallic and electrolytic conductors are free electrons and free ions respectively. Specific conductance or consuctivity of the electrolyte solution is given by the following relation : kappa=c xx l/A where, c=1//R is the conductance and l//A is the cell constant. Molar conductance (Lambda_(m)) and equivalence conductance (Lambda_(e)) of an electrolyte solution are calculated using the following similar relations : Lambda_(m)= kappa xx 1000/M Lambda_(e)= kappa xx 1000/N Where, M and N are the molarity and normality of the solution respectively. Molar conductance of strong electrolyte depends on concentration : Lambda_(m)=Lambda_(m)^(@)-b sqrt(c) where, Lambda_(m)^(@)= molar conductance at infinite dilution c= concentration of the solution b= constant The degrees of dissociation of weak electrolytes are calculated as : alpha=Lambda_(m)/Lambda_(m)^(@)=Lambda_(e)/Lambda_(e)^(@) Which of the following equality holds good for the strong electrolytes ?
05:29View SolutionStatement-1: Specific conductivity of an electrolytic solution decreases with dilution whereas molar conductivity.
Statement-2: Specific conductivity is the conductance of a specific amount of the electrolyte whereas molar conductivity is for 1 mole of the electrolyte.06:23View SolutionAssertion:Kohlrausch law helps to find the molar conductivity of weak electrolyte at infinite dilution. Reason:Molar conductivity of a weak electrolyte at infinite dilution cannot be determined experimentally.
07:28View SolutionAt a definite temperature, the molar conductivity of a weak electrolytic solution of concentration, C(M) is Lambda_(m) and the value of the molar conductivity at infinite dilution of the same electrolysis solution is Lambda_(m)^(@) . What will be the value of degree of dissociation of the electrolytr C(M) solution?
01:52View SolutionAssertion:Kohlrausch law helps to find the molar conductivity of weak electrolyte at infinite dilution. Reason:Molar conductivity of a weak electrolyte at infinite dilution cannot be determined experimentally.
07:44View Solution

